A ball of mass 'm' moving with a horizontal velocity 'v' strikes the bob of mass 'm' of a pendulum at rest. During this collision, the ball sticks with the bob of the pendulum. The height to which the combined mass raises is (g = acceleration due to gravity).
v24g
v28g
v2g
v22g
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Understand the scenario
We have a ball of mass 'm' moving with a horizontal velocity 'v' that strikes a pendulum bob of mass 'm' at rest. After the collision, the ball sticks to the bob, and we need to find the height 'h' to which the combined mass rises.
Step 2: Apply the conservation of momentum
Before the collision, the momentum of the system is given by the moving ball:
Initial momentum=m⋅v+m⋅0=mv
After the collision, the two masses stick together, so the total mass is m+m=2m. Let v′ be the velocity of the combined mass after the collision. By the conservation of momentum:
mv=(m+m)v′
mv=2mv′
Step 3: Solve for the velocity after the collision
Dividing both sides by 2m:
v′=v2
Step 4: Use energy conservation to find the height
After the collision, the kinetic energy of the combined mass is converted into potential energy at the maximum height 'h'. The kinetic energy just after the collision is:
KE=12(2m)(v′)2=m(v′)2=m(v2)2=m⋅v24
The potential energy at height 'h' is given by:
PE=(2m)gh=2mgh
Setting the kinetic energy equal to the potential energy:
m⋅v24=2mgh
Step 5: Simplify and solve for 'h'
Dividing both sides by m:
v24=2gh
Now, divide both sides by 2g:
h=v28g
Final Answer
The height to which the combined mass rises is:
h=v28g
We have a ball of mass 'm' moving with a horizontal velocity 'v' that strikes a pendulum bob of mass 'm' at rest. After the collision, the ball sticks to the bob, and we need to find the height 'h' to which the combined mass rises.
Before the collision, the momentum of the system is given by the moving ball:
Initial momentum=m⋅v+m⋅0=mv
mv=(m+m)v′
mv=2mv′
Dividing both sides by 2m:
v′=v2
After the collision, the kinetic energy of the combined mass is converted into potential energy at the maximum height 'h'. The kinetic energy just after the collision is:
KE=12(2m)(v′)2=m(v′)2=m(v2)2=m⋅v24
PE=(2m)gh=2mgh
m⋅v24=2mgh
Dividing both sides by m:
v24=2gh
h=v28g
The height to which the combined mass rises is:
h=v28g
Topper's Solved these Questions
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Match the coloumns
Explore 9 Videos
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEY ENGLISHChapter:CALORIMETRY AND HEAT TRANSFERExercise:Medical entrance s gallery
Explore 38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
Similar Questions
A bullet of mass m moving with velocity υ1 strikes a suspended wooden block of mass M as shown in the figure and sticks to it. If the block rises to a height y. the initial of the bullet is

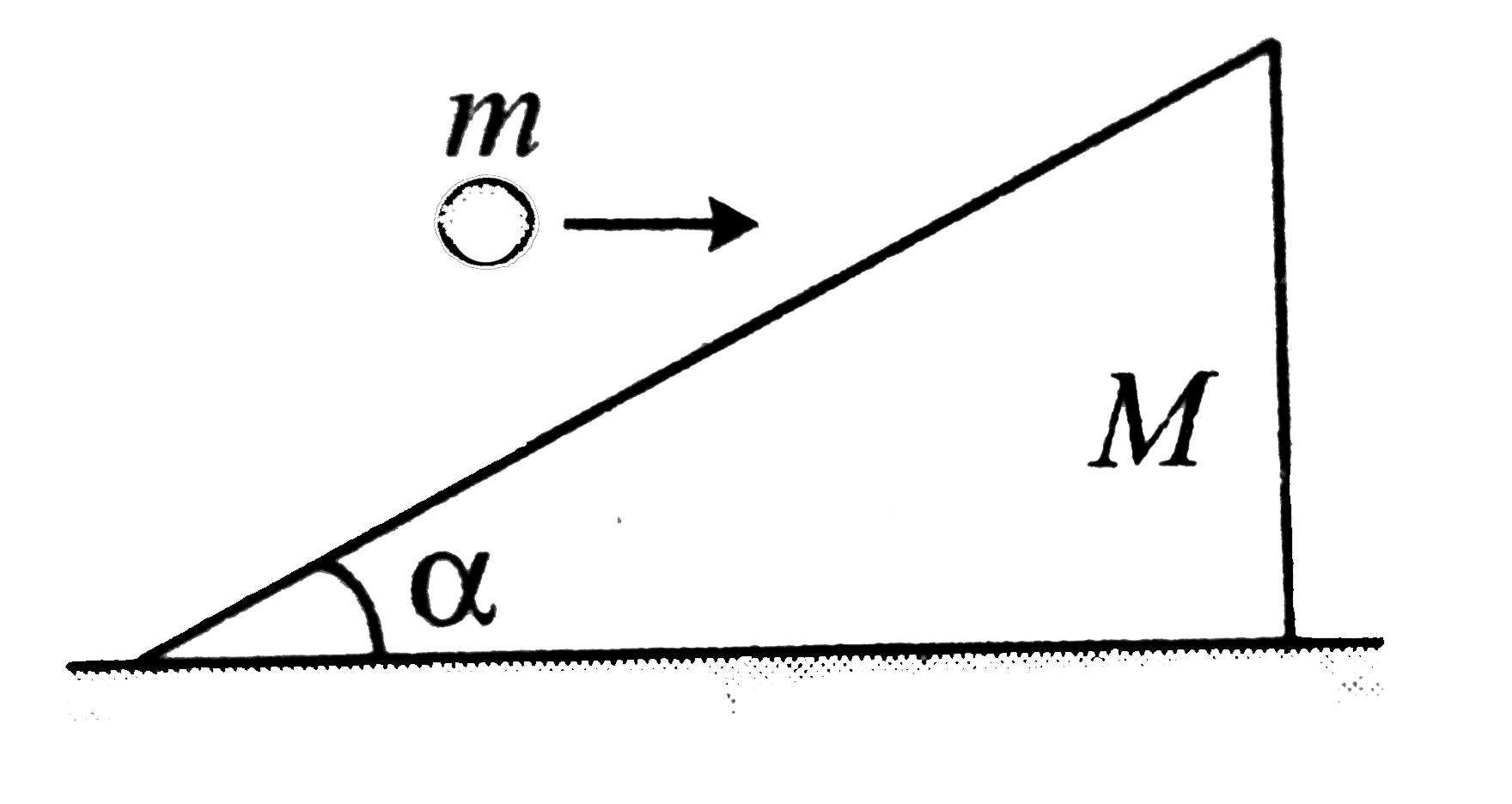
View SolutionA ball of mass m moving with constant horizontal velocity u strikes a stationary wedge of mass M on its inclined surface as shown in the figure. After collision, the ball starts moving up the inclined plane. The wedge is placed on frictionless horizontal surface.
a. Calculate the velocity of wedge immediately after collision.
b. Calculate the maximum height the ball can ascend on the wedge.
View SolutionA ball of mass m moving horizontally at a speed v collides with the bob of a simple pendulum at rest. The mass of the bob is also m. If the collision is perfectly elastic and both balls sticks, the height to which the two balls rise after the collision will be given by:
View SolutionA particle of mass 2m moving with velocity v strikes a stationary particle of mass 3m and sticks to it . The speed of the system will be
View SolutionA ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionA small ball of mass m moving with speed v collides elastically with a simple pendulum with bob of mass m at rest. The maximum height attained by the bob after collision is
View SolutionThe first ball of mass m moving with the velocity υ collides head on with the second ball of mass m at rest. If the coefficient of restitution is e, then the ratio of the velocities of the first and the second ball after the collision is
View SolutionThe period P of a simple pendulum is the time for one complete swing. How does P depend on the mass m of the bob, the length l of the string, and the acceleration due to gravity g?
View SolutionA mass m moving horizontal (along the x-axis) with velocity v collides and sticks to mass of 3m moving vertically upward (along the y-axis) with velocity 2v. The final velocity of the combination is
View SolutionA ball of mass '2m' moving with velocity uˆi collides with another ball of mass 'm' moving with velocity −2uˆi. After the collision, mass 2m moves with velocity u2(ˆi−ˆj). Then the change in kinetic energy of the system ( both balls ) is
View Solution