The potential energy of a particle of mass m is given by U=12kx2 for x<0 and U = 0 for x≥0. If total mechanical energy of the particle is E. Then its speed at x=√2Ek is
zero
√2Em
√Em
√E2m
The correct Answer is:B
To solve the problem, we need to find the speed of a particle at a specific position given its potential energy function and total mechanical energy.
1. Understand the Potential Energy Function:
The potential energy U is given by:
U=12kx2for x<0
and
U=0for x≥0
2. Total Mechanical Energy:
The total mechanical energy E of the particle is the sum of its kinetic energy K and potential energy U:
E=K+U
3. Determine Potential Energy at the Given Position:
We need to find the potential energy when x=√2Ek. Since x is positive, we use the second part of the potential energy function:
U=0for x≥0
4. Calculate Kinetic Energy:
Substitute U into the total mechanical energy equation:
E=K+0⟹K=E
5. Relate Kinetic Energy to Speed:
The kinetic energy K is given by:
K=12mv2
Setting this equal to E:
E=12mv2
6. Solve for Speed v:
Rearranging the equation to find v:
v2=2Em
Taking the square root gives:
v=√2Em
7. Conclusion:
Therefore, the speed of the particle at x=√2Ek is:
v=√2Em
The potential energy U is given by:
U=12kx2for x<0
and
U=0for x≥0
The total mechanical energy E of the particle is the sum of its kinetic energy K and potential energy U:
E=K+U
We need to find the potential energy when x=√2Ek. Since x is positive, we use the second part of the potential energy function:
U=0for x≥0
Substitute U into the total mechanical energy equation:
E=K+0⟹K=E
The kinetic energy K is given by:
K=12mv2
Setting this equal to E:
E=12mv2
Rearranging the equation to find v:
v2=2Em
Taking the square root gives:
v=√2Em
Therefore, the speed of the particle at x=√2Ek is:
v=√2Em
Topper's Solved these Questions
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - B More than One Option is Correct
26 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - C Comprehension Type Questions
18 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions
11 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
The potential energy of a particle of mass 1 kg moving along x-axis given by U(x)=[x22−x]J. If total mechanical speed (in m/s):-
View SolutionThe potential energy of a particle of mass 2 kg in SHM is (9x2)J. Here x is the displacement from mean position . If total mechanical energy of the particle is 36 J. The maximum speed of the particle is
View SolutionThe potential energy of a particle of mass m free to move along the x-axis is given by U=(1/2)kx2 for x<0 and U=0 for x≥0 (x denotes the x-coordinate of the particle and k is a positive constant). If the total mechanical energy of the particle is E, then its speed at x=−√2E/k is
View SolutionThe potential energy of a particle of mass m free to move along the x-axis is given by U=(1/2)kx2 for x<0 and U=0 for x≥0 (x denotes the x-coordinate of the particle and k is a positive constant). If the total mechanical energy of the particle is E, then its speed at x=−√2E/k is
View SolutionThe potential energy of a particle oscillating along x-axis is given as
U=20+(x−2)2
Here, U is in joules and x in meters. Total mechanical energy of the particle is 36J.
(a) State whether the motion of the particle is simple harmonic or not.
(b) Find the mean position.
(c) Find the maximum kinetic energy of the particle.
View SolutionPotential energy of a particle in SHM along x - axis is gives by
U=10+(x−2)2
Here, U is in joule and x in metre. Total mechanical energy of the particle is 26J. Mass of the particle is 2kg. Find
(a) angular frequency of SHM,
(b) potential energy and kinetic energy at mean position and extreme position,
(c ) amplitude of oscillation,
(d) x - coordinates between which particle oscillates.
View SolutionThe potential energy of a particle moving along y axis is given by U(y)= 3y4+12y2 ,(where U is in joule and y is in metre). If total mechanical energy is 15 joule, then limits of motion are
View SolutionThe figure shows the variation of potential energy of a particle as a function pf x, the x-coordination of the region. It has been assumed that potential energy depends only on x. For all other values x,U is zero. i.e. forx<−10 and x>15,U=0.

If total mechanical energy of the particle is 25J, then it can found in the region
View SolutionThe figure shows the variation of potential energy of a particle as a funcation of x, the x-coordinate of the region. It has been assumed that potential energy depends only on x. For all other values x,U is zero. i.e. forx<−10 and x>15,U=0.
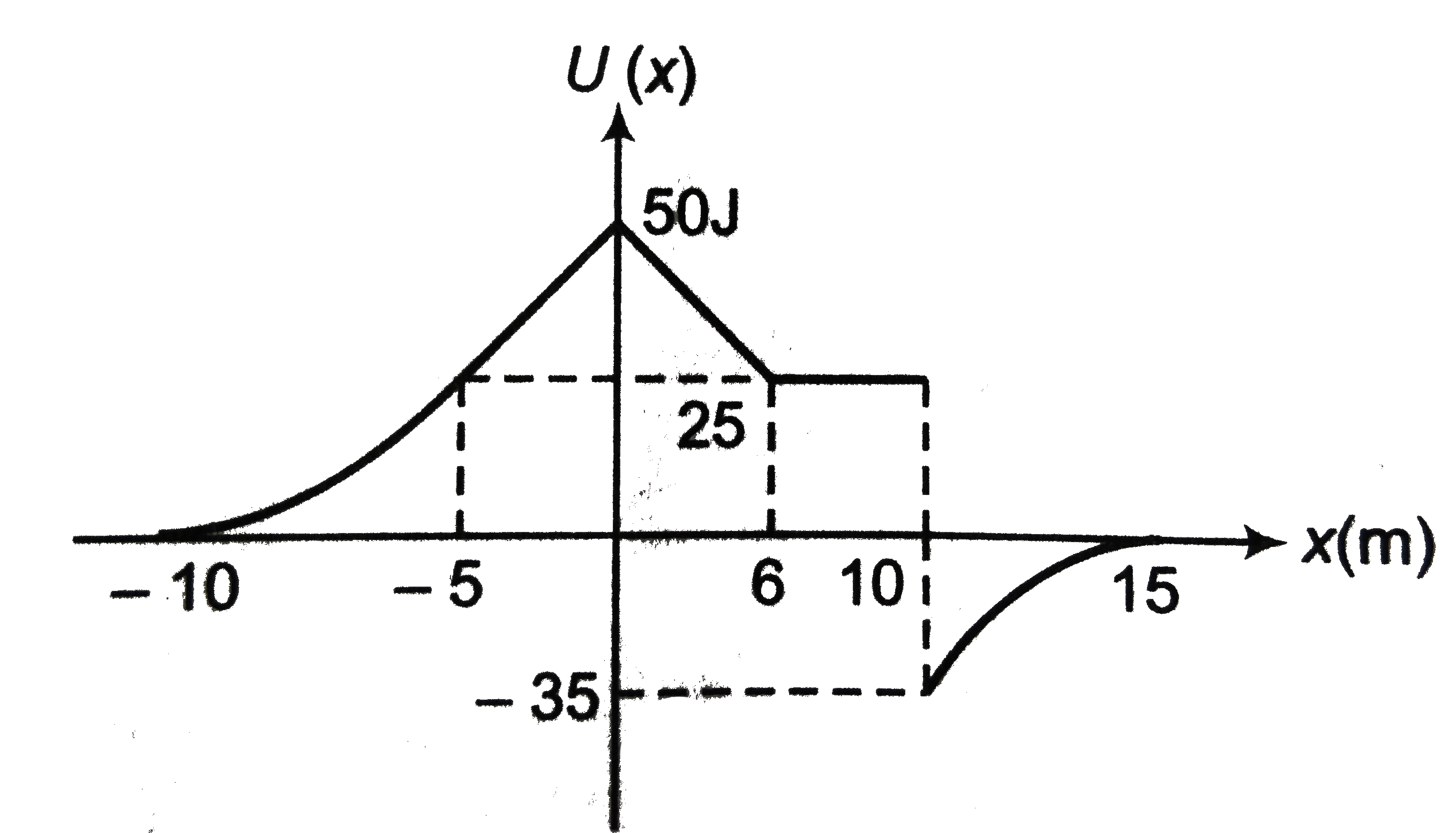
If total mechanical energy of the particle is −40J, then it can be found in region.
View Solutionathe potential energy of a particle of mass 2kg moving along the x-axis is given by U(x)=4x2−2x3( where U is in joules and x is in meters). The kinetic energy of the particle is maximum at
View Solution