Choose the correct option:
A wire of length l and mass m is first bent in a circle, then in a square and then in an equilateral triangle. The moment of inertia in these three cases about an axis perpendicular to their planes and passing through their centre of mass are I1,I2 and I3 respectively. Then maximum of them is
(a) I1
(b) I2
(c) I3
(d) Data insufficient
The correct Answer is:A
To solve the problem, we need to calculate the moment of inertia for a wire bent into three different shapes: a circle, a square, and an equilateral triangle. We will denote the moments of inertia for these shapes as I1, I2, and I3 respectively.
Step 1: Calculate the moment of inertia for the circle
1. Circumference of the circle: The circumference C is equal to the length of the wire L:
C=2πr⟹L=2πr⟹r=L2π
2. Moment of inertia for a circular wire: The moment of inertia I1 about an axis perpendicular to the plane of the circle and through its center of mass is given by:
I1=mr2=m(L2π)2=mL24π2
Step 2: Calculate the moment of inertia for the square
1. Perimeter of the square: The perimeter P is equal to the length of the wire L:
P=4a⟹L=4a⟹a=L4
where a is the length of one side of the square.
2. Moment of inertia for a square wire: The moment of inertia I2 about an axis perpendicular to the plane of the square and through its center of mass is given by:
I2=16m(2a2)=16m(2⋅(L4)2)=mL296
Step 3: Calculate the moment of inertia for the equilateral triangle
1. Perimeter of the equilateral triangle: The perimeter P is equal to the length of the wire L:
P=3b⟹L=3b⟹b=L3
where b is the length of one side of the triangle.
2. Moment of inertia for an equilateral triangle wire: The moment of inertia I3 about an axis perpendicular to the plane of the triangle and through its center of mass is given by:
I3=16mb2=16m(L3)2=mL254
Step 4: Compare the moments of inertia
Now we have the expressions for the moments of inertia:
- I1=mL24π2
- I2=mL296
- I3=mL254
To determine which is the maximum, we can compare the denominators:
- 4π2≈39.478
- 96
- 54
Since 4π2<54<96, we find that:
I1>I3>I2
Conclusion
Thus, the maximum moment of inertia is:
Maximum of (I1,I2,I3)=I1
The correct option is A, I1.
C=2πr⟹L=2πr⟹r=L2π
2. Moment of inertia for a circular wire: The moment of inertia I1 about an axis perpendicular to the plane of the circle and through its center of mass is given by:
I1=mr2=m(L2π)2=mL24π2
P=4a⟹L=4a⟹a=L4
where a is the length of one side of the square.
I2=16m(2a2)=16m(2⋅(L4)2)=mL296
P=3b⟹L=3b⟹b=L3
where b is the length of one side of the triangle.
I3=16mb2=16m(L3)2=mL254
- I1=mL24π2
- I2=mL296
- I3=mL254
- 4π2≈39.478
- 96
- 54
I1>I3>I2
Maximum of (I1,I2,I3)=I1
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - More than one option is correct36videos
ROTATIONAL MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Comprehension Type Questions40videos
ROTATIONAL MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions17videos
ROTATIONAL MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions2videos
ROTATIONAL MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct3videos
SEMICONDUCTORS AND ELECTRONIC DEVICES
Similar Questions
A rod of mass 3 kg and of length 3m is bent in the form of an equilateral triangle. The moment of inertia of the triangle about a vertical axis perpendicular to plane and passing through centre of mass is
 View Solution
View SolutionA light rod of length l has two masses m1 and m2 attached to its two ends. The moment of inertia of the system about an axis perpendicular to the rod and passing through the centre of mass is.
View SolutionConsider a uniform square plate of of side and mass m. The moment of inertia of this plate about an axis perpendicular to its plane and passing through one of its corners is -
View SolutionCalculate the moment of inertia of a rod of mass 2 kg and length 5 m about an axis perpendicular to it and passing through one of its ends.
View SolutionCalculate the moment of inertia of a rod of mass M, and length l about an axis perpendicular to it passing through one of its ends.
View SolutionThe moment of inertia of a straight thin rod of mass M and length l about an axis perpendicular to its length and passing through its one end, is
View SolutionTwo point masses m and 4m are connected by a light rigid rod of length l at the opposite ends. Moment of inertia of the system about an axis perpendicular to the length and passing through the centre of mass is
View SolutionA rod of mass Mkg and length Lm is bent in the from of an equilateral tringle as shown in fig. The moment of inertia of the triangle about a vertical axis perpendicular to the plane of the trinangle and passing through the centre (in units of kgm2) is.
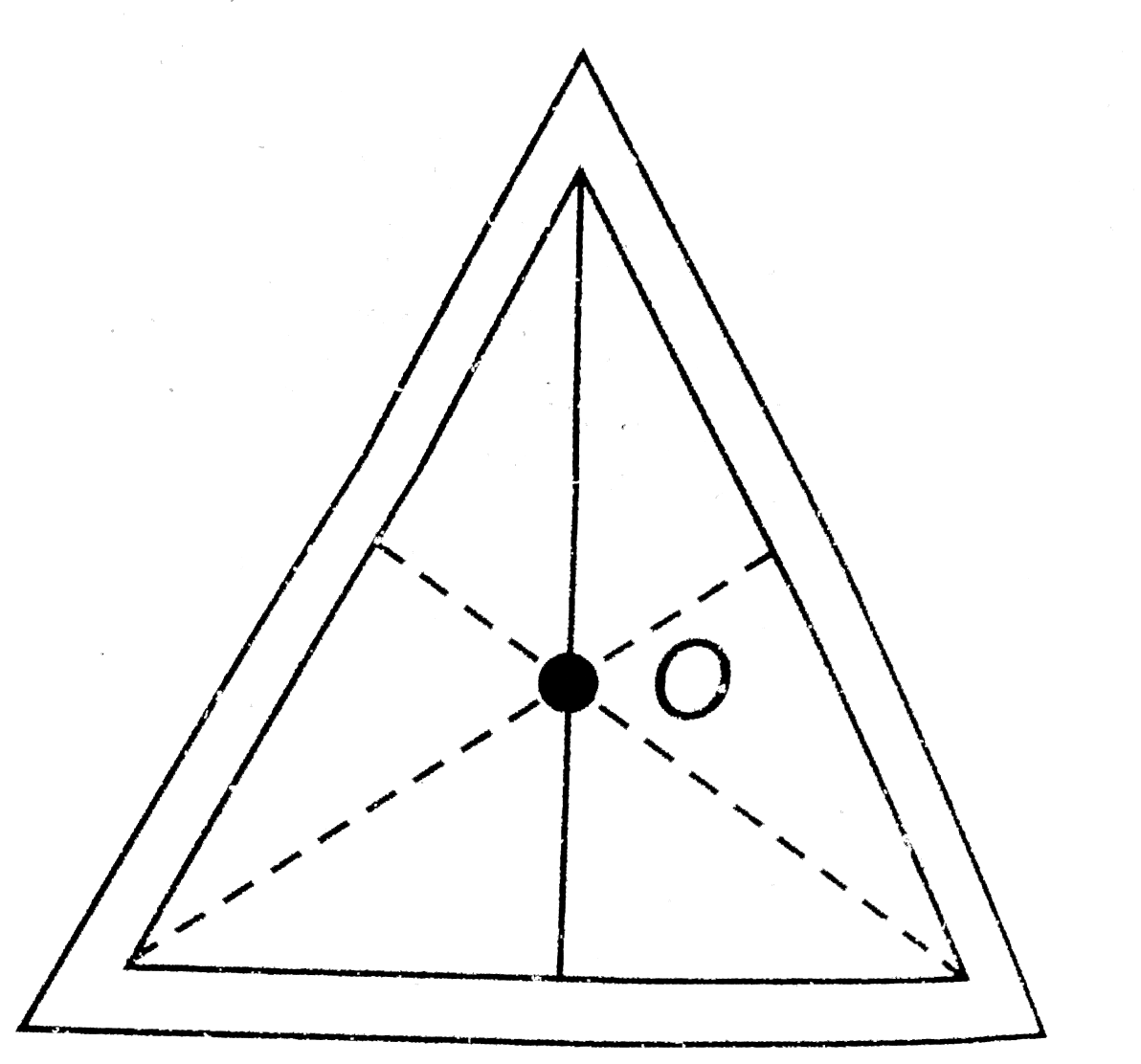 .View Solution
.View SolutionThe moment of inertia of a uniform semicircularwire of mass M and radius r about a line perpendicular to the plane of the wire through the centre is :
View SolutionA thin rod of length 4l, mass 4 m is bent at the point as shown in the figure. What is the moment of inertia of the rod about the axis passing through O and perpendicular to the plane of the paper?
 View Solution
View Solution

