A uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B, perpendicular to the rod in the horizontal direction. Speed of particlem P at a distance l6 from the centre towards A of the rod after time t=πml12J is.
2JM
J√2M
JM
√2JM
The correct Answer is:D
To solve the problem, we need to analyze the motion of the uniform rod after an impulse is applied. The impulse causes both translational and rotational motion of the rod. Let's break down the steps to find the speed of the particle P after a given time.
1. Understanding the System:
- We have a uniform rod AB of mass m and length l resting on a smooth horizontal surface.
- An impulse J is applied at the end B perpendicular to the rod.
2. Finding the Velocity of the Center of Mass:
- The impulse J causes a change in momentum. Since the rod is initially at rest, the change in momentum is equal to the impulse:
\(
J = m \cdot v_{cm}
\)
- Therefore, the velocity of the center of mass (vcm) of the rod after the impulse is:
\(
v_{cm} = \frac{J}{m}
\)
3. Calculating the Angular Velocity:
- The impulse also causes the rod to rotate about its center of mass. The angular impulse is given by:
\(
\text{Angular Impulse} = J \cdot \frac{l}{2}
\)
- The moment of inertia I of the rod about its center is:
\(
I = \frac{ml^2}{12}
\)
- The change in angular momentum is equal to the angular impulse:
\(
J \cdot \frac{l}{2} = I \cdot \omega
\)
- Substituting for I:
\(
J \cdot \frac{l}{2} = \frac{ml^2}{12} \cdot \omega
\)
- Solving for angular velocity (ω):
\(
\omega = \frac{6J}{ml}
\)
4. Finding the Angle Rotated After Time t:
- The time given is:
\(
t = \frac{\pi ml}{12J}
\)
- The angle rotated (θ) in time t is:
\(
θ = \omega \cdot t = \left(\frac{6J}{ml}\right) \cdot \left(\frac{\pi ml}{12J}\right) = \frac{\pi}{2}
\)
- This means the rod has rotated 90 degrees and is now horizontal.
5. Finding the Velocity of Point P:
- Point P is located at a distance of l6 from the center of the rod towards A.
- The linear velocity of point P due to the translational motion of the center of mass is:
\(
v_{P, cm} = v_{cm} = \frac{J}{m}
\)
- The tangential velocity of point P due to rotation is:
\(
v_{P, rot} = \omega \cdot \left(\frac{l}{6}\right) = \left(\frac{6J}{ml}\right) \cdot \left(\frac{l}{6}\right) = \frac{J}{m}
\)
- Since the rod is horizontal, the velocities are perpendicular. Therefore, the resultant velocity of point P is:
\(
v_P = \sqrt{(v_{P, cm})^2 + (v_{P, rot})^2} = \sqrt{\left(\frac{J}{m}\right)^2 + \left(\frac{J}{m}\right)^2} = \sqrt{2 \left(\frac{J}{m}\right)^2} = \frac{J}{m} \sqrt{2}
\)
6. Final Result:
- The speed of particle P after time t is:
\(
v_P = \frac{J \sqrt{2}}{m}
\)
- We have a uniform rod AB of mass m and length l resting on a smooth horizontal surface.
- An impulse J is applied at the end B perpendicular to the rod.
- The impulse J causes a change in momentum. Since the rod is initially at rest, the change in momentum is equal to the impulse:
\(
J = m \cdot v_{cm}
\)
- Therefore, the velocity of the center of mass (vcm) of the rod after the impulse is:
\(
v_{cm} = \frac{J}{m}
\)
- The impulse also causes the rod to rotate about its center of mass. The angular impulse is given by:
\(
\text{Angular Impulse} = J \cdot \frac{l}{2}
\)
- The moment of inertia I of the rod about its center is:
\(
I = \frac{ml^2}{12}
\)
- The change in angular momentum is equal to the angular impulse:
\(
J \cdot \frac{l}{2} = I \cdot \omega
\)
- Substituting for I:
\(
J \cdot \frac{l}{2} = \frac{ml^2}{12} \cdot \omega
\)
- Solving for angular velocity (ω):
\(
\omega = \frac{6J}{ml}
\)
- The time given is:
\(
t = \frac{\pi ml}{12J}
\)
- The angle rotated (θ) in time t is:
\(
θ = \omega \cdot t = \left(\frac{6J}{ml}\right) \cdot \left(\frac{\pi ml}{12J}\right) = \frac{\pi}{2}
\)
- This means the rod has rotated 90 degrees and is now horizontal.
- Point P is located at a distance of l6 from the center of the rod towards A.
- The linear velocity of point P due to the translational motion of the center of mass is:
\(
v_{P, cm} = v_{cm} = \frac{J}{m}
\)
- The tangential velocity of point P due to rotation is:
\(
v_{P, rot} = \omega \cdot \left(\frac{l}{6}\right) = \left(\frac{6J}{ml}\right) \cdot \left(\frac{l}{6}\right) = \frac{J}{m}
\)
- Since the rod is horizontal, the velocities are perpendicular. Therefore, the resultant velocity of point P is:
\(
v_P = \sqrt{(v_{P, cm})^2 + (v_{P, rot})^2} = \sqrt{\left(\frac{J}{m}\right)^2 + \left(\frac{J}{m}\right)^2} = \sqrt{2 \left(\frac{J}{m}\right)^2} = \frac{J}{m} \sqrt{2}
\)
- The speed of particle P after time t is:
\(
v_P = \frac{J \sqrt{2}}{m}
\)
Topper's Solved these Questions
- ROTATIONAL MOTIONBook:DC PANDEY ENGLISHChapter:ROTATIONAL MOTIONExercise:More than one option is correct
Explore 36 Videos
- ROTATIONAL MOTIONBook:DC PANDEY ENGLISHChapter:ROTATIONAL MOTIONExercise:Comprehension Type Questions
Explore 40 Videos
- ROTATIONAL MOTIONBook:DC PANDEY ENGLISHChapter:ROTATIONAL MOTIONExercise:Integer Type Questions
Explore 17 Videos
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Subjective Questions
Explore 2 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBook:DC PANDEY ENGLISHChapter:SEMICONDUCTORS AND ELECTRONIC DEVICESExercise:More than One Option is Correct
Explore 3 Videos
Similar Questions
A uniform rod AB of mass m and length l at rest on a smooth horizontal surface . An impulse P is applied to the end B. The time taken by the rod to turn through a right angle is :

View SolutionA uniform rod AB of mass m and length l at rest on a smooth horizontal surface . An impulse P is applied to the end B. The time taken by the rod to turn through a right angle is :

View SolutionA uniform rod of mass m, length l rests on a smooth horizontal surface. Rod is given a sharp horizontal impulse p perpendicular to the rod at a distance l/4 from the centre. The angular velocity of the rod will be
View SolutionA uniform rod of mass m and length l is lying on a smooth table. An impulse J acts on the rod momentarily as shown in figure at point R. Just after that:


View SolutionA uniform rod of mass m and length L is at rest on a smooth horizontal surface. A ball of mass m, moving with velocity v0, hits the rod perpendicularly at its one end and sticks to it. The angular velocity of rod after collision is

View SolutionTwo particles connected by a rigid light rod AB, lying on a smooth horizontal table. An impulse J is applied at A in the plane of thetable and perpendicular at AB. Then the velocity of particle at A is
View Solution
A uniform rod of mass 3 m and length l is lying on a smooth horizontal surface. Two particle of mass m and 2 m moving with speed 2v0 and v0 in the opposite directly hit the rod perpendicularly at a distance l3 and l6 from the midpoint O of the rod ad stick to it after collision. if the loss in kinetic energy of the system due to collision is n5mv20 find the value of n.
View SolutionA uniform rod of mass M and length L lies flat on a frictionless horizantal surface. Two forces F and 2F are applied along the length of the rod as shown. The tension in the rod at point P is

View Solution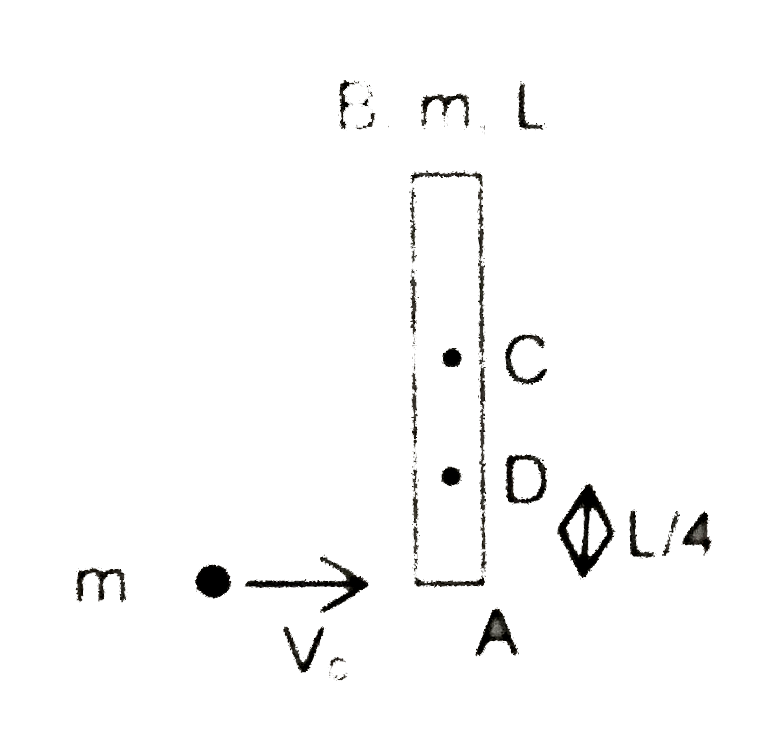
A thin, uniform rod of mass M and length L is at rest on a smooth horizontal surface. A particle of same mass M collides with the rod at one end perpendicular to its length with velocity V∘ and sticks to it. C is the middle point of the rod and D is a point at a distance of L/4 from end A. the time taken by the rod turn through 90∘ after collisions.
View SolutionA uniform rod of mass m and length l rests on a smooth horizontal surface. One of the ends of the rod is struck in a horizontal direction at right angles to the rod. As a result the rod obtains velocity v0. Find the force with which one-half of the rod will act ont he other in the process of motion.
View Solution