A person can hear frequencies only upto 10kHz. A steel piano pipe wire 50cm long of mass 5g is streched with a tension of 400N. The number of the hightest overtone of the sound produced by this plano wire that the person can hear is
48
50
49
51
The correct Answer is:C
To solve the problem step by step, we will follow the principles of wave motion and the properties of vibrating strings.
Step 1: Understand the given data
- Maximum frequency a person can hear, fmax=10kHz=10×103Hz
- Length of the wire, L=50cm=0.5m
- Mass of the wire, m=5g=5×10−3kg
- Tension in the wire, T=400N
Step 2: Calculate the linear mass density (μ)
The linear mass density μ is given by:
μ=mL
Substituting the values:
μ=5×10−3kg0.5m=0.01kg/m
Step 3: Calculate the wave speed (V)
The wave speed V in the wire can be calculated using the formula:
V=√Tμ
Substituting the values:
V=√400N0.01kg/m=√40000=200m/s
Step 4: Calculate the fundamental frequency (f1)
The fundamental frequency f1 is given by the formula:
f1=V2L
Substituting the values:
f1=200m/s2×0.5m=2001=200Hz
Step 5: Calculate the overtone frequencies
The frequency of the n-th overtone is given by:
fn=n⋅f1
Thus, the maximum frequency that can be heard is:
fn≤fmax
Substituting the values:
n⋅200Hz≤10×103Hz
n≤10×103200=50
Step 6: Determine the highest overtone
The highest overtone is given by:
Highest overtone=n−1
Substituting the value of n:
Highest overtone=50−1=49
Final Answer
The number of the highest overtone of the sound produced by this piano wire that the person can hear is 49.
---
- Maximum frequency a person can hear, fmax=10kHz=10×103Hz
- Length of the wire, L=50cm=0.5m
- Mass of the wire, m=5g=5×10−3kg
- Tension in the wire, T=400N
The linear mass density μ is given by:
μ=mL
Substituting the values:
μ=5×10−3kg0.5m=0.01kg/m
The wave speed V in the wire can be calculated using the formula:
V=√Tμ
Substituting the values:
V=√400N0.01kg/m=√40000=200m/s
The fundamental frequency f1 is given by the formula:
f1=V2L
Substituting the values:
f1=200m/s2×0.5m=2001=200Hz
The frequency of the n-th overtone is given by:
fn=n⋅f1
Thus, the maximum frequency that can be heard is:
fn≤fmax
Substituting the values:
n⋅200Hz≤10×103Hz
n≤10×103200=50
The highest overtone is given by:
Highest overtone=n−1
Substituting the value of n:
Highest overtone=50−1=49
The number of the highest overtone of the sound produced by this piano wire that the person can hear is 49.
Topper's Solved these Questions
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - ONLY ONE OPTION IS CORRECT
62 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - More Than One Option is Correct
23 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Subjective Questions
2 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
Similar Questions
An organ pipe is closed at one end has fundamental frequency of 1500 Hz. The maximum number of overtones generated by this pipe which a normal person can hear is
View SolutionA steel wire 100 cm long has a mass of 10 gm. If the wire is under a tension of 400 N, what is the speed of transverse waves in the wire?
View Solution
Knowledge Check
A 10 m long steel wire has mass 5 g. If the wire is under a tension of 80 N, the speed of transverse waves on the wire is
A100ms−1B200ms−1C400ms−1D500ms−1
A steel wire 75cm long has mass of 5.5 10−3kg. If the wire is under a tension of 65N, what is the speed of transverse waves on the wire?
View SolutionA steel wire 0.72 m long has a mass of 5.0×10−3 kg . If the wire is under a tension of 60 N, what is the speed of transverse waves on the wire ?
View SolutionWhat is the second lowest frequency for standing waves on a wire that is 10.0 m long has a mass of 100 g and is stretched under a tension of 25 N which is fixed at both ends ?
View SolutionA simple harmonic oscillator at the point x=0 genrates a wave on a rope. The oscillator operates at a frequency of 40.0 Hz and with an amplitude of 3.00 cm. the rope has a linear mass density of 50.0g/m and is strectches with a tension of 5.00 N. (a) determine the speed of the wave. (b) find the wavelength. (c ) write the wave function y(x,t) for the wave, Assume that the oscillator has its maximum upward displacement at time t=0. (d) find the maximum transverse acceleration at time t=0. (d) find the maximum transverse of points on the rope. (e) in the discussion of transverse waves in this chapter, the force of gravity was ignored. is that a reasonable assumption for this wave? explain.
View SolutionThe ciliary muscles of eye control the curvature of the lens in the eye and hence can alter the effective focal length of the system. When the muscles are fully relaxed, the focal length is maximum. When the muscles are strained, the curvature of lens increases. That means radius of curvature decreases and focal length decreases. For a clear vision, the image must be on the retina. The image distance is therefore fixed for clear vision and it equals the distance of retina from eye lens. It is about 2.5cm for a grown up person.
A perosn can theoretically have clear vision of an object situated at any large distance from the eye. The smallest distance at which a person can clearly see is related to minimum possible focal length. The ciliary muscles are most strained in this position. For an average grown up person, minimum distance of the object should be around 25cm.
A person suffering from eye defects uses spectacles (eye glass). The function of lens of spectacles is to form the image of the objects within the range in which the person can see clearly. The image o the spectacle lens becomes object for the eye lens and whose image is formed on the retina.
The number of spectacle lens used for th eremedy of eye defect is decided by the power fo the lens required and the number of spectacle lens is equal to the numerical value of the power of lens with sign. For example, if power of the lens required is +3D (converging lens of focal length 100/3cm ), then number of lens will be +3.
For all the calculations required, you can use the lens formula and lensmaker's formula. Assume that the eye lens is equiconvex lens. Neglect the distance between the eye lens and the spectacle lens.
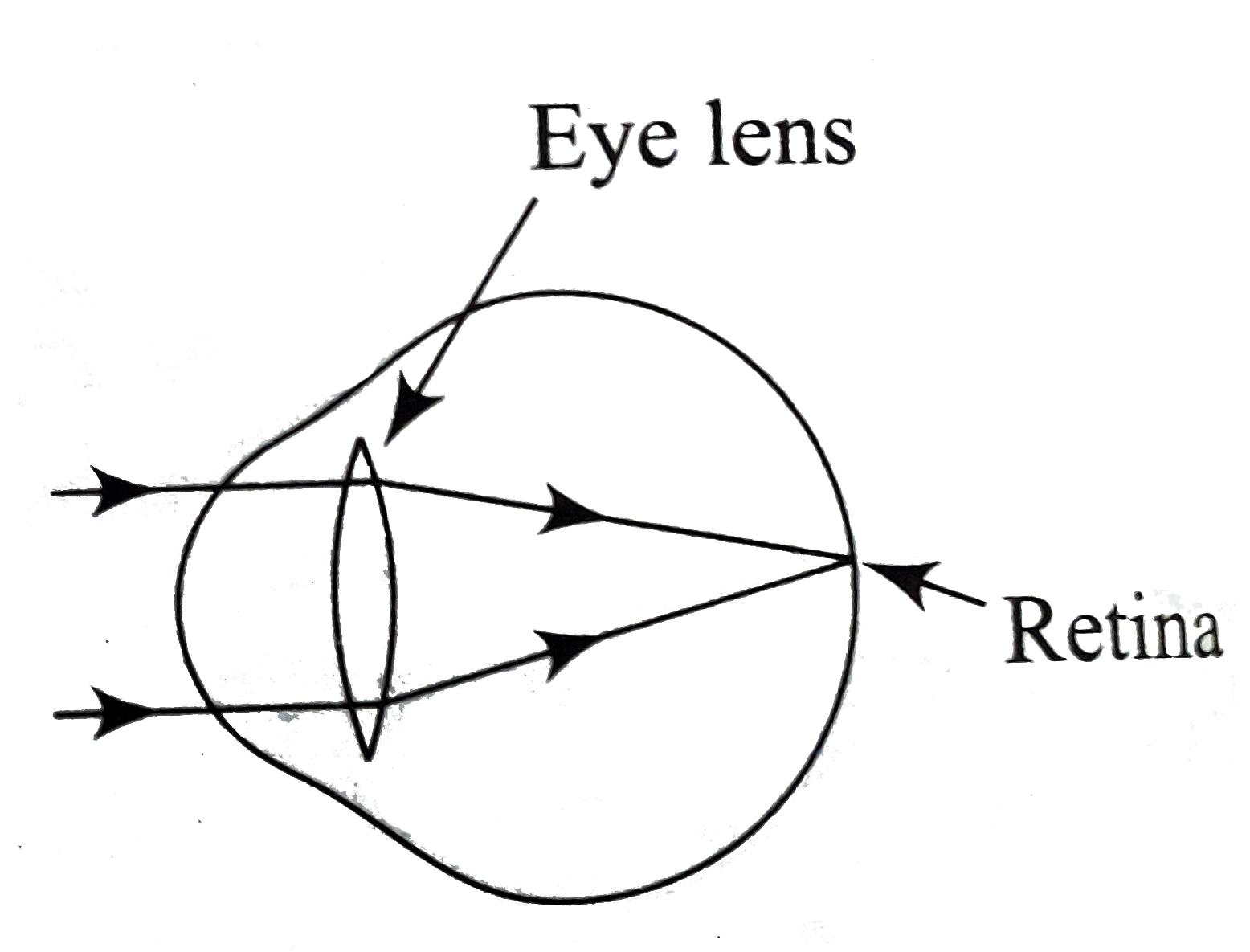
Q. A near sighted man can clearly see ojects only upto a distance of 100cm and not beyond this. The number of the spectacle lenses necessary for the remedy of this defect will be
View SolutionA person can hear sound waves in the frequency range 20 Hz to 20 kHz. Find the minimum and the maximum wavelengths of sound that is audible to the person. The speed of sound is 360ms−1.
View SolutionA 30.0-cm-long wire having a mass of 10.0 g is fixed at the two ends and is vibrated in its fundamental mode.A 50.0-cm-long closed organ pipe, placed with its open end near the wire, is set up into resonance in its fundamental mode by the vibrating wire. Find the tension in the wire. Speed of sound in air =340ms−1.
View Solution
