A Uniform rope having mass m hags vertically from a rigid support. A transverse wave pulse is produced at the lower end. The speed v of wave pulse varies with height h from the lower end as

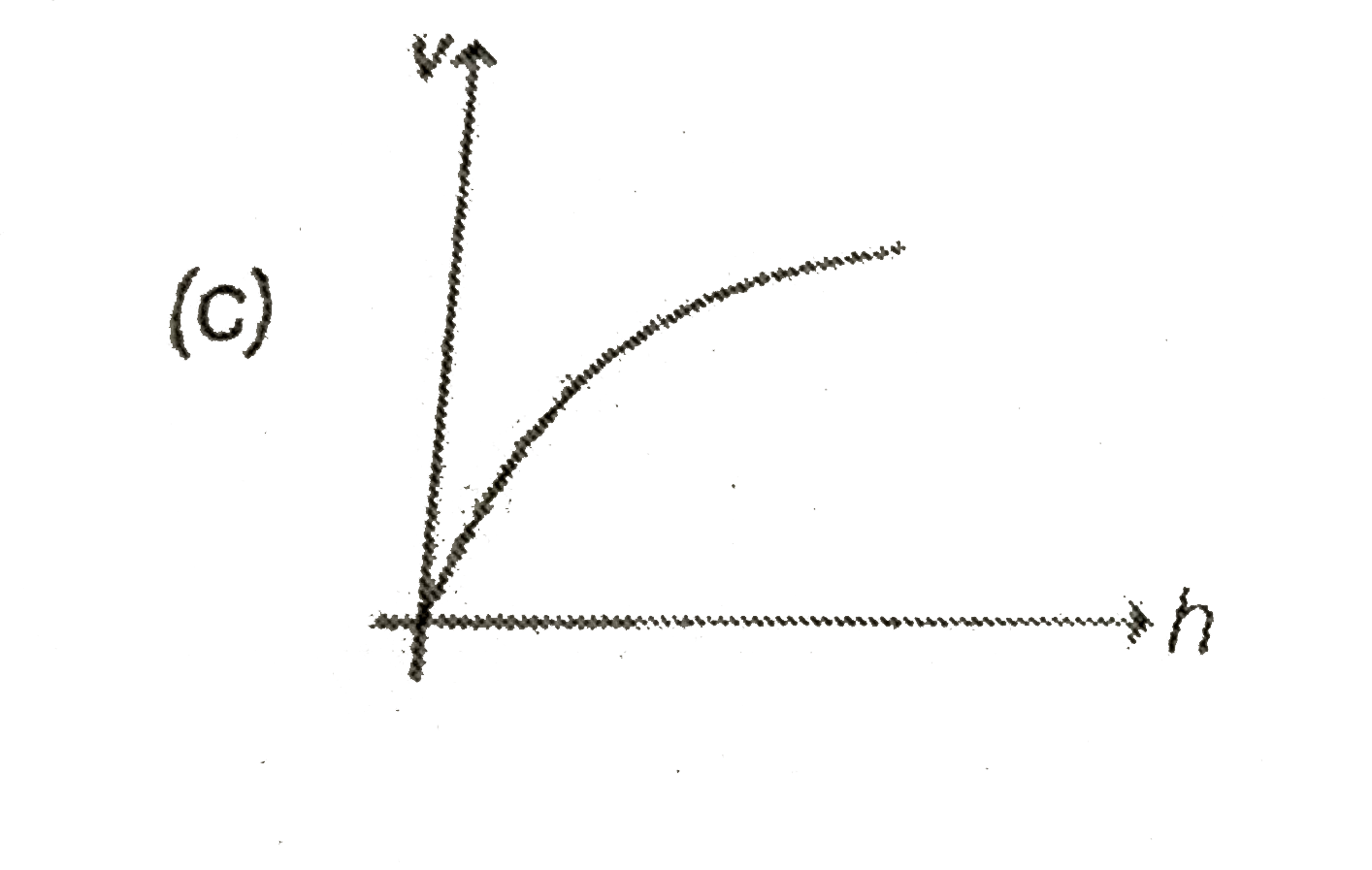

The correct Answer is:C
To solve the problem, we need to determine how the speed v of a transverse wave pulse in a hanging rope varies with the height h from the lower end of the rope. Here’s a step-by-step solution:
Step 1: Understand the relationship between wave speed, tension, and linear mass density
The speed v of a wave in a rope is given by the formula:
v=√Tμ
where T is the tension in the rope and μ is the linear mass density of the rope.
Step 2: Determine the tension in the rope at a height h
Consider a small segment of the rope of length x from the bottom. The mass of this segment is:
mx=μx
The tension T at height h is due to the weight of the rope below that point. Therefore, the tension can be expressed as:
T=mxg=μxg
where g is the acceleration due to gravity.
Step 3: Substitute the tension into the wave speed formula
Substituting the expression for tension into the wave speed formula, we get:
v=√μxgμ=√xg
Step 4: Relate height h to the length x
Since the rope is hanging vertically, the height h from the bottom of the rope is related to the length x of the rope below the point of interest. Thus, we can express x in terms of h:
x=L−h
where L is the total length of the rope.
Step 5: Substitute x into the wave speed equation
Now substituting x=L−h into the wave speed equation gives:
v=√(L−h)g
Step 6: Analyze the relationship between v and h
From the equation v=√(L−h)g, we can see that as h increases (moving up the rope), v decreases. The relationship is a square root function, which indicates that the speed v varies with height h in a parabolic manner.
Conclusion
Thus, the speed v of the wave pulse varies with height h from the lower end of the rope as:
v∝√(L−h)
This indicates that the speed decreases as we move up the rope.
The speed v of a wave in a rope is given by the formula:
v=√Tμ
where T is the tension in the rope and μ is the linear mass density of the rope.
Consider a small segment of the rope of length x from the bottom. The mass of this segment is:
mx=μx
The tension T at height h is due to the weight of the rope below that point. Therefore, the tension can be expressed as:
T=mxg=μxg
where g is the acceleration due to gravity.
Substituting the expression for tension into the wave speed formula, we get:
v=√μxgμ=√xg
Since the rope is hanging vertically, the height h from the bottom of the rope is related to the length x of the rope below the point of interest. Thus, we can express x in terms of h:
x=L−h
where L is the total length of the rope.
Now substituting x=L−h into the wave speed equation gives:
v=√(L−h)g
From the equation v=√(L−h)g, we can see that as h increases (moving up the rope), v decreases. The relationship is a square root function, which indicates that the speed v varies with height h in a parabolic manner.
Thus, the speed v of the wave pulse varies with height h from the lower end of the rope as:
v∝√(L−h)
This indicates that the speed decreases as we move up the rope.
Topper's Solved these Questions
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - ONLY ONE OPTION IS CORRECT
62 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - More Than One Option is Correct
23 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Subjective Questions
2 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
Similar Questions
A uniform rope of length 12 m and mass 4 kg is hung vertically from a rigid support transverse wave pulse is produced at lower end. The speed of the wave pulse al x = 2.5 m from lower and will be kg=10 m/s)
View SolutionA heavy rope is suspended from a rigid support A wave pulse is set up at the lower end, then
View SolutionA heavy rope is suspended from a rigid support A wave pulse is set up at the lower end, then
View SolutionA wire of length L, is hanging vertically from a rigid support. If a transverse wave pulse is generated at the free end of wire then which of the following statement is wrong
View SolutionA string is hanging from a rigid support. A transverse
pulse is excited at its free end. The speed at which the
pulse travels a distance x is proportional to
View SolutionA uniform rope of elngth l and mass m hangs vertically from a rigid support. A block of mass m is attached to the free end of the rope. A transverse pulse of wavelength λ is produced at the lower end of the rope. The wavelength of the pulse, when it reaches the top of the rope. is aλ. Find a2

View SolutionA string is hanging from a rigid support. A transverse wave pulse is set up at the bottom. The velocity v of thr pulse related to the distance covered by it is given as

View SolutionA uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 10 cm and mass 4 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope, what is the wavelength of the pulse when it reaches the top of the rope?
View Solution
