A parallel plate capacitor of area 100cm2 and plate separation 8.85 mm is charged to a potential difference of 100 V when air is used between the plates. The capacitor is now isolated and air is replaced by glass (εr−5), then:
The original capacity will be 10 pF
The new capacity will be 50 pF
The new potential difference is 200 V
The new energy stored is 50 μJ
The correct Answer is:A, B
To solve the problem step by step, we will calculate the original capacitance, the new capacitance after replacing air with glass, the new potential difference, and the energy stored in the capacitor.
Step 1: Calculate the Original Capacitance (C₀)
The formula for the capacitance of a parallel plate capacitor is given by:
C=ϵAd
Where:
- C = capacitance
- ϵ = permittivity of the dielectric material (for air, ϵ=ϵ0)
- A = area of the plates
- d = separation between the plates
Given:
- Area A=100cm2=100×10−4m2=0.01m2
- Plate separation d=8.85mm=8.85×10−3m
- Permittivity of free space ϵ0=8.85×10−12F/m
Substituting the values:
C0=(8.85×10−12F/m)(0.01m2)8.85×10−3m=8.85×10−148.85×10−3=10×10−12F=10pF
Step 2: Calculate the New Capacitance (C)
When the air is replaced by glass with a dielectric constant ϵr=5, the new capacitance C is given by:
C=ϵrC0
Substituting the values:
C=5×10pF=50pF
Step 3: Calculate the Charge (Q)
The charge on the capacitor when it was charged to 100 V is given by:
Q=C0V0
Where V0=100V:
Q=10pF×100V=1000pC=100pC
Step 4: Calculate the New Potential Difference (V)
Since the capacitor is isolated, the charge remains constant. The new potential difference V can be calculated using:
V=QC
Substituting the values:
V=100pC50pF=100×10−12C50×10−12F=2V
Step 5: Calculate the Energy Stored (U)
The energy stored in the capacitor is given by:
U=12CV2
Substituting the values:
U=12×50×10−12F×(2V)2=12×50×10−12×4=100×10−12J=10−10J
Converting to microjoules:
U=10−10J=0.1μJ=100nJ
Summary of Results
1. Original capacitance C0=10pF
2. New capacitance C=50pF
3. New potential difference V=2V
4. Energy stored U=0.1μJ
The formula for the capacitance of a parallel plate capacitor is given by:
- C = capacitance
- ϵ = permittivity of the dielectric material (for air, ϵ=ϵ0)
- A = area of the plates
- d = separation between the plates
- Area A=100cm2=100×10−4m2=0.01m2
- Plate separation d=8.85mm=8.85×10−3m
- Permittivity of free space ϵ0=8.85×10−12F/m
When the air is replaced by glass with a dielectric constant ϵr=5, the new capacitance C is given by:
The charge on the capacitor when it was charged to 100 V is given by:
Since the capacitor is isolated, the charge remains constant. The new potential difference V can be calculated using:
The energy stored in the capacitor is given by:
1. Original capacitance C0=10pF
2. New capacitance C=50pF
3. New potential difference V=2V
4. Energy stored U=0.1μJ
Similar Questions
A parallel-plate condenser of area 100 cm2, plate separation 5 mm is charged to a p.d. of 100 V when air is used between the plates. If now air is replaced by glass (εr=6) calculate (a) the new capacity, (b) the new p.d. and (c) the loss of energy. Account for the loss of energy.
View SolutionA 5.80μF parallel-plate air capacitor has a plate separation of 5.00mm and is charged to a potential difference of 400V. Calculate the energy density in the region between the plates, in Jm3
View SolutionA parallel plate capacitor having a plate separation of 2mm is charged by connecting it to a 300v supply. The energy density is
View SolutionA parallel - plate capacitor of plate area A and plate separation d is charged to a potential difference V and then the battery is disconnected . A slab of dielectric constant K is then inserted between the plate of the capacitor so as to fill the space between the plate .Find the work done on the system in the process of inserting the slab.
View SolutionFigure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
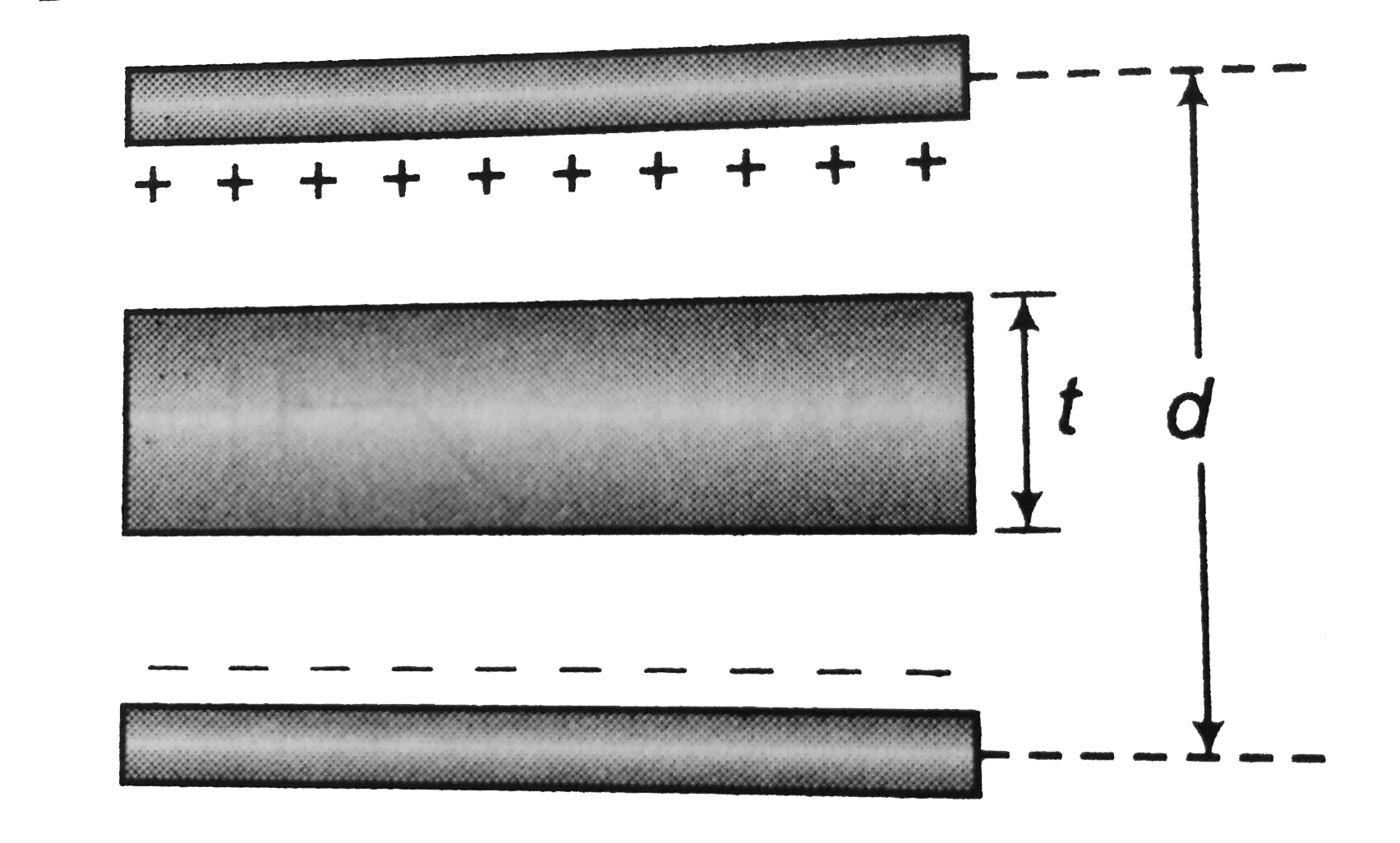
The electric field in the gaps between the plates and the electric slab will be
View SolutionFigue shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
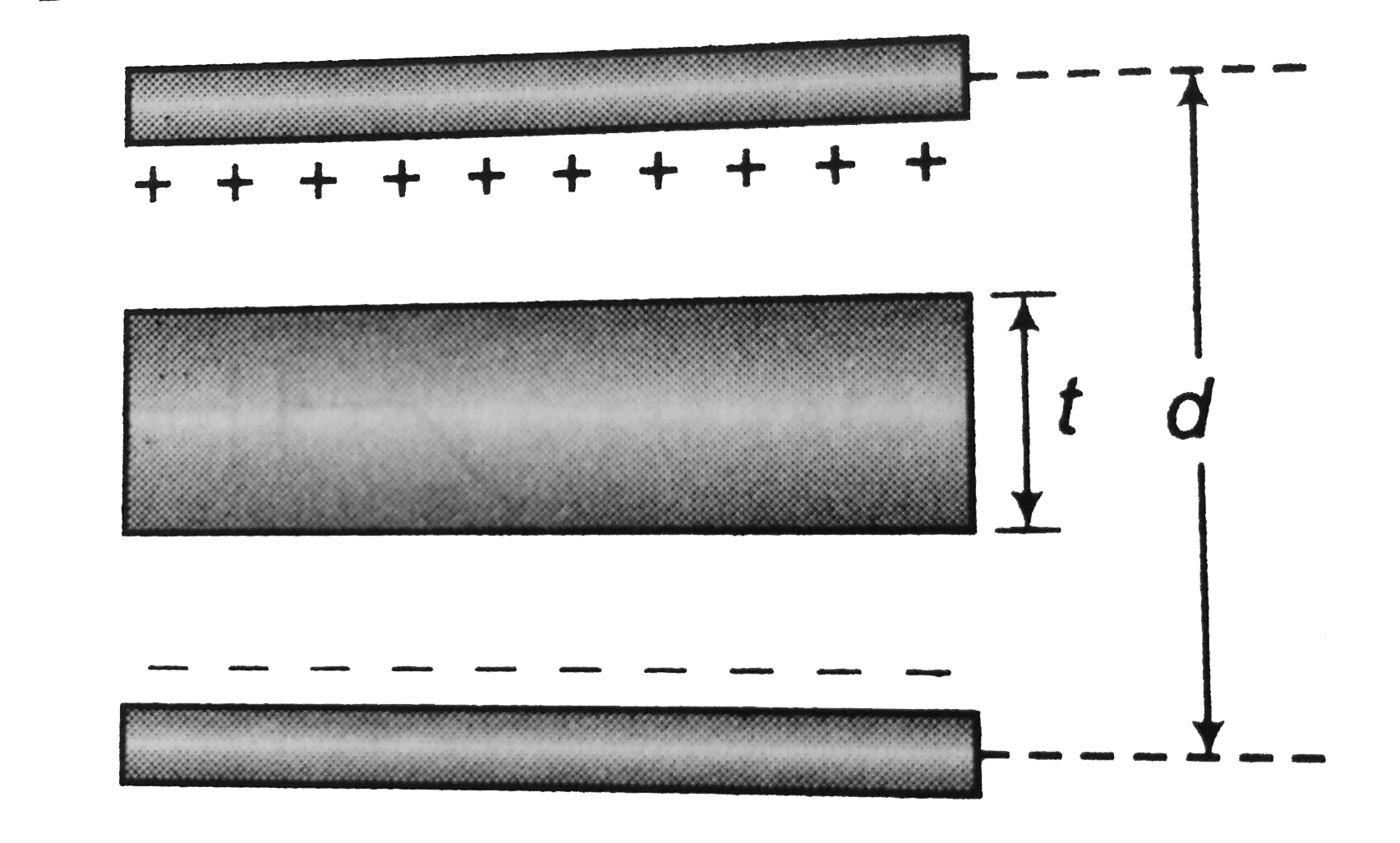
The electric field in the dielectric slab is
View SolutionImagine a light planet revolving around a very massive star in a circular orbit of radius r with direction T. On what power of r, will the square of time period depends if the gravitational force of attraction between the planet and the star is proportional to r−5/2 .
View SolutionA parallel plate air-core capacitor is connected across a source of constant potential difference. When a dielectric plate is introduced between the two plates then:
View SolutionIn a parallel plate capacitor, the distance between the plates is d and potential difference across the plate is V. Energy stored per unit volume between the plates of capacitor is
View SolutionA paller- plate capacitor has plate area 100cm2 and plate separation 1.0cm.A glass plate (dielectric constant 4.0)are inserted one over the other the fill the space between the plate of the capacitor.Find the new capacitance.
View Solution
