A balloon rises up with constant net acceleration of 10m/s2. After 2 s a particle drops from the balloon. After further 2 s match the following : (Takeg=10m/s2)
Column IColumn II(A)Height of perticle from ground(p)Zero(B)Speed of particle(q)10SIunits(C)Displacement of Particle(r)40SIunits(D)Acceleration of particle(s)20SIunits
The correct Answer is:A, B, C, D
To solve the problem step by step, we will analyze the motion of the balloon and the particle dropped from it.
Step 1: Calculate the height of the balloon after 2 seconds.
The balloon rises with a constant acceleration of 10m/s2. We can use the formula for displacement:
h=ut+12at2
where:
- u=0m/s (initial velocity of the balloon),
- a=10m/s2 (acceleration),
- t=2s (time).
Substituting the values:
h=0⋅2+12⋅10⋅(2)2=0+12⋅10⋅4=20m
Step 2: Calculate the speed of the balloon after 2 seconds.
We can use the formula for final velocity:
v=u+at
where:
- u=0m/s,
- a=10m/s2,
- t=2s.
Substituting the values:
v=0+10⋅2=20m/s
Step 3: Analyze the motion of the particle after it is dropped.
When the particle is dropped from the balloon, it has an initial velocity of 20m/s (upward) and will experience a downward acceleration due to gravity (g=10m/s2).
Step 4: Calculate the height of the particle after 2 seconds.
We can use the formula for displacement of the particle:
h=ut−12gt2
where:
- u=20m/s (initial velocity of the particle),
- g=10m/s2,
- t=2s.
Substituting the values:
h=20⋅2−12⋅10⋅(2)2=40−12⋅10⋅4=40−20=20m
Step 5: Calculate the height of the particle from the ground.
Since the balloon was at a height of 20m after 2 seconds, and the particle dropped from it, the total height of the particle from the ground is:
Height from ground=Height of balloon+Height of particle=20+20=40m
Step 6: Calculate the speed of the particle after 2 seconds.
Using the formula for final velocity:
v=u−gt
where:
- u=20m/s,
- g=10m/s2,
- t=2s.
Substituting the values:
v=20−10⋅2=20−20=0m/s
Step 7: Calculate the acceleration of the particle.
The particle is in free fall after being dropped, so it experiences an acceleration equal to g (downward):
Acceleration=g=10m/s2
Summary of Results:
- Height of particle from ground: 40m
- Speed of particle: 0m/s
- Displacement of particle: 20m (from the point of release)
- Acceleration of particle: 10m/s2
Matching with Columns:
- (A) Height of particle from ground: (p) 40SI units
- (B) Speed of particle: (q) 0SI units
- (C) Displacement of particle: (r) 20SI units
- (D) Acceleration of particle: (s) 10SI units
The balloon rises with a constant acceleration of 10m/s2. We can use the formula for displacement:
h=ut+12at2
where:
- u=0m/s (initial velocity of the balloon),
- a=10m/s2 (acceleration),
- t=2s (time).
h=0⋅2+12⋅10⋅(2)2=0+12⋅10⋅4=20m
We can use the formula for final velocity:
v=u+at
where:
- u=0m/s,
- a=10m/s2,
- t=2s.
v=0+10⋅2=20m/s
When the particle is dropped from the balloon, it has an initial velocity of 20m/s (upward) and will experience a downward acceleration due to gravity (g=10m/s2).
We can use the formula for displacement of the particle:
h=ut−12gt2
where:
- u=20m/s (initial velocity of the particle),
- g=10m/s2,
- t=2s.
h=20⋅2−12⋅10⋅(2)2=40−12⋅10⋅4=40−20=20m
Since the balloon was at a height of 20m after 2 seconds, and the particle dropped from it, the total height of the particle from the ground is:
Height from ground=Height of balloon+Height of particle=20+20=40m
Using the formula for final velocity:
v=u−gt
where:
- u=20m/s,
- g=10m/s2,
- t=2s.
v=20−10⋅2=20−20=0m/s
The particle is in free fall after being dropped, so it experiences an acceleration equal to g (downward):
Acceleration=g=10m/s2
- Height of particle from ground: 40m
- Speed of particle: 0m/s
- Displacement of particle: 20m (from the point of release)
- Acceleration of particle: 10m/s2
- (A) Height of particle from ground: (p) 40SI units
- (B) Speed of particle: (q) 0SI units
- (C) Displacement of particle: (r) 20SI units
- (D) Acceleration of particle: (s) 10SI units
Topper's Solved these Questions
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - COMPREHENSION_TYPE
19 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
Similar Questions
For the velocity -time graph shown in figure, in a time interval from t=0 to t=6s, match the following:
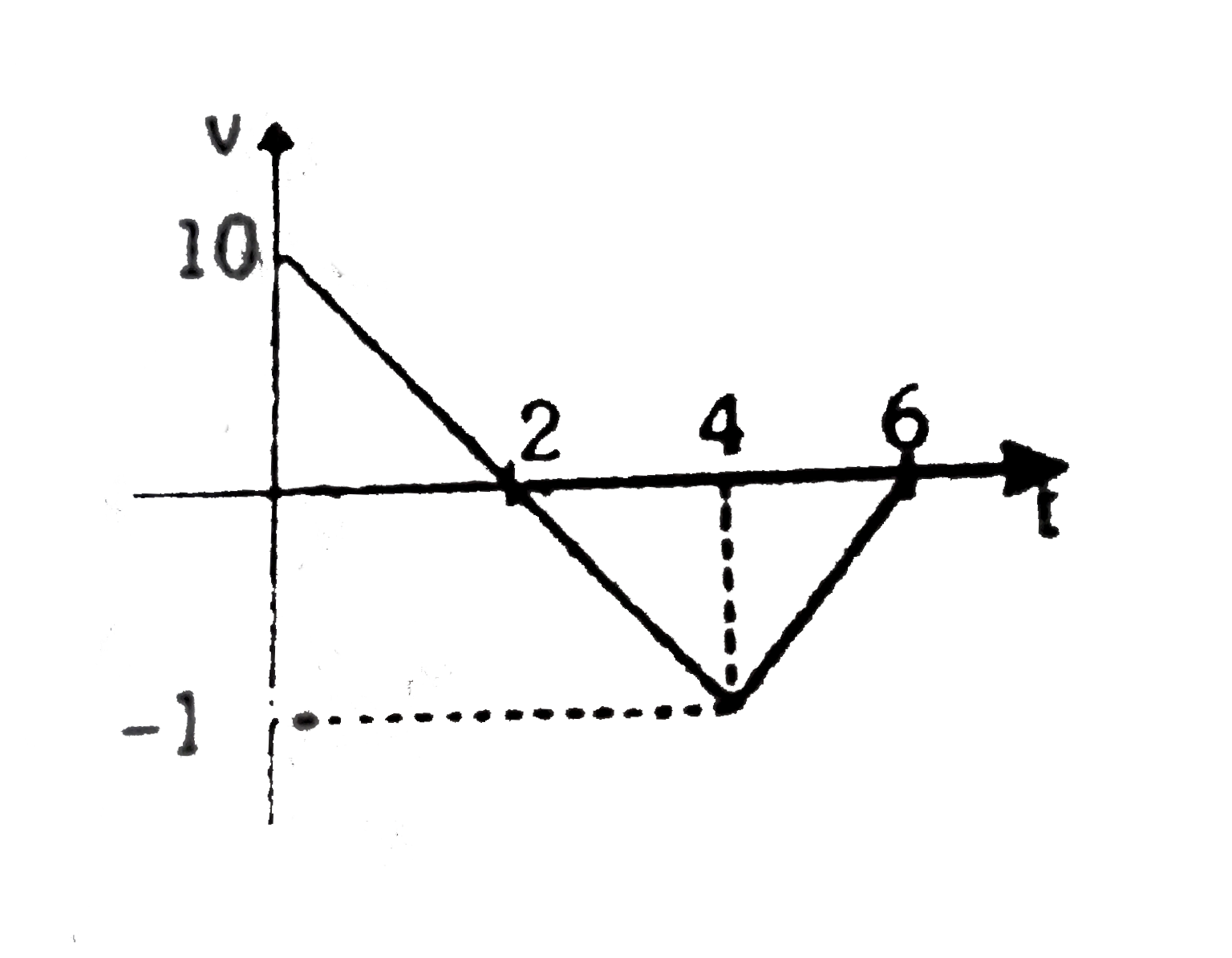
Column IColumn II(A)Change in velocity(p)−5/3SIunit(B)Average acceleration(q)−20SIunit(C)Total displacement(r)−10SIunit(D)Acceleration ay t=3s(s)−5SIunit
View SolutionA balloon starts rising from the ground with a constant acceleration of 1.25m/s2. After 8 s, a stone is released from the balloon. Find the time taken by the stone to reach the ground. (Take g=10m/s2)
View SolutionIn the diagram shown in figure, match the following columns (take g=10ms−2)
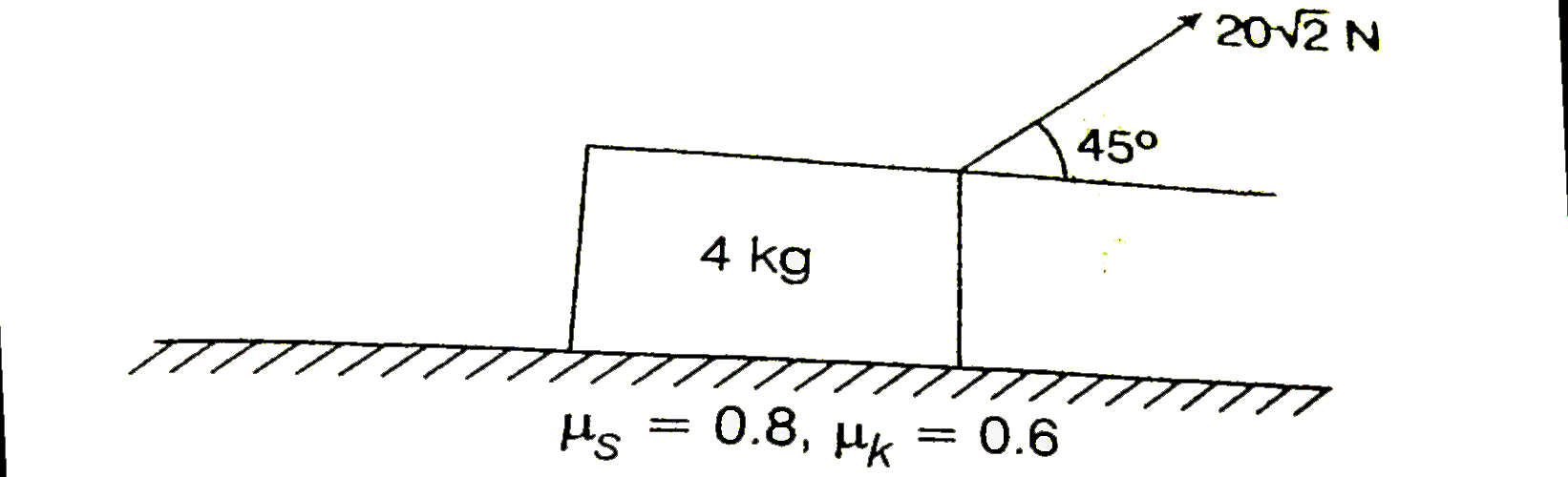
Column IColumn II(A) Normal reaction(p) 12 SI unit(B) Force of friction(q) 20 SI unit(C) Acceleration of block(r) zero(s) 2 SI unit
View SolutionIn the diagram shown in figure, match the following (g=10m/s2)

Column-IColoumn-II(A)Acceleration of 2kg block(P)8 Si unit(B)Net force on 3kg block(Q)25 SI unit(C)Normal reaction between 2 kg and 1kg(R)2 SI unit(D)Normal reaction between 3 kg and 2kg (S) 45 SI unit(T) None
View SolutionIn the s-t equation (s=10+20t−5t2) match the following columns.
∣∣ ∣ ∣ ∣ ∣ ∣∣Column IColumn II(A)Distancec travelled in 3s(p)−20 units(B)Displacement 1 s(q)15 units(C)Initial acceleration(r)25 units(D)Velocity at 4 s(s)−10 units∣∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionA balloon os mass 2 KG is rising up with an acceleration of 4ms2 . The upward force acting on the balloon is (g = 10 ms^-2)
View SolutionA particle of mass 1 kg is projected upwards with velocity 60ms−1. Another particle of mass 2kg is just dropped from a certain height. After 2s, match the following.
[Take, g =10ms−2]
column1column2Accelertion of CMP ZeroB Velocity of CMQ 10 SI unitC Displacement of CMR 20 SI unit-S None
View SolutionA balloon rises rest on the ground with constant acceleration 1ms−2. A stone is dropped when balloon has risen to a height of 39.2m. Find the time taken by the stone to teach the ground.
View SolutionA balloon is going upwards with a constant velocity 15m/s. When the ballooon is at 50 m height, a stone is dropped outside from the balloon. How long will stone take to reach at the ground? (take g=10m/s2)
View SolutionA balloon starts rising from the ground with an acceleration of 1.25 m/s2 . A stone is released from the balloon after 10s. Determine
(1) maximum height of ston from ground
(2) time taken by stone to reach the ground
View Solution
