In a potentiometer wire experiment the emf of a battery in the primary circuit is 20V and its internal resistance is 5Ω. There is a resistance box in series with the battery and the potentiometer wire, whose resistance can be varied from 120Ω to 170Ω. Resistance of the potentiometer wire is 75Ω. The following potential differences can be measured using this potentiometer.
A) 5V
B) 6V
C) 7V
D) 8V
The correct Answer is:A, B, C
To solve the problem step by step, we will calculate the potential difference that can be measured using the potentiometer based on the given values.
Step 1: Identify the given values
- EMF of the battery (E) = 20 V
- Internal resistance of the battery (r) = 5 Ω
- Resistance of the potentiometer wire (R1) = 75 Ω
- Resistance of the resistance box (R) varies from 120 Ω to 170 Ω
Step 2: Calculate the total resistance in the circuit
The total resistance (R_total) in the circuit when the resistance box is set to its minimum value (120 Ω) is:
Rtotal=r+R+R1
Substituting the values:
Rtotal=5Ω+120Ω+75Ω=200Ω
Step 3: Calculate the current when R = 120 Ω
Using Ohm's law, the current (I) in the circuit can be calculated as:
I=ERtotal
Substituting the values:
I=20V200Ω=0.1A
Step 4: Calculate the potential difference across the potentiometer wire when R = 120 Ω
The potential difference (V) across the potentiometer wire can be calculated using:
V=I×R1
Substituting the values:
V=0.1A×75Ω=7.5V
Step 5: Calculate the total resistance in the circuit when R = 170 Ω
Now, calculate the total resistance when the resistance box is set to its maximum value (170 Ω):
Rtotal=r+R+R1
Substituting the values:
Rtotal=5Ω+170Ω+75Ω=250Ω
Step 6: Calculate the current when R = 170 Ω
Using Ohm's law again:
I=ERtotal
Substituting the values:
I=20V250Ω=0.08A
Step 7: Calculate the potential difference across the potentiometer wire when R = 170 Ω
Using the same formula for potential difference:
V=I×R1
Substituting the values:
V=0.08A×75Ω=6V
Final Result
The potential differences that can be measured using the potentiometer range from 6 V (when R = 170 Ω) to 7.5 V (when R = 120 Ω). Therefore, the potential differences that can be measured are between 6 V and 7.5 V.
- EMF of the battery (E) = 20 V
- Internal resistance of the battery (r) = 5 Ω
- Resistance of the potentiometer wire (R1) = 75 Ω
- Resistance of the resistance box (R) varies from 120 Ω to 170 Ω
The total resistance (R_total) in the circuit when the resistance box is set to its minimum value (120 Ω) is:
Rtotal=r+R+R1
Substituting the values:
Rtotal=5Ω+120Ω+75Ω=200Ω
Using Ohm's law, the current (I) in the circuit can be calculated as:
I=ERtotal
Substituting the values:
I=20V200Ω=0.1A
The potential difference (V) across the potentiometer wire can be calculated using:
V=I×R1
Substituting the values:
V=0.1A×75Ω=7.5V
Now, calculate the total resistance when the resistance box is set to its maximum value (170 Ω):
Rtotal=r+R+R1
Substituting the values:
Rtotal=5Ω+170Ω+75Ω=250Ω
Using Ohm's law again:
I=ERtotal
Substituting the values:
I=20V250Ω=0.08A
Using the same formula for potential difference:
V=I×R1
Substituting the values:
V=0.08A×75Ω=6V
The potential differences that can be measured using the potentiometer range from 6 V (when R = 170 Ω) to 7.5 V (when R = 120 Ω). Therefore, the potential differences that can be measured are between 6 V and 7.5 V.
Topper's Solved these Questions
Similar Questions
If a ideal battery of e.m.f.10 V is connected with external resistance 9Ω and a wire of length 10 m and resistance 1Ω in series as shown. Then the potential gradient of wire is
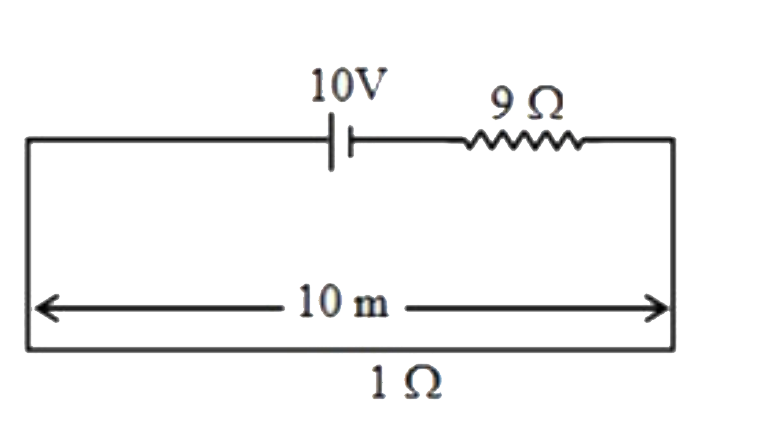 View Solution
View SolutionA 2 V battery, a 990Ω resistor and a potentiometer of 2 m length, all are connected in series of the resistance of potentiometer wire is 10Ω, then the potential gradient of the potentiometer wire is
View SolutionA 2 V battery, a 990Ω resistor and a potentiometer of 2 m length, all are connected in series of the resistance of potentiometer wire is 10Ω, then the potential gradient of the potentiometer wire is
View SolutionIn a potentiometer circuit, the emf of driver cell is 2V and internal resistance is 0.5Ω. The potentiometer wire is 1m long . It is found that a cell of emf 1V and internal resistance 0.5Ω is balanced against 60 cm length of the wire. Calculate the resistance of potentiometer wire.
View SolutionIn order to determine the internal resistance of a primary cell by means of potentiometer the emf of the battery connected across the ends of the potentiometer wire should be
View SolutionThe wire of potentiometer has resistance 4Ω and length 1m. It is connected to a cell of e.m.f. 2V and internal resistance 1Ω. The current flowing through the potentiometer wire is
View SolutionA potentiometer wire has resistance 40Ω and its length is 10m. It is connected by a resistance of 760Ω in series if emf of battery is 2V then potential gradient is:-
View SolutionThe potentiometer wire is of length 1200 cam and it carries a current of 60 mA. For a cell of emf 5V and internal resistance of 20Ω, the null point of it is found to be at 1000 cm.The resistance of potentiometer wire is
View SolutionAn ideal battery of emf 2 V and a series resistance R are connected in the primary circuit of a potentiometer of length 1 m and resistance 5Ω The value of R, to give a potential difference of 5 m V across 10 cm of potentiometer wire is
View SolutionAN ideal battery of 4 V and resistance R are connected in series in the primary circuit of a potentiometer of length 1 m and and resistance 5Ω the value of R, to give a difference of 56mV across 10 cm of potentiometer wire , is :
View Solution

