An ideal battery of emf 2 V and a series resistance R are connected in the primary circuit of a potentiometer of length 1 m and resistance 5Ω The value of R, to give a potential difference of 5 m V across 10 cm of potentiometer wire is
180Ω
190Ω
195Ω
200Ω
The correct Answer is:C
To solve the problem, we need to determine the value of the resistance R that will give a potential difference of 5 mV across 10 cm of a potentiometer wire. Here’s how we can approach this step by step:
Step 1: Understand the relationship between potential difference, length, and total length of the potentiometer wire.
The total length of the potentiometer wire is given as 1 m (or 100 cm). We need to find the potential difference across 10 cm of this wire, which is given as 5 mV.
Step 2: Calculate the potential difference per unit length of the potentiometer wire.
The potential difference across the entire length of the potentiometer wire can be calculated using the formula:
V=V0×lL
where:
- V is the potential difference across the length l,
- V0 is the total voltage across the potentiometer wire,
- l is the length of the wire for which we want to find the potential difference (10 cm),
- L is the total length of the wire (100 cm).
Given that V is 5 mV (or 5×10−3 V), we can rearrange the equation to find V0:
5×10−3=V0×10100
V0=5×10−3×10=5×10−2 V=0.05 V
Step 3: Use Ohm's Law to find the current in the circuit.
The total resistance in the circuit is the sum of the series resistance R and the resistance of the potentiometer wire (5 ohms). The current I through the circuit can be expressed using Ohm's Law:
I=VbatteryR+Rpot
where:
- Vbattery=2 V,
- Rpot=5 ohm.
Thus,
I=2R+5
Step 4: Relate the potential difference across the potentiometer wire to the current and resistance.
The potential difference across the potentiometer wire can also be expressed as:
V=I×Rpot
Substituting the expression for I:
0.05=(2R+5)×5
Step 5: Solve for R.
Rearranging the equation gives:
0.05=10R+5
Cross-multiplying yields:
0.05(R+5)=10
Expanding and simplifying:
0.05R+0.25=10
0.05R=10−0.25
0.05R=9.75
R=9.750.05=195 ohms
Final Answer:
The value of R that will give a potential difference of 5 mV across 10 cm of the potentiometer wire is R=195 ohms.
---
The total length of the potentiometer wire is given as 1 m (or 100 cm). We need to find the potential difference across 10 cm of this wire, which is given as 5 mV.
The potential difference across the entire length of the potentiometer wire can be calculated using the formula:
V=V0×lL
where:
- V is the potential difference across the length l,
- V0 is the total voltage across the potentiometer wire,
- l is the length of the wire for which we want to find the potential difference (10 cm),
- L is the total length of the wire (100 cm).
5×10−3=V0×10100
V0=5×10−3×10=5×10−2 V=0.05 V
The total resistance in the circuit is the sum of the series resistance R and the resistance of the potentiometer wire (5 ohms). The current I through the circuit can be expressed using Ohm's Law:
I=VbatteryR+Rpot
where:
- Vbattery=2 V,
- Rpot=5 ohm.
I=2R+5
The potential difference across the potentiometer wire can also be expressed as:
V=I×Rpot
Substituting the expression for I:
0.05=(2R+5)×5
Rearranging the equation gives:
0.05=10R+5
Cross-multiplying yields:
0.05(R+5)=10
Expanding and simplifying:
0.05R+0.25=10
0.05R=10−0.25
0.05R=9.75
R=9.750.05=195 ohms
The value of R that will give a potential difference of 5 mV across 10 cm of the potentiometer wire is R=195 ohms.
Similar Questions
AN ideal battery of 4 V and resistance R are connected in series in the primary circuit of a potentiometer of length 1 m and and resistance 5Ω the value of R, to give a difference of 56mV across 10 cm of potentiometer wire , is :
View SolutionA potentiometer consists of a wire of length 4 m and resistance 10Ω. It is connected to a cell of emf 2V.The potential gradient of the wire is
View SolutionA current of 2amp is flowing in the primary circuit of a potentiometer wire having resistance of 0.2ohm/m. In a coil when current of 1A flows, then potential difference across its terminals are balanced at 2.5m of the potentiometer wire. The resistance of the coil is
View SolutionIf a ideal battery of e.m.f.10 V is connected with external resistance 9Ω and a wire of length 10 m and resistance 1Ω in series as shown. Then the potential gradient of wire is
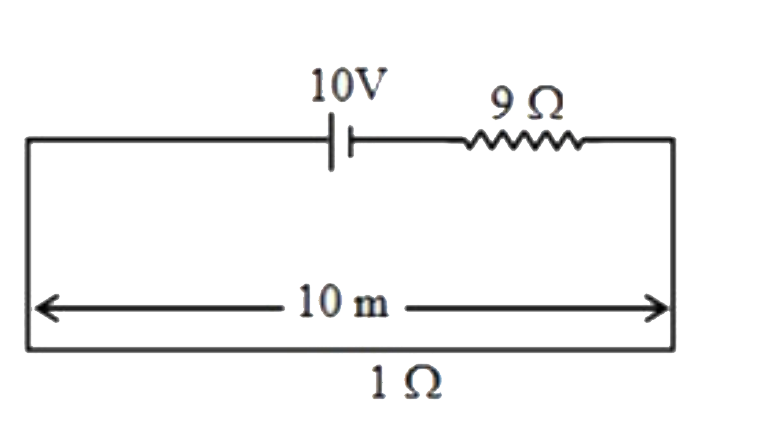 View Solution
View SolutionA battery of e.m.f. 10 V and internal resistance 2Ω is connected in primary circuit with a uniform potentiometer wire and a rheostat whose resistance is fixed at 998Ω.A battery of unknown e.m.f. is being balanced on this potentiometer wire and balancing length is found to be 50 cm.When area of cross section of potentiometer wire is doubled, then balancing length is found to be 75 cm.
(i)Calculate e.m.f. of the battery.
(ii)Calculate resistivity of potentiometer wire if length of wire is 100 cm and area of cross-section (initially) is 100cm2.View SolutionA potentiometer wire of length 10 m and resistance 10Ω per meter is connected in serice with a resistance box and a 2 volt battery. If a potential difference of 100 mV is balanced across the whole length of potentiometer wire, then then the resistance introduce introduced in the resistance box will be
View SolutionA battery of emf 2 V and internal resistance r is connected in series with a resistor of 10Ω through an ammeter of resistance 2Ω. The ammeter reads 50 mA. Draw the circuit diagram and calculate the value of r.
View SolutionCalculate the current flowing through a wire of resistance 7.5 Ω connected to a battery of potential difference 1.5. V.
View SolutionA battery of e.m.f. 10V and internal resistance 0.5ohm is connected across a variable resistance R. The value of R for which the power delivered in it is maximum is given by
View SolutionA potentiometer wire of length 10m and resistance 30Ω is connected in series with a battery of emf 2.5V, internal resistance 5Ω and an external resistance R. If the fall of potential along the potentiometer wire is 50μVmm−1, then the value of R is found to be 23nΩ. What is n?
View Solution

