During adiabatic process pressure (p) versus density (ρ) equation is
pρλ=constant
pρ−λ=constant
pλρ1+λ=constant
p1/λρλ=constant
The correct Answer is:B
To solve the question regarding the relationship between pressure (P) and density (ρ) during an adiabatic process, we can follow these steps:
1. Understand the Adiabatic Process:
In an adiabatic process, there is no heat exchange with the surroundings. The relationship between pressure (P) and volume (V) can be expressed as:
P⋅Vλ=constant
where λ is the adiabatic index (ratio of specific heats).
2. Relate Density to Volume:
Density (ρ) is defined as mass (m) per unit volume (V):
ρ=mV
This implies that volume is inversely proportional to density:
V=mρ
3. Substitute Volume in the Adiabatic Equation:
Substitute the expression for volume (V) in terms of density (ρ) into the adiabatic equation:
P⋅(mρ)λ=constant
This simplifies to:
P⋅mλρλ=constant
4. Rearranging the Equation:
Rearranging the equation gives us:
P⋅ρ−λ=constant
This indicates that the product of pressure and density raised to the power of negative lambda is constant.
5. Conclusion:
From the derived relationship, we can conclude that during an adiabatic process, the equation relating pressure (P) and density (ρ) can be expressed as:
P∝ρ−λ
This means that pressure is inversely proportional to density raised to the power of lambda.
Final Answer:
The correct relationship for pressure versus density during an adiabatic process is:
P⋅ρ−λ=constant
In an adiabatic process, there is no heat exchange with the surroundings. The relationship between pressure (P) and volume (V) can be expressed as:
P⋅Vλ=constant
where λ is the adiabatic index (ratio of specific heats).
Density (ρ) is defined as mass (m) per unit volume (V):
ρ=mV
This implies that volume is inversely proportional to density:
V=mρ
Substitute the expression for volume (V) in terms of density (ρ) into the adiabatic equation:
P⋅(mρ)λ=constant
This simplifies to:
P⋅mλρλ=constant
Rearranging the equation gives us:
P⋅ρ−λ=constant
This indicates that the product of pressure and density raised to the power of negative lambda is constant.
From the derived relationship, we can conclude that during an adiabatic process, the equation relating pressure (P) and density (ρ) can be expressed as:
P∝ρ−λ
This means that pressure is inversely proportional to density raised to the power of lambda.
The correct relationship for pressure versus density during an adiabatic process is:
P⋅ρ−λ=constant
Topper's Solved these Questions
Similar Questions
In adiabatic process
View SolutionIn an adiabatic process
View SolutionIn a adiabatic process.
View SolutionFor an adiabatic process :
View SolutionIn an adiabatic process-
View SolutionDuring adiabatic process, pressure P and density equation is:
View SolutionIn the process PV = constant, pressure (P) versus density (ρ) graph of an ideal gas is
View Solutionp-V diagram of an ideal gas for a process ABC is as shown in the figure.
(a) Find total heat absorbed or released by the gas during the process ABC.
(b) Change in internal energy of the gas during the process ABC.
(c) Plot pressure versus density graph of the gas for the process ABC.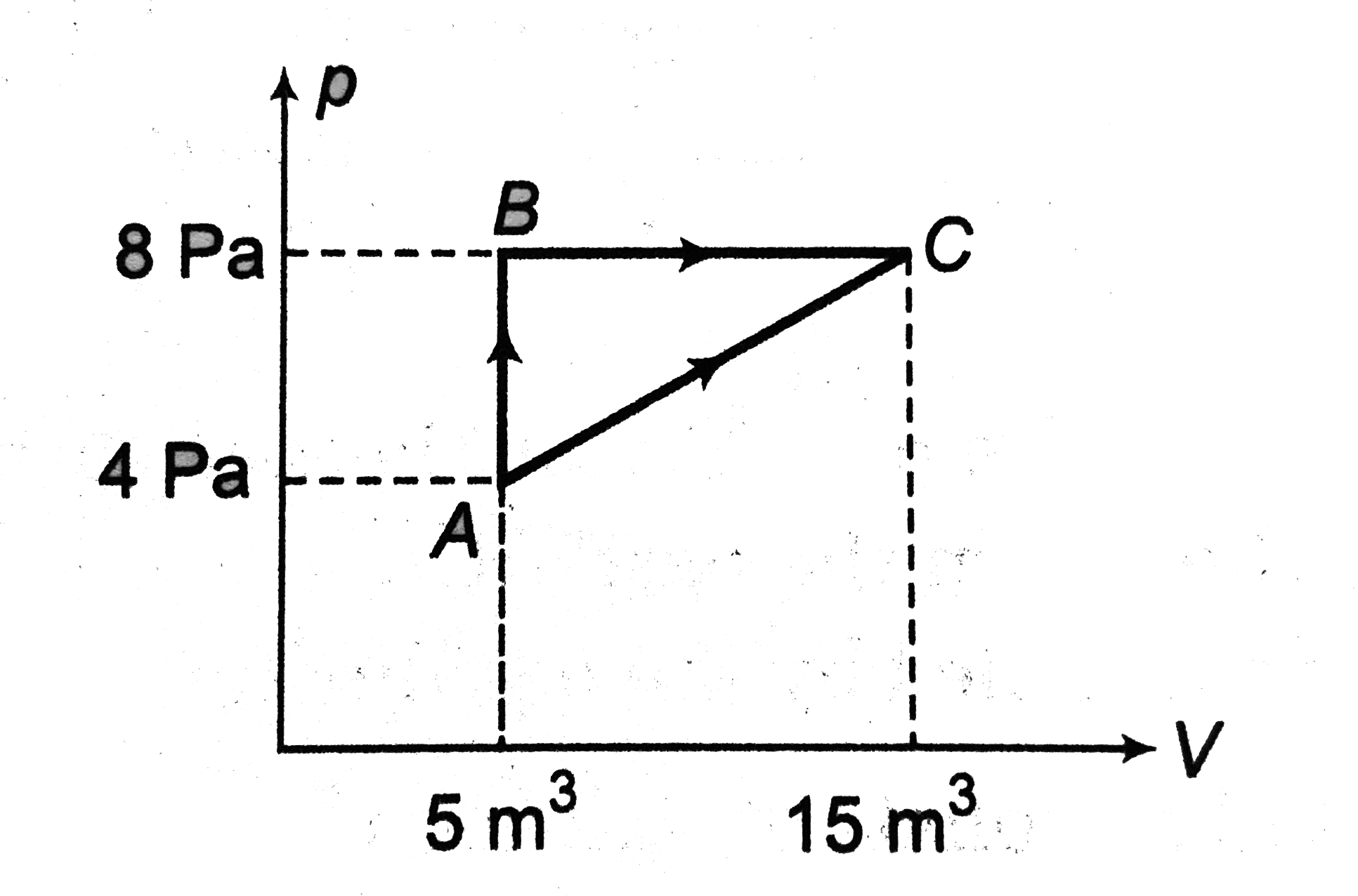
View SolutionDuring an adiabatic process, the pressure of a gas is found to be proportional to the cube of its absolute temperature. The ratio CP/CV for the gas is
View SolutionDuring an adiabatic process, the pressure of a gas is found to be proportional to the cube of its absolute temperature. The ratio CP/CV for the gas is
View Solution
