A certain amount of ideal monoatomic gas undergoes a process given by TV1/2= constant. The molar specific heat of the gas for the process will be given by
R/2
−3R/2
3R/2
−R/2
The correct Answer is:D
To solve the problem, we need to find the molar specific heat of an ideal monoatomic gas undergoing a process defined by the equation TV1/2=constant.
1. Understanding the Process:
We start with the equation given in the problem:
TV1/2=K(1)
where K is a constant.
2. Relating Temperature and Pressure:
We know from the ideal gas law that:
PV=nRT(2)
Rearranging this gives:
T=PVnR(3)
3. Substituting Temperature into the Process Equation:
Substitute equation (3) into equation (1):
(PVnR)V1/2=K
This simplifies to:
PV3/2nR=K
Rearranging gives:
PV3/2=nRK(4)
This shows that PV3/2 is also constant.
4. Identifying the Value of Gamma:
The equation PVγ=constant allows us to identify γ:
γ=32(5)
5. Using the First Law of Thermodynamics:
The first law of thermodynamics states:
δQ=δU+δW(6)
We know that:
δQ=nCΔT(7)
where C is the molar specific heat we want to find.
6. Calculating Change in Internal Energy:
For a monoatomic gas, the change in internal energy is given by:
δU=nCVΔT(8)
where CV=32R for a monoatomic gas.
7. Calculating Work Done:
The work done can be expressed as:
δW=nRΔT⋅11−γ(9)
Substituting γ=32:
δW=nRΔT⋅11−32=nRΔT⋅1−12=−2nRΔT
8. Substituting into the First Law:
Substitute equations (7), (8), and (9) into equation (6):
nCΔT=nCVΔT−2nRΔT
Dividing through by nΔT (assuming ΔT≠0):
C=CV−2R(10)
9. Substituting CV:
Now substitute CV=32R into equation (10):
C=32R−2R=32R−42R=−12R
10. Final Result:
Thus, the molar specific heat C for the process is:
C=−R2
Conclusion:
The molar specific heat of the gas for the process is −R2.
We start with the equation given in the problem:
TV1/2=K(1)
where K is a constant.
We know from the ideal gas law that:
PV=nRT(2)
Rearranging this gives:
T=PVnR(3)
Substitute equation (3) into equation (1):
(PVnR)V1/2=K
This simplifies to:
PV3/2nR=K
Rearranging gives:
PV3/2=nRK(4)
This shows that PV3/2 is also constant.
The equation PVγ=constant allows us to identify γ:
γ=32(5)
The first law of thermodynamics states:
δQ=δU+δW(6)
We know that:
δQ=nCΔT(7)
where C is the molar specific heat we want to find.
For a monoatomic gas, the change in internal energy is given by:
δU=nCVΔT(8)
where CV=32R for a monoatomic gas.
The work done can be expressed as:
δW=nRΔT⋅11−γ(9)
Substituting γ=32:
δW=nRΔT⋅11−32=nRΔT⋅1−12=−2nRΔT
Substitute equations (7), (8), and (9) into equation (6):
nCΔT=nCVΔT−2nRΔT
Dividing through by nΔT (assuming ΔT≠0):
C=CV−2R(10)
Now substitute CV=32R into equation (10):
C=32R−2R=32R−42R=−12R
Thus, the molar specific heat C for the process is:
C=−R2
The molar specific heat of the gas for the process is −R2.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The specific heat of a gas in an isothermal process is
AinfiniteBzeroCnegativeDremains constant
A monoatomic gas undergoes a process given by 2dU+3dW=0, then what is the process
View SolutionOne mole of an ideal monatomic gas undergoes a process described by the equation PV3= constant. The heat capacity of the gas during this process is
View SolutionTempareture and volume of one mole of an ideal momatomic gas in a process are related as TV23=K ,where k is constant.The molar specific heat capacity for the process is
View Solutionan ideal diatomic gas undergoes a polytropic process described by the equation P√V=constant . The molar heat capacity of the gas during this process is
View SolutionA certain amount of an ideal monoatomic gas undergoes a thermodynamic process such that VT2= constat where V= volume of gas, T= temperature of gas. Then under process
View SolutionOne mole of a diatomic gas undergoes a thermodynamic process, whose process equation is P∝V2. The molar specific heat of the gas is
View SolutionOne mole of a monoatomic ideal gas undergoes the process A→B in the given P-V diagram. What is the specific heat for this process?
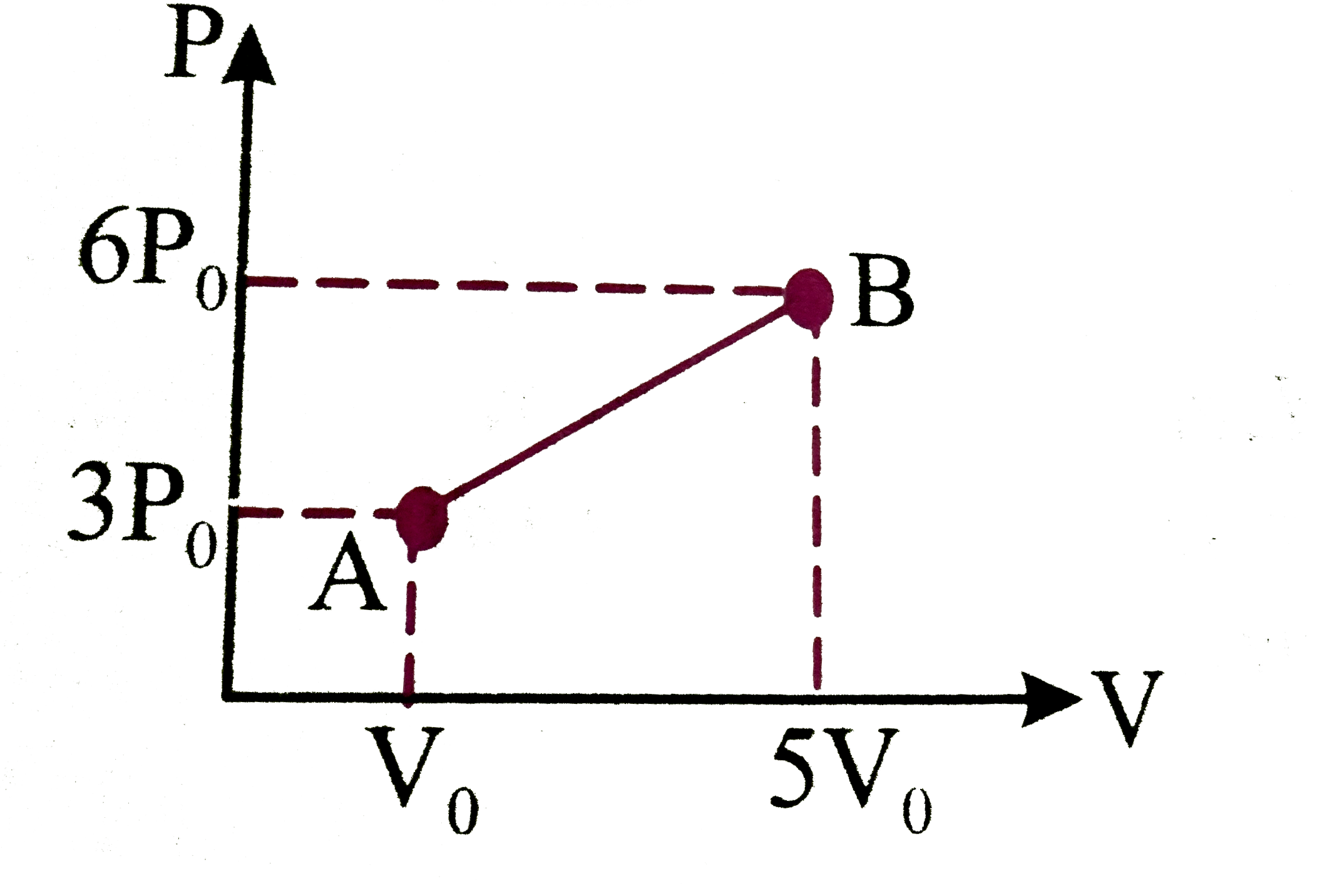
View Solution