Three concentric spherical shells have radii a,b and c(a<b<c) and have surface charge densities σ,−σ and σ respectively. If VA,VB and VC denote the potentials of the three shells, then for c=q+b, we have
VC=VA≠VB
VC=VB≠VA
VC≠VB≠VA
VC=VB=VA
The correct Answer is:A
To solve the problem of finding the potentials VA, VB, and VC for three concentric spherical shells with given surface charge densities, we can follow these steps:
Step 1: Understand the Setup
We have three concentric spherical shells with radii a, b, and c (where a<b<c). The surface charge densities are:
- Shell at radius a: σ
- Shell at radius b: −σ
- Shell at radius c: σ
Step 2: Calculate the Potential VA
The potential VA at radius a is due to the charges on all three shells. The contributions to the potential from each shell are:
- From shell at a: Va=k⋅σ⋅4πa2/a=k⋅σ⋅4πa
- From shell at b: Vb=k⋅(−σ)⋅4πb2/a=−k⋅σ⋅4πb2/a
- From shell at c: Vc=k⋅σ⋅4πc2/a=k⋅σ⋅4πc2/a
Thus, the total potential at point A is:
VA=k⋅σ⋅4πa−k⋅σ⋅4πb2a+k⋅σ⋅4πc2a
Factoring out k⋅σ⋅4π:
VA=k⋅σ⋅4π(a−b2a+c2a)
Step 3: Calculate the Potential VB
For the potential VB at radius b, we consider contributions from the shells at a and c:
- From shell at a: Va=k⋅σ⋅4πa
- From shell at b: Vb=−σ⋅4πb2/b=−k⋅σ⋅4πb
- From shell at c: Vc=k⋅σ⋅4πc2/b
Thus, the total potential at point B is:
VB=k⋅σ⋅4πa−k⋅σ⋅4πb+k⋅σ⋅4πc2b
Factoring out k⋅σ⋅4π:
VB=k⋅σ⋅4π(a−b+c2b)
Step 4: Calculate the Potential VC
For the potential VC at radius c, we consider contributions from all shells:
- From shell at a: Va=k⋅σ⋅4πa
- From shell at b: Vb=−k⋅σ⋅4πb
- From shell at c: Vc=k⋅σ⋅4πc
Thus, the total potential at point C is:
VC=k⋅σ⋅4πa−k⋅σ⋅4πb+k⋅σ⋅4πc
Factoring out k⋅σ⋅4π:
VC=k⋅σ⋅4π(a−b+c)
Step 5: Analyze the Relationship
Given that c=a+b, we can substitute c into our expressions for VA, VB, and VC:
- From the expression for VA:
VA=k⋅σ⋅4π(a−b2a+(a+b)2a)
- From the expression for VB:
VB=k⋅σ⋅4π(a−b+(a+b)2b)
- From the expression for VC:
VC=k⋅σ⋅4π(a−b+(a+b))=k⋅σ⋅4π(2a)
Conclusion
From the calculations, we find that:
- VA and VC are equal.
- VB is different from VA and VC.
We have three concentric spherical shells with radii a, b, and c (where a<b<c). The surface charge densities are:
- Shell at radius a: σ
- Shell at radius b: −σ
- Shell at radius c: σ
The potential VA at radius a is due to the charges on all three shells. The contributions to the potential from each shell are:
- From shell at a: Va=k⋅σ⋅4πa2/a=k⋅σ⋅4πa
- From shell at b: Vb=k⋅(−σ)⋅4πb2/a=−k⋅σ⋅4πb2/a
- From shell at c: Vc=k⋅σ⋅4πc2/a=k⋅σ⋅4πc2/a
VA=k⋅σ⋅4πa−k⋅σ⋅4πb2a+k⋅σ⋅4πc2a
Factoring out k⋅σ⋅4π:
VA=k⋅σ⋅4π(a−b2a+c2a)
For the potential VB at radius b, we consider contributions from the shells at a and c:
- From shell at a: Va=k⋅σ⋅4πa
- From shell at b: Vb=−σ⋅4πb2/b=−k⋅σ⋅4πb
- From shell at c: Vc=k⋅σ⋅4πc2/b
VB=k⋅σ⋅4πa−k⋅σ⋅4πb+k⋅σ⋅4πc2b
Factoring out k⋅σ⋅4π:
VB=k⋅σ⋅4π(a−b+c2b)
For the potential VC at radius c, we consider contributions from all shells:
- From shell at a: Va=k⋅σ⋅4πa
- From shell at b: Vb=−k⋅σ⋅4πb
- From shell at c: Vc=k⋅σ⋅4πc
VC=k⋅σ⋅4πa−k⋅σ⋅4πb+k⋅σ⋅4πc
Factoring out k⋅σ⋅4π:
VC=k⋅σ⋅4π(a−b+c)
Given that c=a+b, we can substitute c into our expressions for VA, VB, and VC:
- From the expression for VA:
VA=k⋅σ⋅4π(a−b2a+(a+b)2a)
- From the expression for VB:
VB=k⋅σ⋅4π(a−b+(a+b)2b)
- From the expression for VC:
VC=k⋅σ⋅4π(a−b+(a+b))=k⋅σ⋅4π(2a)
From the calculations, we find that:
- VA and VC are equal.
- VB is different from VA and VC.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.3
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.4
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.1
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
Three concentric spherical metallic spheres A,B and C of radii a,b and c(a<b<c) have surface charge densities σ,−σ and σ respectively.
View SolutionThree concentric spherical metallic shells A,B,andC of radii a,b,andc(a<b<c) have surface charge densities σ,−σ and σ, respectively.
If VA,VB and VC are potential of shells A, B and C, respectively, match the columns
Column AColumn Ba.VAi.σε0[a2−b2+c2c]b.VBii.σ2ε0[a2b−b+c]c.VCiii.σε0[a−b+c]
View Solution
Knowledge Check
Three concentric spherical shells have radii a,b and c(a<b<c) and have surface charge densities σ,−sigam and σ respectively. If VA,VB and VC denote the potentials of the three shells, then for c=a+b, we have
AVC=VB=VABVC=VA≠VBCVC=VB≠VADVC≠VB≠VA
Three concentric metallic spherical shells A,B and C of radii a,b and c(a<b<c) have surface charge densities +σ,−σand +σ respectively ,the potential of shell B is
View SolutionThree concentric metallic spherical shell A,B and C or radii a,b and c(a<b<c) have surface charge densities −σ,+σand−σ. Respectively. The potential of shel A is :
View SolutionThree concentric spherical metallic shells A,B and C of radii a,b and c (a<b<c) have surface charge densities σ,−σandσ respectively.
If the potential of shell B is VB=σ∈0(anb−b+c) and the potential of shell C is VC=σ∈0(anc−bnc+c) then n is .
View SolutionThree concentric spherical metal shells A.B.C of radii a,b,c(c>b>a) have surface charge density +σ,−σand+σ respectively. The potential of the middle shell is σε0 times
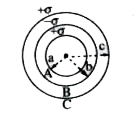
View SolutionThree concentric charged metallic spherical shells A, B and C have radii a,b and c, charge densities σ,−σ and σ and potentials VA,VB and VC respectively. Then which of the following relations is correct?
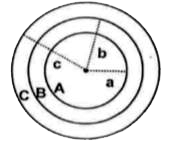
View SolutionA charge Q is distributed over three concentric spherical shells of radii a, b, c (a< b < c) such that their surface charge densities are equal to one another.
The total potential at a point at distance r from their common centre, where r<a, would be :
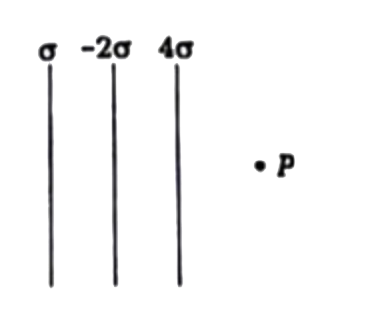
View SolutionThree non-conducting large parallel plates have surface charge densities σ,−2σand4σ respectively as shown in the figure. The electric field at the point P is
View Solution
