A disc is rotating with angular velocity ω. A force F acts at a point whose position vector with respect to the axis of rotation is r. The power associated with torque due to the force is given by
(r×F).ω
(r×F)×ω
r×(F.ω)
r.(F×ω)
The correct Answer is:A
To solve the problem, we need to find the power associated with the torque due to the force acting on a rotating disc. Let's break down the steps to arrive at the solution.
1. Understand the System:
- We have a disc rotating with an angular velocity ω.
- A force F is applied at a point whose position vector with respect to the axis of rotation is R.
2. Define Torque:
- The torque τ due to the force F acting at point R is given by the cross product:
τ=R×F
3. Define Power:
- The power P associated with the torque is defined as the dot product of the torque vector and the angular velocity vector:
P=τ⋅ω
4. Substitute Torque into Power Equation:
- Substitute the expression for torque into the power equation:
P=(R×F)⋅ω
5. Final Expression:
- Thus, the power associated with the torque due to the force is:
P=R×F⋅ω
6. Identify the Correct Option:
- The correct expression for power P is R×F⋅ω, which matches with option 1.
Conclusion:
The power associated with the torque due to the force is given by:
P=R×F⋅ω
Thus, the correct answer is option 1.
- We have a disc rotating with an angular velocity ω.
- A force F is applied at a point whose position vector with respect to the axis of rotation is R.
- The torque τ due to the force F acting at point R is given by the cross product:
τ=R×F
- The power P associated with the torque is defined as the dot product of the torque vector and the angular velocity vector:
P=τ⋅ω
- Substitute the expression for torque into the power equation:
P=(R×F)⋅ω
- Thus, the power associated with the torque due to the force is:
P=R×F⋅ω
- The correct expression for power P is R×F⋅ω, which matches with option 1.
The power associated with the torque due to the force is given by:
P=R×F⋅ω
Thus, the correct answer is option 1.
Topper's Solved these Questions
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - Check point 9.3
15 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (A) Chapter Exercises
83 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - Check point 9.1
20 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
Similar Questions
A disc is rotating with angular velocity ω. If a child sits on it, what is conserved?
View SolutionA rod is rotating with angular velocity ω about axis AB. Find cosθ.

View SolutionA body rotating with angular velocity ω stops after rotating for 2 sec. The torque necessary for this is?
View SolutionA circular disc is rotating about its own axis at an angular velocity ω. If P is exact midpoint of dise between axis and rim of disc then angular velocity of Pis
View SolutionA metal rod length l rotates about on end with a uniform angular velocity ω. A uniform magnetic field →B exists in the direction of the axis of rotation. Calculate the emf induced between the ends of the rod. Neglect the centripetal force acting on the free electrons as they moving in circular paths.
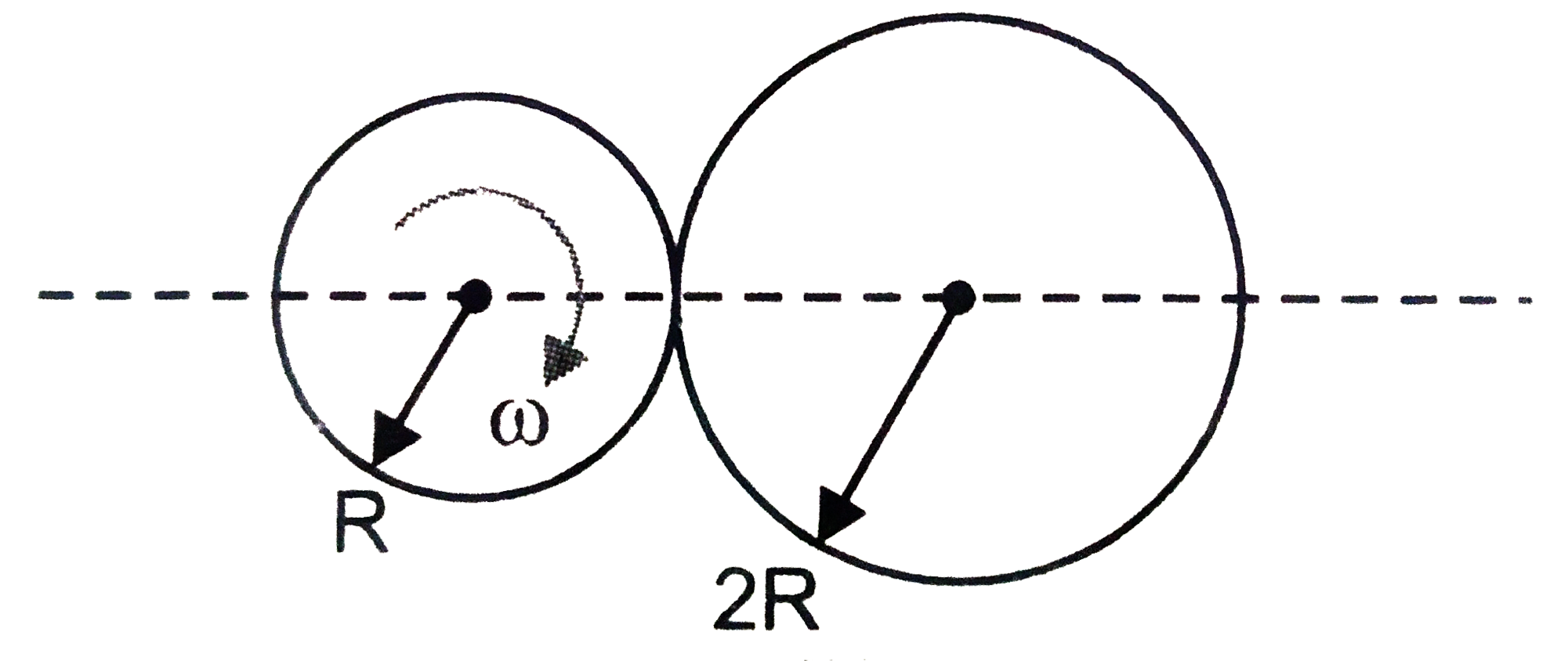
View SolutionTwo discs of radii Rand2R are pressed against each other. Initially, disc with radius R is rotating with angular velocity ω and other disc is stationary. Both discs are hinged at their respective centres and are free to rotate about them. Moment of inertia of smaller disc is I and of bigger disc is 2I about their respective axis of rotation. Find the angular velocity of bigger disc after long time.
View SolutionTwo discs of same moment of inertia rotating their regular axis passing through centre and perpendicular to the plane of disc with angular velocities ω1 and ω2. They are brought into contact face to the face coinciding the axis of rotation. The expression for loss of enregy during this process is :
View SolutionTwo discs of same moment of inertia rotating about their regular axis passing through centre and perpendicular to the plane of disc with angular velocities ω1 and ω2. They are brought into contact face to face coinciding the axis of rotation. The expression for loss of energy during this process is
View SolutionThe force 7ˆi+3ˆj−5ˆk acts on a particle whose position vector is ˆi−ˆj+ˆk. What is the torque of a given force about the origin ?
View SolutionA force →F=4ˆi−10ˆj acts on a body at a point having position vector −5ˆi−3ˆj relative to origin of co-ordinates on the axis of rotation. The torque acting on the body is
View Solution
