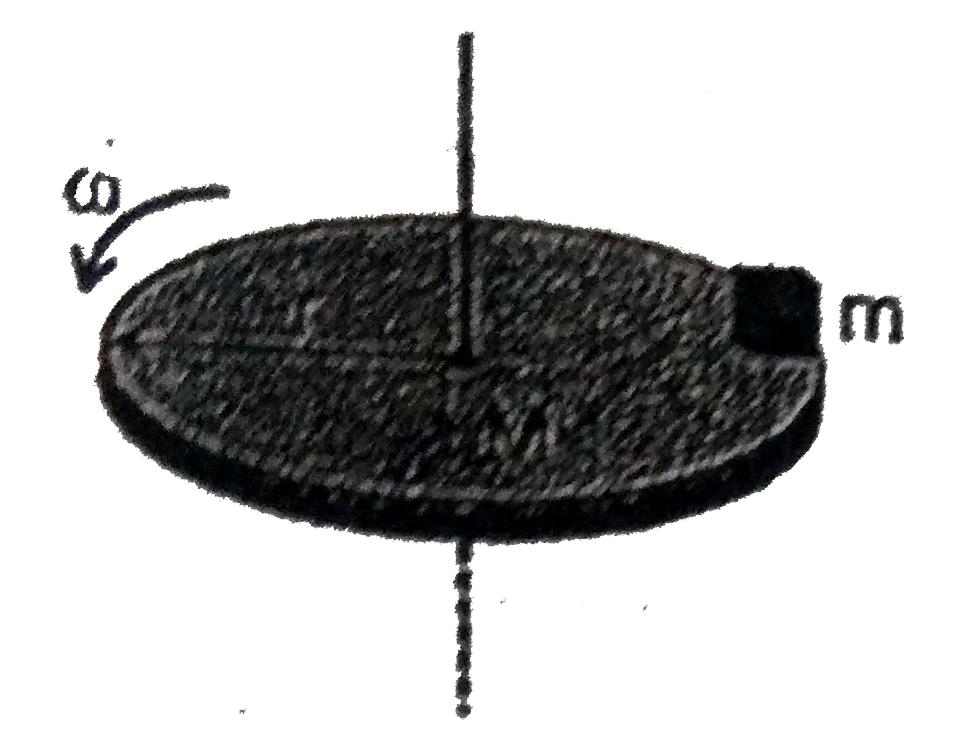
A uniform disc of radius a and mass m, is rotating freely with angular speed ω in a horizontal plane about a smooth fixed vertical axis through its centre. A particle, also of mass m, is suddenly attached to the rim of the disc of the disc and rotates with it. The new angular speed is
ω6
ω3
ω2
ω5
The correct Answer is:B
To solve the problem of finding the new angular speed of a uniform disc after a particle is attached to it, we will follow these steps:
Step 1: Determine the initial moment of inertia of the disc
The moment of inertia I of a uniform disc about an axis through its center is given by the formula:
I=12ma2
where m is the mass of the disc and a is its radius.
Step 2: Calculate the initial angular momentum
The initial angular momentum Li of the disc can be calculated using the formula:
Li=Iω
Substituting the expression for I:
Li=(12ma2)ω
Step 3: Determine the moment of inertia after the particle is attached
When a particle of mass m is attached to the rim of the disc, the new moment of inertia I′ of the system (disc + particle) becomes:
I′=I+Iparticle=12ma2+ma2
The moment of inertia of the particle about the same axis is ma2. Therefore:
I′=12ma2+ma2=32ma2
Step 4: Apply the conservation of angular momentum
Since there is no external torque acting on the system, the angular momentum is conserved:
Li=Lf
where Lf is the final angular momentum after the particle is attached, given by:
Lf=I′ω′
Setting the initial and final angular momentum equal gives:
12ma2ω=32ma2ω′
Step 5: Solve for the new angular speed ω′
We can cancel ma2 from both sides of the equation:
12ω=32ω′
Rearranging gives:
ω′=13ω
Conclusion
The new angular speed after the particle is attached to the rim of the disc is:
ω′=ω3
The moment of inertia I of a uniform disc about an axis through its center is given by the formula:
I=12ma2
where m is the mass of the disc and a is its radius.
The initial angular momentum Li of the disc can be calculated using the formula:
Li=Iω
Substituting the expression for I:
Li=(12ma2)ω
When a particle of mass m is attached to the rim of the disc, the new moment of inertia I′ of the system (disc + particle) becomes:
I′=I+Iparticle=12ma2+ma2
The moment of inertia of the particle about the same axis is ma2. Therefore:
I′=12ma2+ma2=32ma2
Since there is no external torque acting on the system, the angular momentum is conserved:
Li=Lf
where Lf is the final angular momentum after the particle is attached, given by:
Lf=I′ω′
Setting the initial and final angular momentum equal gives:
12ma2ω=32ma2ω′
We can cancel ma2 from both sides of the equation:
12ω=32ω′
Rearranging gives:
ω′=13ω
The new angular speed after the particle is attached to the rim of the disc is:
ω′=ω3
Topper's Solved these Questions
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (B) Chapter Exercises
25 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - Check point 9.3
15 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
Similar Questions
A uniform rod of mass m and length L lies radialy on a disc rotating with angular speed ω in a horizontal plane about vertical axis passing thorugh centre of disc. The rod does not slip on the disc and the centre of the rod is at a distance 2L from the centre of the disc. them the kinetic energy of the rod is

View SolutionA disc of mass M and Radius R is rolling with an angular speed ω on the horizontal plane. The magnitude of angular momentum of the disc about origin is:
View SolutionA smoothh disc is rotating with uniform angular speed ω about a fixed vertical axis passing through its centre and normal to its plane as shown. A small block of mass m is gently placed at the periphery of the disc. Then (pickup the correct alternative or alternatives)
View SolutionA uniform brass disc of radius a and mass m is set into spinning with angular speed ω0 about an axis passing through centre of disc and perpendicular to the palne of disc. If its temperature increases from θ∘1C to θ∘2C with out disturbing the disc, what will be its new angular speed ?
View SolutionA disc of mass m, radius r and carrying charge q, is rotating with angular speed ω about an axis passing through its centre and perpendicular to its plane. Calculate its magnetic moment
View SolutionA disc of mass m, radius r and carrying charge q, is rotating with angular speed ω about an axis passing through its centre and perpendicular to its plane. Calculate its magnetic moment
View SolutionA disc of mass m and radius R rotating with angular speed ω0 is placed on a rough surface (co-officient of friction =μ). Then

View SolutionA uniform disc is rotating at a constantt speed in a vertical plane about a fixed horizontal axis passing through the centre of the disc. A piece of the disc from its rim detaches itself from the disc at the instant when it is at horizontal level with the centre of the disc and moving upward. Then about the fixed axis, the angular speed of the
View SolutionAn uniform disc is rotating at a constant speed in a vertical plane about a fixed horizontal axis passing through the centre of the disc. A piece of the disc from its rim detaches itself from the disc at the instant when it is at horizontal level with the centre of the disc and moving upwards, then about the fixed axis.
STATEMENT-1 : Angular speed of the disc about the aixs of rotation will increase.
and
STATEMENT-2 : Moment of inertia of the disc is decreased about the axis of rotation.
View SolutionA horizontal disc rotates freely with angular velocity ω about a vertical axes through its centre. A ring having the same mass and radius as the disc, is now gently placed coaxially on the disc. After some time, the two rotate with a common angular velocity. then.
View Solution
