Match the following columns,
Column1Column2a Thermal resitancep [MT^(-3)K^(-4)]b Stefan's constantq [M^(-1)L^(-2)T^(3)K]c Wien's constantr [ML^(2)T^(-3)]d Heat currents [LK]
The correct Answer is:A, B, C, D
To solve the problem of matching the physical quantities in Column 1 with their respective dimensions in Column 2, we will analyze each quantity step by step.
1. Thermal Resistance (A):
- The unit of thermal resistance is Kelvin per watt (K/W).
- Power (watt) can be expressed as work done per unit time, which has the dimension of ML2T−3.
- Therefore, the dimension of thermal resistance can be calculated as:
Thermal Resistance=KPower=KML2T−3=M−1L−2T3K
- This matches with q(M−1L−2T3K).
- Match: A → q
2. Stefan's Constant (B):
- The unit of Stefan's constant is watts per square meter per Kelvin to the fourth power (W/m²K⁴).
- Substituting the dimension of power:
Stefan's Constant=ML2T−3L2K4=ML0T−3K−4
- This simplifies to MT−3K−4.
- Match: B → p (M^(-1)L^(-2)T^(3)K)
3. Wien's Constant (C):
- Wien's displacement law relates wavelength (λ) and temperature (T). The dimension of wavelength is length (L), and temperature is in Kelvin (K).
- Therefore, the dimension of Wien's constant is:
Wien's Constant=LK
- Match: C → s (LK)
4. Heat Current (D):
- Heat current (H) can be expressed as the change in temperature (ΔT) divided by thermal resistance (Rth).
- The dimension of ΔT is K, and we already calculated the dimension of thermal resistance as M−1L−2T3K.
- Therefore, the dimension of heat current is:
Heat Current=KM−1L−2T3K=ML2T−3
- Match: D → r (ML^(2)T^(-3))
Final Matches:
- A → q
- B → p
- C → s
- D → r
- The unit of thermal resistance is Kelvin per watt (K/W).
- Power (watt) can be expressed as work done per unit time, which has the dimension of ML2T−3.
- Therefore, the dimension of thermal resistance can be calculated as:
Thermal Resistance=KPower=KML2T−3=M−1L−2T3K
- This matches with q(M−1L−2T3K).
- Match: A → q
- The unit of Stefan's constant is watts per square meter per Kelvin to the fourth power (W/m²K⁴).
- Substituting the dimension of power:
Stefan's Constant=ML2T−3L2K4=ML0T−3K−4
- This simplifies to MT−3K−4.
- Match: B → p (M^(-1)L^(-2)T^(3)K)
- Wien's displacement law relates wavelength (λ) and temperature (T). The dimension of wavelength is length (L), and temperature is in Kelvin (K).
- Therefore, the dimension of Wien's constant is:
Wien's Constant=LK
- Match: C → s (LK)
- Heat current (H) can be expressed as the change in temperature (ΔT) divided by thermal resistance (Rth).
- The dimension of ΔT is K, and we already calculated the dimension of thermal resistance as M−1L−2T3K.
- Therefore, the dimension of heat current is:
Heat Current=KM−1L−2T3K=ML2T−3
- Match: D → r (ML^(2)T^(-3))
- A → q
- B → p
- C → s
- D → r
Topper's Solved these Questions
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Assertion and reason
17 Videos
- CALORIMETRY & HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY & HEAT TRANSFEREXERCISE - Level 2 Subjective
14 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery
27 Videos
Similar Questions
Match the following columns, Column1Coloumn2a Specific heatp [MLT^(-3)K^(-1)]b Coefficient of thermal conductivityq [MT^(-3)K^(-4)]c Boltzmann constantr [L^(2)T^(-2)K^(-1)]d Stefan's constats [ML^(2)T^(-2)K^(-1)]
View SolutionMatch the following columns.
Column IColumn IIA.Teslap[ML2A−2T−2]B.Weberq[MLA−2T−1]C.Weber m−2r.[MA−1T−2]D.Henrys.None
View SolutionMatch the following two columns.
Column IColumn IIA.Electrical resistance1.[ML3T−3A−2]B.Electrical potential2.[ML2T−3A−2]C.Specific resistance3.[ML2T−3A−1]D.Specific conductance4.None
View SolutionIn the diagram shown in figure mass of both the balls in same. Match the following columns.
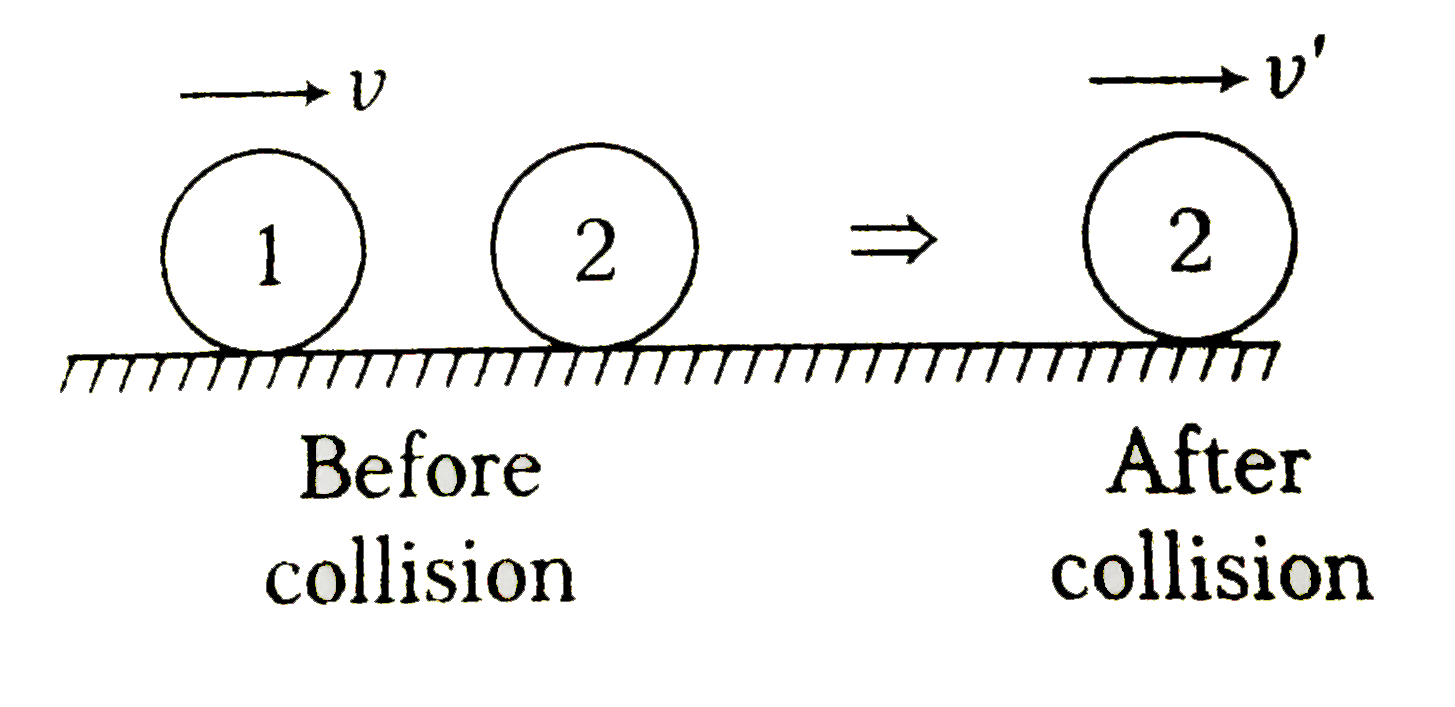
Column1Column2A Forv′=vP e=0B Forv′=v/2Q e=1C Forv′=3/4vR e=1/2-(S data is insufficient
View SolutionFour point masses are placede at four corners of a square of side 4m as shown. Match the following columns.
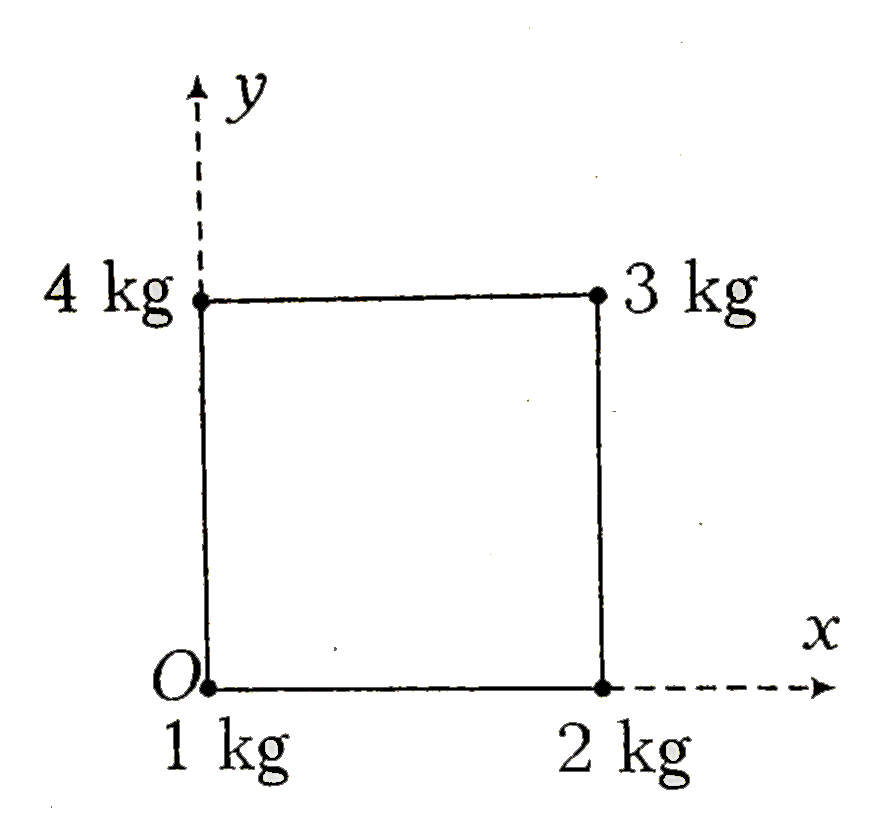
Column1Column2A x-coordinate of center of mass of 4kg and 2kgP (7//2)mB x-coordinates of center of mass of 4kg 2kg and 3kgQ (4//3)mC y-coordinate of center of mass of 1kg 4kg and 3kgR 3my-coordinate of center of mass of 1kg and 3kg(20/9)m
View SolutionColumn IColumn II(A)Electrical resistance(p)[M−1L−2T4A2](B)Capacitance(q)[ML2T−2A−2](C)Magnetic field(r)[ML2T−3A−2](D)Inductance(s)[MT−2A−1]
View SolutionMatch the following.
∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)Coefficient of viscosity(P)[M2L−1T−2](B)Surface tension(Q)[ML0T−2](C)Modulus of elasticity(R)[ML−1T−2](D)Energy per unit volume(S)Noneof a fiuid∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionMatch the following
Column-IColumn-II(A)Kepler's first law(p)T2∝r3(B)Kepler's second law(q)Areal velocity is constant(C)Kepler's third law(r)Orbit of planet is elliptical
View SolutionMatch List I with List II and select the correct answer using the codes given below the lists:
List IList IIP.Boltzmann constant1.[ML2T−1]Q.Coefficient of viscosity2.[ML−1T−1]R.Planck constant3.[MLT−3K−1]S.Thermal conductivity4.[ML2T−2K−1]
View SolutionColumn IColumn II(A)Work(p)[A1/2T−1](B)Moment of inertia(q)[FA1/2](C)Velocity(r)[FA1/2T2]
View Solution
