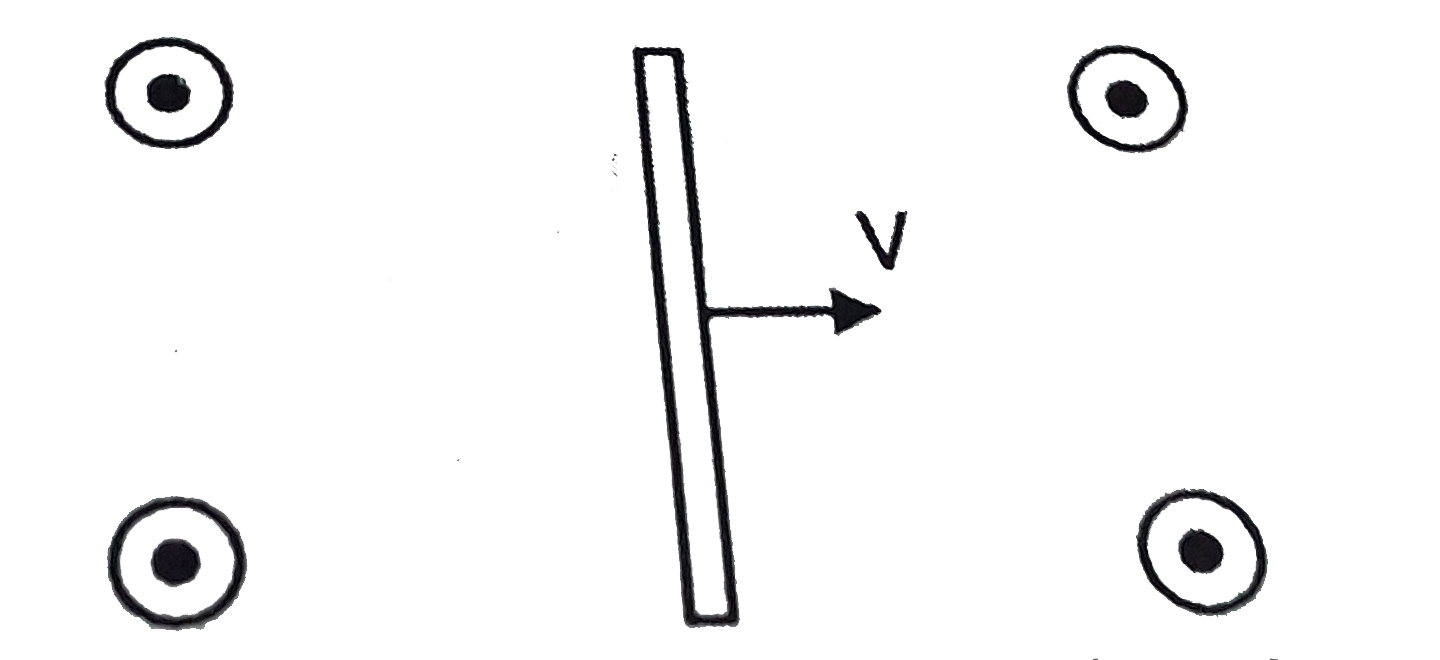
A metal rod moves at a constant velocity in a direction perpendicular to its length. A constant, uniform magnetic field exists in space in a direction perpendicular to the rod as well as its velocity. Select the correct statements(s) from the following
The entire rod is at same electric potential
There is an electric field in the rod
The electric potential is highest at the center of the rod and decreases towards its ends
The electric potential is lowest at the center of the rod and increases towards its ends
The correct Answer is:B
To solve the problem, we need to analyze the scenario step by step.
1. Understanding the Setup:
- We have a metal rod of length l moving with a constant velocity v.
- The direction of the rod is perpendicular to its velocity.
- A uniform magnetic field →B is also present, perpendicular to both the rod and its velocity.
2. Identifying Charge Movement:
- Since the rod is made of metal, it contains free electrons that can move.
- As the rod moves through the magnetic field, the electrons experience a magnetic force due to their motion in the field.
3. Applying the Lorentz Force:
- The magnetic force →F on a charge q moving with velocity →v in a magnetic field →B is given by the equation:
→F=q(→v×→B)
- For electrons, the charge q is negative, which means the direction of the force will be opposite to that calculated for a positive charge.
4. Determining the Direction of the Force:
- Using the right-hand rule, we can determine the direction of →v×→B:
- Point your fingers in the direction of →v (the velocity of the rod).
- Curl your fingers in the direction of →B (the magnetic field).
- Your thumb will point in the direction of the force on a positive charge.
- Since electrons are negatively charged, they will move in the opposite direction of the thumb.
5. Charge Accumulation:
- As a result of the force, electrons will accumulate at one end of the rod, creating a negative charge at that end and a positive charge at the opposite end.
- This leads to a separation of charge, resulting in an electric field being established within the rod.
6. Electric Field Direction:
- The induced electric field →E will point from the positive charge (higher potential) to the negative charge (lower potential).
7. Potential Difference:
- Since there is a charge separation, the two ends of the rod will have different electric potentials.
- The end where electrons accumulate will have a lower potential, and the end where positive charges accumulate will have a higher potential.
8. Conclusion:
- Based on the analysis, we can conclude:
- There is an induced electric field in the rod.
- The rod does not have the same electric potential throughout; there is a potential difference between the ends.
Correct Statements:
- The induced electric field exists in the rod (correct).
- The entire rod is not at the same electric potential (incorrect).
- The electric potential is highest at one end and lowest at the other end (correct).
- We have a metal rod of length l moving with a constant velocity v.
- The direction of the rod is perpendicular to its velocity.
- A uniform magnetic field →B is also present, perpendicular to both the rod and its velocity.
- Since the rod is made of metal, it contains free electrons that can move.
- As the rod moves through the magnetic field, the electrons experience a magnetic force due to their motion in the field.
- The magnetic force →F on a charge q moving with velocity →v in a magnetic field →B is given by the equation:
→F=q(→v×→B)
- For electrons, the charge q is negative, which means the direction of the force will be opposite to that calculated for a positive charge.
- Using the right-hand rule, we can determine the direction of →v×→B:
- Point your fingers in the direction of →v (the velocity of the rod).
- Curl your fingers in the direction of →B (the magnetic field).
- Your thumb will point in the direction of the force on a positive charge.
- Since electrons are negatively charged, they will move in the opposite direction of the thumb.
- As a result of the force, electrons will accumulate at one end of the rod, creating a negative charge at that end and a positive charge at the opposite end.
- This leads to a separation of charge, resulting in an electric field being established within the rod.
- The induced electric field →E will point from the positive charge (higher potential) to the negative charge (lower potential).
- Since there is a charge separation, the two ends of the rod will have different electric potentials.
- The end where electrons accumulate will have a lower potential, and the end where positive charges accumulate will have a higher potential.
- Based on the analysis, we can conclude:
- There is an induced electric field in the rod.
- The rod does not have the same electric potential throughout; there is a potential difference between the ends.
- The induced electric field exists in the rod (correct).
- The entire rod is not at the same electric potential (incorrect).
- The electric potential is highest at one end and lowest at the other end (correct).
Similar Questions
A conducting rod AB of length l=1m is moving at a velocity vA=4m/s making an angle 30∘ with its length. A uniform magnetic field B=2T exists in a direction perpendicular to the plane of motion. Then
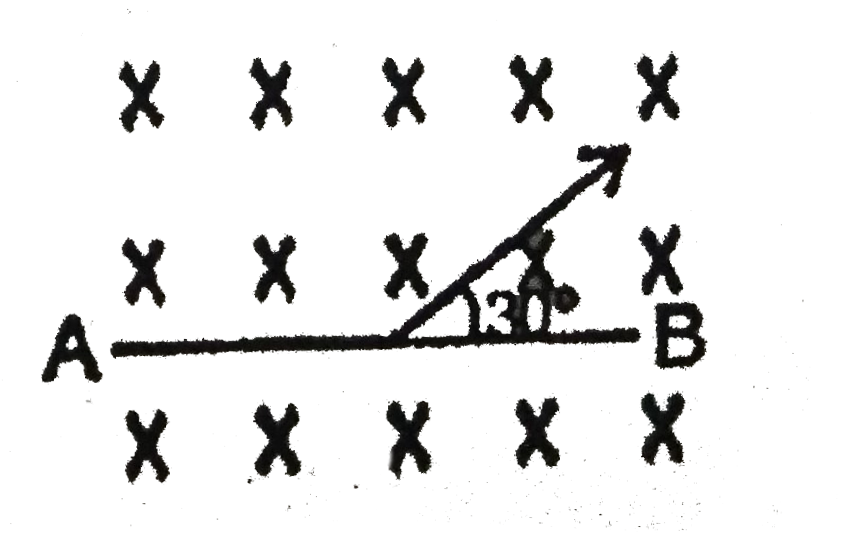
View SolutionA proton and an electron are projected into a region of uniform magnetic field in a direction perpendicular to the field. If they have the same initial velocities then
View SolutionA conducting loop rotates with constant angular velocity about its fixed diameter in a uniform magnetic field, whose direction is perpendicular to that fixed diameter.
View SolutionA conducting loop rotates with constant angular velocity about its fixed diameter in a uniform magnetic field, whose direction is perpendicular to that fixed diameter.
View SolutionA neutral metal bare moves at a constant velocity v to the right through a region of uniform magnetic field directed out the page, as shown. Therefore,
View SolutionA 20 cm long conducting rod is set into pure translation with a uniform velocity of 10cm s ^(-1) perpendicular to its length. A uniform magnetic field of magnitude 0.10 T exists in a direction perpendicular to the plane of motion. Find emf developed in the rod.
View SolutionA metallic metre sitck moves with a velocity of 2 ms ^(-1) in a direction perpendicular to its length and perpendicular to a uniform magnetic field of magnitude 0.2 T. Find the emf indcued between the ends of the stick.
View SolutionA rod of length l is translating at a velocity v making an angle θ with its length. A uniform magnetic field B exists in a direction perpendicular to the plane of motion. Calculate the emf induced In the rod, Draw a figure representing the induced emf by on equivalent battery.


View SolutionA conducting rod of unit length moves with a velocity of 5m/s in a direction perpendicular to its length and perpendicular to a uniform magnetic field of magnitude 0.4T. Find the emf induced between the ends of the stick.
View SolutionAssertion When a charged particle moves perpendicular to a uniform magnetic field then its momentum remains constant.
Reason Magnetic force acts perpendicular to the velocity of the particle.
View Solution
