In an L-C -R search circuit connected to an AC source , resistance
Choose the correct option
both (a) and (b) are correct
Both a and b are wrong
The correct Answer is:A
To solve the problem involving an L-C-R circuit connected to an AC source, we will follow these steps:
Step 1: Identify Given Values
We have the following values from the problem statement:
- Voltage across the resistor, VR=40V
- Voltage across the inductor, VL=40V
- Voltage across the capacitor, VC=10V
- Resistance, R=4Ω
- The voltage source is given by V=V0sin(100πt+π6)
Step 2: Calculate RMS Current
Using the formula for the voltage across the resistor:
VR=IRMS×R
Substituting the known values:
40=IRMS×4
Solving for IRMS:
IRMS=404=10A
Step 3: Calculate Inductive Reactance
Using the formula for the voltage across the inductor:
VL=IRMS×XL
Substituting the known values:
40=10×XL
Solving for XL:
XL=4010=4Ω
Step 4: Calculate Inductance L
From the relationship between inductive reactance and inductance:
XL=ωL
Where ω=100π. Therefore:
4=100πL
Solving for L:
L=4100π=125πH
Step 5: Calculate Capacitive Reactance
Using the formula for the voltage across the capacitor:
VC=IRMS×XC
Substituting the known values:
10=10×XC
Solving for XC:
XC=1010=1Ω
Step 6: Calculate Capacitance C
From the relationship between capacitive reactance and capacitance:
XC=1ωC
Substituting ω=100π:
1=1100πC
Solving for C:
C=1100πF
Conclusion
The values we calculated are:
- L=125πH
- C=1100πF
Final Answer
The correct option for the inductance L is 125πH, and for capacitance C is 1100πF.
---
We have the following values from the problem statement:
- Voltage across the resistor, VR=40V
- Voltage across the inductor, VL=40V
- Voltage across the capacitor, VC=10V
- Resistance, R=4Ω
- The voltage source is given by V=V0sin(100πt+π6)
Using the formula for the voltage across the resistor:
VR=IRMS×R
Substituting the known values:
40=IRMS×4
Solving for IRMS:
IRMS=404=10A
Using the formula for the voltage across the inductor:
VL=IRMS×XL
Substituting the known values:
40=10×XL
Solving for XL:
XL=4010=4Ω
From the relationship between inductive reactance and inductance:
XL=ωL
Where ω=100π. Therefore:
4=100πL
Solving for L:
L=4100π=125πH
Using the formula for the voltage across the capacitor:
VC=IRMS×XC
Substituting the known values:
10=10×XC
Solving for XC:
XC=1010=1Ω
From the relationship between capacitive reactance and capacitance:
XC=1ωC
Substituting ω=100π:
1=1100πC
Solving for C:
C=1100πF
The values we calculated are:
- L=125πH
- C=1100πF
The correct option for the inductance L is 125πH, and for capacitance C is 1100πF.
Similar Questions
In an L-C -R series circuit connected to an AC source V=V0sin(100π(t)+π6)VR=40V,VL=40andVC=10V, resistance R=4Ω
peak value of current is
View SolutionResistor, inductor and capacitor are connected in parallel to an AC source of emf V=100sinωt If R=2Ω,XL=2ΩandXC=4Ω,, then choose correct option:

View Solution
Knowledge Check
In a series L-R circuit (L=35mHandR=11Ω), a variable emf source (V=V0sinωt) of Vrms=220V and frequency 50 Hz is applied. Find the current amplitude in the circuit and phase of current with respect to voltage. Draw current-time graph on given graph (π=227).
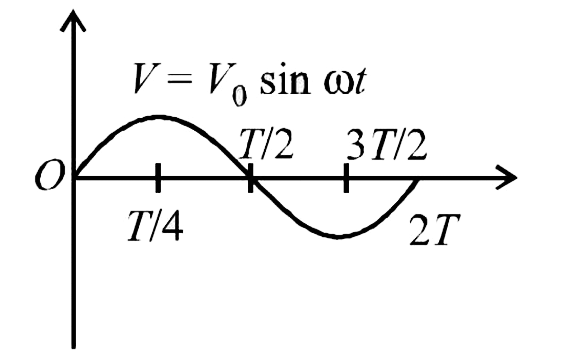 A10AB20 AC30 AD40 A
A10AB20 AC30 AD40 A
In an LCR series ac circuit the voltage across L,C and R are V1,V2 and V3 respectively The voltage of the source is .
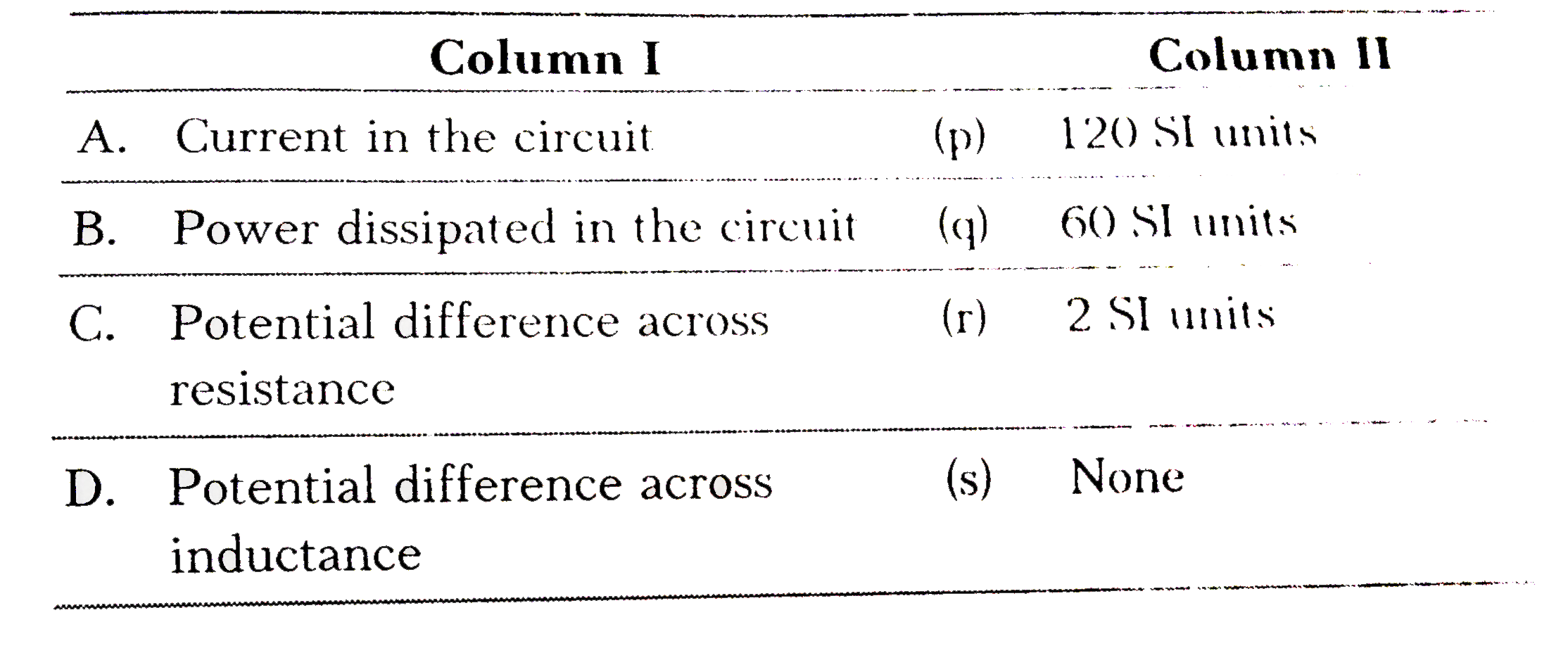
View SolutionIn an AC series L−C−R circuit, applied voltage is
V=(100√2sin(ωt+45∘))V
Given that, R=30Ω, XL=50Ω and XC=10Ω Now match the following two columns.
View SolutionA series L-C-R circuit is connected across an AC source V = 10sin[100πt−π6]. Current from the supply is I = 2sin[100πt+π12], What is the average power dissipated?
View SolutionA series L-C-R circuit is connected across a variable frequency source of emf. VL,VC,VR are instantaneous voltage drops across inductor capacitor and resistor and V is instantaneous voltage across the circuit.
Column I Column II(A)|V|(p)Can be greater than zero(B)|V|−|VL|(q)Can be less than zero(C)|V|−|VC|(r)Can be equal to zero(D)|V|−|VR|
View SolutionIn a series LCR circuit, the the rms voltage supply is 170 V. If VR,VLandVC represent the rms voltage drop across resistor , inductor and capacitor, then
View SolutionConsider a series LCR circuit connected to an AC supply of 220V. If voltage drop across resistance R is VR, voltage drop across capacitor is Vc=2VR and that across inudctor coil is VL=2VR then choose correct alternative(s)
View SolutionIn a series RC circuit with an AC source, R=300Ω,C=25μF,ε0=50V and v=50πHz. Find the peak current and the average power dissipated in the circuit.
View Solution
