A block of mass 1kg is pushed against a rough vertical wall with a force of 20N, coefficient of static friction being 14 Another horizontal force of 10N is applied on the block in a direction parallel to the wall. Will the block move? If yes, in which direction ? If no, find the frictional force exerted by the wall on the block. (g=10m/s2)
To solve the problem, we will analyze the forces acting on the block and determine whether it will move or not.
1. Identify the Forces Acting on the Block:
- The block has a mass of 1kg.
- The weight of the block (mg) acting downwards is:
mg=1kg×10m/s2=10N
- A force of 20N is applied perpendicular to the wall, pushing the block against the wall.
- A horizontal force of 10N is applied parallel to the wall.
2. Calculate the Normal Force:
- The normal force (N) exerted by the wall on the block is equal to the applied force pushing the block against the wall:
N=20N
3. Calculate the Maximum Static Friction:
- The coefficient of static friction (μs) is given as 14.
- The maximum static friction (fs) can be calculated as:
fs=μs×N=14×20N=5N
4. Determine the Forces Acting Horizontally:
- The horizontal force applied on the block is 10N.
- The maximum static friction opposing this force is 5N.
5. Analyze the Net Force:
- The net force acting on the block in the horizontal direction is:
Fnet=Applied Force−Frictional Force=10N−5N=5N
- Since the net force is positive, it indicates that the block will move.
6. Direction of Motion:
- The block will move in the direction of the applied horizontal force, which is parallel to the wall.
7. Conclusion:
- Yes, the block will move in the direction of the applied horizontal force (to the right).
- The frictional force exerted by the wall on the block is 5N.
Final Answer:
- The block will move to the right.
- The frictional force exerted by the wall on the block is 5N.
- The block has a mass of 1kg.
- The weight of the block (mg) acting downwards is:
mg=1kg×10m/s2=10N
- A force of 20N is applied perpendicular to the wall, pushing the block against the wall.
- A horizontal force of 10N is applied parallel to the wall.
- The normal force (N) exerted by the wall on the block is equal to the applied force pushing the block against the wall:
N=20N
- The coefficient of static friction (μs) is given as 14.
- The maximum static friction (fs) can be calculated as:
fs=μs×N=14×20N=5N
- The horizontal force applied on the block is 10N.
- The maximum static friction opposing this force is 5N.
- The net force acting on the block in the horizontal direction is:
Fnet=Applied Force−Frictional Force=10N−5N=5N
- Since the net force is positive, it indicates that the block will move.
- The block will move in the direction of the applied horizontal force, which is parallel to the wall.
- Yes, the block will move in the direction of the applied horizontal force (to the right).
- The frictional force exerted by the wall on the block is 5N.
- The block will move to the right.
- The frictional force exerted by the wall on the block is 5N.
Similar Questions
A block of mass 2 kg is pushed against a rough vertical wall with a force of 40N. Coefficient of static friction being 0.5. Another horizontal force of 15 N, is applied on the block in a direction parallel to the wall. Will the block move? If yes in which direction? If no, find the frictional force exerted by the wall on the block.
View SolutionA block of mass 2 kg is pushed against a rough vertical wall with a force is 40 N. Coefficient of static friction being 0.5. Another horizontal force is 15 N, is applied on the block in a direction parallel to the wall. Will the block move? If yes in which direction? If no, find the frictional force exerted by the wall on the block.
View SolutionA block of mass 1 kg is held at rest against a rough vertical surface by pushing by a force F horizontally. The coefficient of friction is 0.5. When

View SolutionA block of mass 1 kg is pushed against a rough vertical wall of μ=0.1
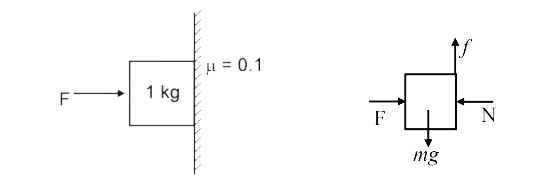
For F = 150, 50 or 100 N the force of friction on the blocks is respectively :
View SolutionA block of mass 2 kg is pressed against a rigid vertical wall by a horizontal force of 100 N. If co-efficient of static and kinetic friction are each equal to 0.3 then find the magnitude and direction of frictional force on the block.

View SolutionA block of mass 20kg is placed on a rough horizontal plane and a horizontal force of 12N is applied. If coefficient of friction is 0.1 the frictional force acting on it is,
View SolutionA block of 10kg is pulled at a constant speed on a rough horizontal plane by a force of 20N. Calculate the coefficient of friction ?
View SolutionA block of mass 20kg is pushed with a horizontal force of 90N. If the coefficient of static & kinetic friction are 0.4 & 0.3, the frictional force acting on the block is: (g=10ms−2)
View SolutionA block of mass 4 kg is kept on a rough horizontal surface. The coefficient of static friction is 0.8. If a forace of 19 N is applied on the block parallel to the floor, then the force of friction between the block and floor is
View SolutionA block of mass 2 kg is at rest on a floor . The coefficient of static friction between block and the floor is 0.54. A horizonatl force of 2.8 N is applied to the block . What should be the frictional force between the block and the floor ? ( take , g=10m/s2)
View Solution
