If both the resistance and the inductance in an LR AC series circuit are doubled the new impedance will be
halved
fourfold
doubled
quadrupted
The correct Answer is:C
To solve the problem, we need to determine the new impedance of an LR AC series circuit when both the resistance (R) and the inductance (L) are doubled.
1. Understand the Formula for Impedance:
The impedance (Z) in an LR series circuit is given by the formula:
Z=√R2+X2L
where XL (the inductive reactance) is given by:
XL=ωL
Here, ω is the angular frequency of the AC source.
2. Identify the New Values:
If both the resistance and the inductance are doubled, the new resistance (R′) and the new inductance (L′) can be expressed as:
R′=2R
L′=2L
Consequently, the new inductive reactance (X′L) will be:
X′L=ωL′=ω(2L)=2XL
3. Calculate the New Impedance:
Substitute the new values into the impedance formula:
Z′=√(R′)2+(X′L)2
This becomes:
Z′=√(2R)2+(2XL)2
Simplifying this:
Z′=√4R2+4X2L
Factor out the common term:
Z′=√4(R2+X2L)=2√R2+X2L
4. Relate New Impedance to Original Impedance:
Recognizing that the original impedance Z is:
Z=√R2+X2L
We can express the new impedance as:
Z′=2Z
5. Conclusion:
Therefore, the new impedance when both the resistance and the inductance are doubled is:
Z′=2Z
Final Answer:
The new impedance will be doubled.
The impedance (Z) in an LR series circuit is given by the formula:
Z=√R2+X2L
where XL (the inductive reactance) is given by:
XL=ωL
Here, ω is the angular frequency of the AC source.
If both the resistance and the inductance are doubled, the new resistance (R′) and the new inductance (L′) can be expressed as:
R′=2R
L′=2L
Consequently, the new inductive reactance (X′L) will be:
X′L=ωL′=ω(2L)=2XL
Substitute the new values into the impedance formula:
Z′=√(R′)2+(X′L)2
This becomes:
Z′=√(2R)2+(2XL)2
Simplifying this:
Z′=√4R2+4X2L
Factor out the common term:
Z′=√4(R2+X2L)=2√R2+X2L
Recognizing that the original impedance Z is:
Z=√R2+X2L
We can express the new impedance as:
Z′=2Z
Therefore, the new impedance when both the resistance and the inductance are doubled is:
Z′=2Z
The new impedance will be doubled.
Similar Questions
Knowledge Check
In the series LCR circuit shown the impedance is
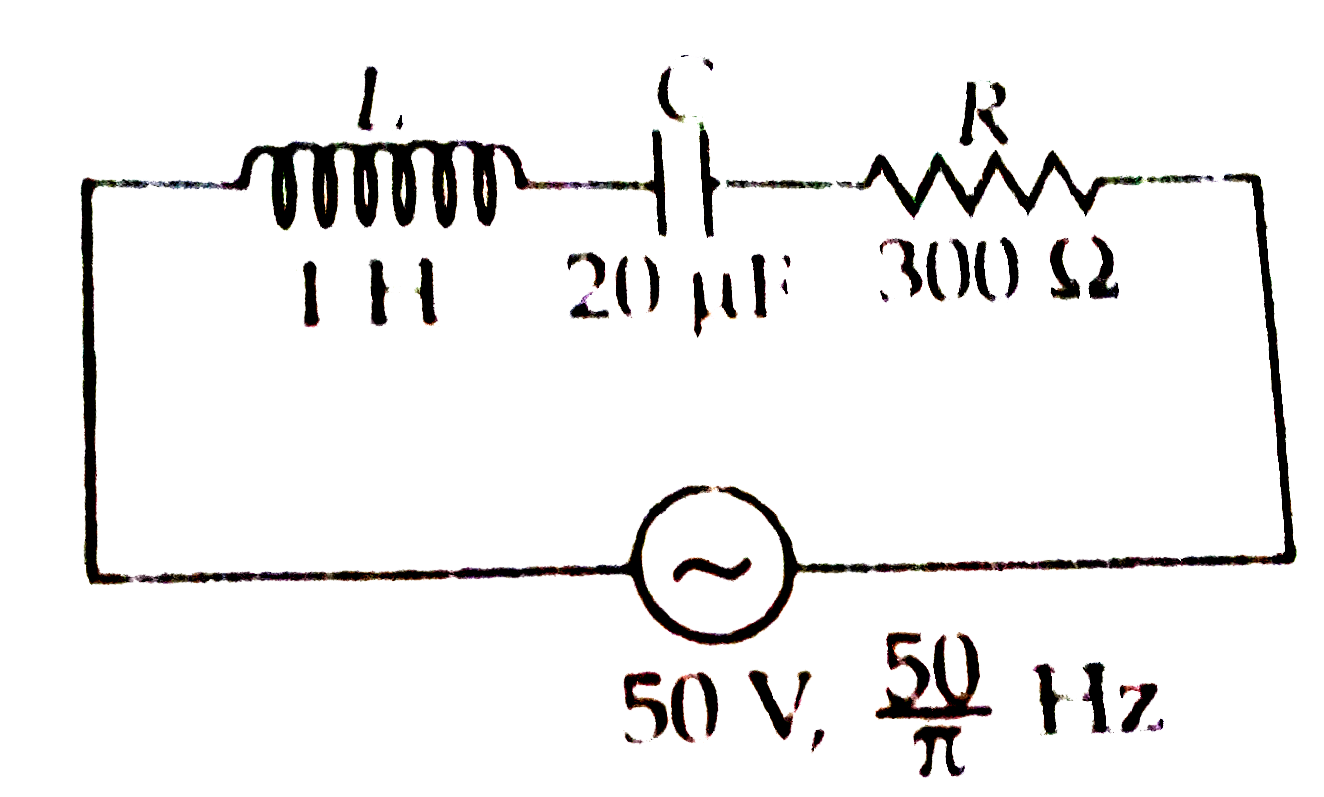 A200ΩB100ΩC300ΩD500Ω
A200ΩB100ΩC300ΩD500ΩAt resonance frequency the impedance in series LCR circuit is
AmaximumBminimumCzeroDinfinity
If an 8Ω resistance and 6Ω reactance are present in an AC series circuit then the impedence of the circuit will be
View SolutionIn an L-C-R series, AC circuit at resonance
View SolutionIf a 16Ωresistance and 12Ω inductive reactance are present in an a.c series circuit. Then the impedance of the circuit will be
View SolutionWhen the frequency of AC is doubled, the impedance of an LCR series circuit
View SolutionA resistance R draws power P when connected to an AC source. If an inductance is now placed in series with the resistance, such that the impedence of the circuit becomes Z, the power drawn will be
View SolutionBy what percentage the impedance in an AC series circuit should be increased so that the power factir changes from (1/2)to(1/4) (when R is constant)?
View Solution
