Two thin converging lenses are placed on a common axis, so that the centre of one of them coincides with the focus of the other. An object is placed at a distance twice the focal length from the left hand lens. Where will its image be? What is the lateral magnification? The focal of each lens is f.
To solve the problem step by step, we will analyze the situation with two converging lenses and apply the lens formula to find the image position and lateral magnification.
Step 1: Understand the Configuration
We have two thin converging lenses, L1 and L2. The center of L1 coincides with the focus of L2. The focal length of each lens is given as f. An object is placed at a distance of 2f from L1.
Step 2: Use the Lens Formula for L1
The lens formula is given by:
1f=1v−1u
Where:
- f = focal length of the lens
- v = image distance from the lens
- u = object distance from the lens (taken as negative in the lens formula)
For lens L1:
- u=−2f (since the object is placed at 2f)
- f=f
Substituting into the lens formula:
1f=1v−1−2f
This simplifies to:
1f=1v+12f
Rearranging gives:
1v=1f−12f=22f−12f=12f
Thus,
v=2f
Step 3: Determine the Image Position for L1
The image formed by L1 is at a distance of 2f on the opposite side of the lens.
Step 4: Use the Image from L1 as the Object for L2
The image formed by L1 acts as the object for L2. The distance of this image from L2 is:
- Since L1's image is at 2f and L2's focus coincides with L1's center, the object distance for L2 is:
u2=−f(since the image is at 2f and L2 is at f)
Step 5: Use the Lens Formula for L2
For lens L2, we again apply the lens formula:
1f=1v2−1u2
Substituting u2=−f:
1f=1v2−1−f
This simplifies to:
1f=1v2+1f
Rearranging gives:
1v2=0⇒v2=∞
This means that the image formed by L2 is at infinity.
Step 6: Calculate the Lateral Magnification
The magnification m for each lens is given by:
m=−vu
For L1:
m1=−v1u1=−2f−2f=−1
For L2:
m2=−v2u2=−∞−f=∞
However, since we are considering the overall magnification:
m=m1×m2=(−1)×(1)=−1
Final Result
The final image is at infinity and the overall lateral magnification is:
m=−12
We have two thin converging lenses, L1 and L2. The center of L1 coincides with the focus of L2. The focal length of each lens is given as f. An object is placed at a distance of 2f from L1.
The lens formula is given by:
1f=1v−1u
Where:
- f = focal length of the lens
- v = image distance from the lens
- u = object distance from the lens (taken as negative in the lens formula)
- u=−2f (since the object is placed at 2f)
- f=f
1f=1v−1−2f
This simplifies to:
1f=1v+12f
Rearranging gives:
1v=1f−12f=22f−12f=12f
Thus,
v=2f
The image formed by L1 is at a distance of 2f on the opposite side of the lens.
The image formed by L1 acts as the object for L2. The distance of this image from L2 is:
- Since L1's image is at 2f and L2's focus coincides with L1's center, the object distance for L2 is:
u2=−f(since the image is at 2f and L2 is at f)
For lens L2, we again apply the lens formula:
1f=1v2−1u2
Substituting u2=−f:
1f=1v2−1−f
This simplifies to:
1f=1v2+1f
Rearranging gives:
1v2=0⇒v2=∞
This means that the image formed by L2 is at infinity.
The magnification m for each lens is given by:
m=−vu
For L1:
m1=−v1u1=−2f−2f=−1
For L2:
m2=−v2u2=−∞−f=∞
However, since we are considering the overall magnification:
m=m1×m2=(−1)×(1)=−1
The final image is at infinity and the overall lateral magnification is:
m=−12
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.110videos
RAY OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.210videos
RAY OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY46videos
NUCLEI
- BOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective9videos
REFLECTION OF LIGHT
Similar Questions
Knowledge Check
- Question 1 - Select One
An object is placed at a distance of 1.5m from a screen and a convex lens is interposed between them. The magnification produced is 4. What is the focal length of the lens ?
A1mB0.5mC0.24mD2m
An object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
nature of the imageView SolutionAn object is placed in front of a converging lens at a distance greater than twice the focal length of the lens. Draw a ray diagram to show the formation of the image.
View SolutionWhen an object is at distances x and y from a lens, a real image and a virtual image is formed respectively having same magnification. The focal length of the lens is
View SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
the position of the imageView SolutionAn object is placed at a distance 24 cm in front of a convex lens of focal length 8 cm.
Calculate the distance of the image from the lens.View SolutionAn object is placed at a distance u from an equiconvex lens such that the distannce between the object and its real image is minimum. The focal length of the lens is f. The value of u is
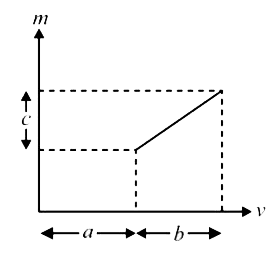
View SolutionThe graph shows how the magnification m produced by a thin lens varies with image distance v. What is the focal length of the lens used?
 View Solution
View Solution

