An object is placed in front of a converging lens at a distance greater than twice the focal length of the lens. Draw a ray diagram to show the formation of the image.
To solve the problem of drawing a ray diagram for an object placed in front of a converging lens (convex lens) at a distance greater than twice the focal length, we will follow these steps:
1. Identify the Components of the Lens:
- Draw a horizontal line to represent the principal axis.
- Mark the position of the convex lens at the center of the diagram.
- Label the focal points (F) on either side of the lens. The distance from the lens to the focal point is the focal length (f).
- Mark the center of curvature (C) which is located at a distance of 2f from the lens on both sides.
2. Position the Object:
- Place the object (represented by an arrow) at a distance greater than 2f on the left side of the lens. Label this distance as "Object".
3. Draw the Rays:
- First Ray: Draw a ray from the top of the object parallel to the principal axis. When this ray passes through the lens, it refracts and passes through the focal point (F) on the opposite side of the lens.
- Second Ray: Draw a ray from the top of the object that passes through the center of the lens. This ray will continue in a straight line without bending.
4. Locate the Image:
- Extend the refracted rays on the opposite side of the lens. The point where these two rays intersect is where the image is formed.
- Mark this intersection point as the "Image".
5. Characteristics of the Image:
- The image is located between F and 2F on the opposite side of the lens.
- The image is real (as it is formed by the actual intersection of rays), inverted (upside down compared to the object), and diminished (smaller than the object).
Final Diagram:
- Draw the complete ray diagram showing the object, the lens, the rays, and the image with appropriate labels.
- Draw a horizontal line to represent the principal axis.
- Mark the position of the convex lens at the center of the diagram.
- Label the focal points (F) on either side of the lens. The distance from the lens to the focal point is the focal length (f).
- Mark the center of curvature (C) which is located at a distance of 2f from the lens on both sides.
- Place the object (represented by an arrow) at a distance greater than 2f on the left side of the lens. Label this distance as "Object".
- First Ray: Draw a ray from the top of the object parallel to the principal axis. When this ray passes through the lens, it refracts and passes through the focal point (F) on the opposite side of the lens.
- Second Ray: Draw a ray from the top of the object that passes through the center of the lens. This ray will continue in a straight line without bending.
- Extend the refracted rays on the opposite side of the lens. The point where these two rays intersect is where the image is formed.
- Mark this intersection point as the "Image".
- The image is located between F and 2F on the opposite side of the lens.
- The image is real (as it is formed by the actual intersection of rays), inverted (upside down compared to the object), and diminished (smaller than the object).
- Draw the complete ray diagram showing the object, the lens, the rays, and the image with appropriate labels.
Topper's Solved these Questions
Similar Questions
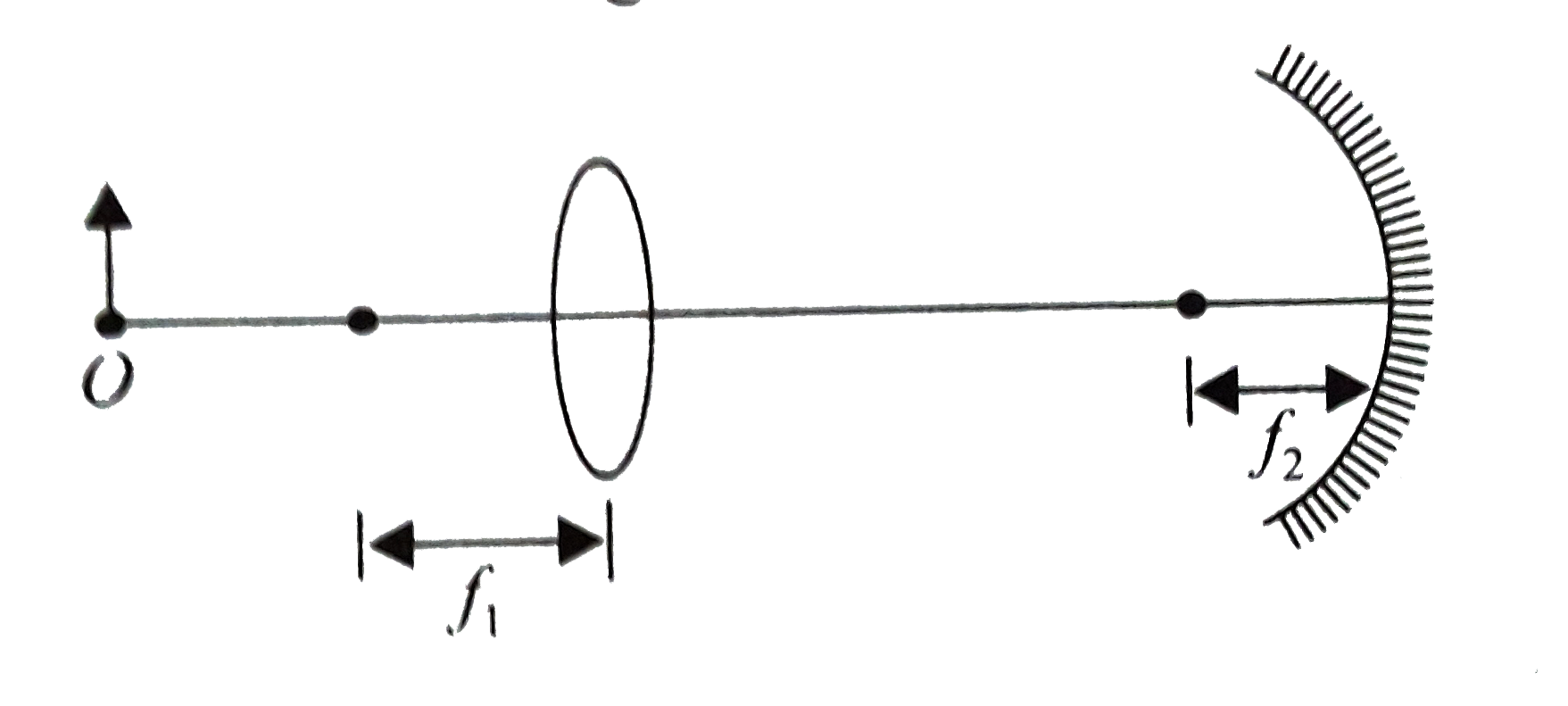
An object is placed in front of a converging lens at a distance equal to twice the focal length f1 of the lens. On the other side of the lens is a concave mirror of focal length f2 separated from the lens is a concave mirror of focal length f2 separated from the lens by a distance 2(f1+f2). Light from the object passes rightward through the lens, reflects from the mirror, passes leftward throught the lens, and forms a final image of the object.
View SolutionA lens produces a virtual image between the object and the lens.
Draw a ray diagram to show the formation of this image.
View SolutionA converging lens is used to obtain an image of an object placed in front of it. The inverted image is formed between F2and2F2 of the lens.
Draw a ray diagram to illustrate the formation of the image obtained.
View SolutionAn object is placed in front of a lens between its optical centre and the focus and forms a virtual, erect and diminished image.
Draw a ray diagram to show the formation of the image with the above stated characteristics.
View SolutionAn object is kept 40 cm in front of a concave mirror of focal length of 20 cm. Find the position and nature of the image. Draw a ray diagram to show the formation of image in this case.
View SolutionIt is desired to obtain an erect image of an object, using concave mirror of focal length of 12 cm.
(i) What should be the range of distance of an object placed in front of mirror ?
(ii) Will the image be smaller or large than the object ? Draw a ray diagram to show the formation of image in this case.
(iii) Where will the image of this object be, if it is placed 24 cm in front of the mirror ? Draw a ray diagram for this situation also to justify your answer.
Show the positions of the pole, the principal focus and the centre of curvature in the above ray diagrams.
View SolutionA virtual, diminished image is formed when an object is placed between the optical centre and the principal focus of a lens.
Draw a ray diagram to show the formation of the image with the above stated characteristics.
View SolutionA linear object is placed on the axis of a lens. An image is formed by refraction in the lens. For all positions of the object on the axis of the lens, the positions of the image are always between the lens and the object.
(i) Name the lens.
(ii) Draw a ray diagram to show the formation of the image of an object placed in front of the lens at any position of your choice except infinity.
View SolutionA convex lens of focal length 20cm is placed coaxilly with a convex mirror of radius of curvature 20 cm. The two are kept 15 cm apart. A point object is placed 40 cm in front of the convex lens. Find the position of the image formed by this combination. Draw the ray diagram showing the image formation.
View SolutionIf the image formed by a lens for all positions of an object placed in front of it is always erect and diminished, what is the nature of this lens ? Draw a ray diagram to justify your answer. If the numerical value of power of this lens is 10 D, what is its focal length in cartesian system ?
View Solution
