The equiconvex lens has focal length f. If is cut perpendicular to the principal axis passin through optical centre, then focal length of each half is
f
2f
The correct Answer is:D
To find the focal length of each half of an equiconvex lens when it is cut perpendicular to the principal axis passing through the optical center, we can follow these steps:
1. Understand the Lens Configuration:
- An equiconvex lens has two surfaces with the same radius of curvature (let's denote this radius as R).
- The lens has a focal length f.
2. Apply the Lens Maker's Formula:
- The lens maker's formula is given by:
1f=(μ−1)(1R1−1R2)
- For an equiconvex lens, we have:
- R1=+R (for the first surface)
- R2=−R (for the second surface)
3. Substituting Values into the Formula:
- Substituting R1 and R2 into the lens maker's formula:
1f=(μ−1)(1R−(−1R))
- This simplifies to:
1f=(μ−1)(2R)
4. Rearranging the Equation:
- Rearranging gives:
f=R2(μ−1)
5. Cutting the Lens:
- When the lens is cut perpendicular to the principal axis through the optical center, each half of the lens will still have one curved surface with radius R and the other surface will be flat (infinite radius of curvature).
- Thus, for each half:
- The new R1=+R (curved surface)
- The new R2=+∞ (flat surface)
6. Applying the Lens Maker's Formula Again:
- For each half, the lens maker's formula becomes:
1f′=(μ−1)(1R−0)
- This simplifies to:
1f′=μ−1R
7. Finding the Focal Length of Each Half:
- Rearranging gives:
f′=Rμ−1
8. Relating the New Focal Length to the Original:
- From the earlier equation f=R2(μ−1), we can see that:
f′=2f
Conclusion:
The focal length of each half of the lens after cutting is 2f.
- An equiconvex lens has two surfaces with the same radius of curvature (let's denote this radius as R).
- The lens has a focal length f.
- The lens maker's formula is given by:
1f=(μ−1)(1R1−1R2)
- For an equiconvex lens, we have:
- R1=+R (for the first surface)
- R2=−R (for the second surface)
- Substituting R1 and R2 into the lens maker's formula:
1f=(μ−1)(1R−(−1R))
- This simplifies to:
1f=(μ−1)(2R)
- Rearranging gives:
f=R2(μ−1)
- When the lens is cut perpendicular to the principal axis through the optical center, each half of the lens will still have one curved surface with radius R and the other surface will be flat (infinite radius of curvature).
- Thus, for each half:
- The new R1=+R (curved surface)
- The new R2=+∞ (flat surface)
- For each half, the lens maker's formula becomes:
1f′=(μ−1)(1R−0)
- This simplifies to:
1f′=μ−1R
- Rearranging gives:
f′=Rμ−1
- From the earlier equation f=R2(μ−1), we can see that:
f′=2f
The focal length of each half of the lens after cutting is 2f.
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - medical entrance special format question
23 Videos
- NUCLEIBOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY
46 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
Similar Questions
If a equiconvex lens of focal length f is cut into two halves by a plane perpendicular to the principal axis, then
View SolutionFind the focal length of the lens used.
View SolutionAn equiconvex lens of glass of focal length 0.1 metre is cut along a plane perpendicular to principle axis into two equal parts. The ratio of focal length of new lenses formed is:
View SolutionAn equiconvex lens of focal length20 cm is cut into two equal halves perpendicular to the principal axis and kept at a separation of 10 cm co-axially. What ils the focal length of the each part is?
View SolutionTwo thin equiconvex lenses each of focal length 0.2 m are placed coaxially with their optic centres 0.5m apart. Then find the focal length of the combination.
View SolutionA convex lens of focal length f cut into parts first horizontally and then vertically. Find the focal length of part A of the lens, as shown:
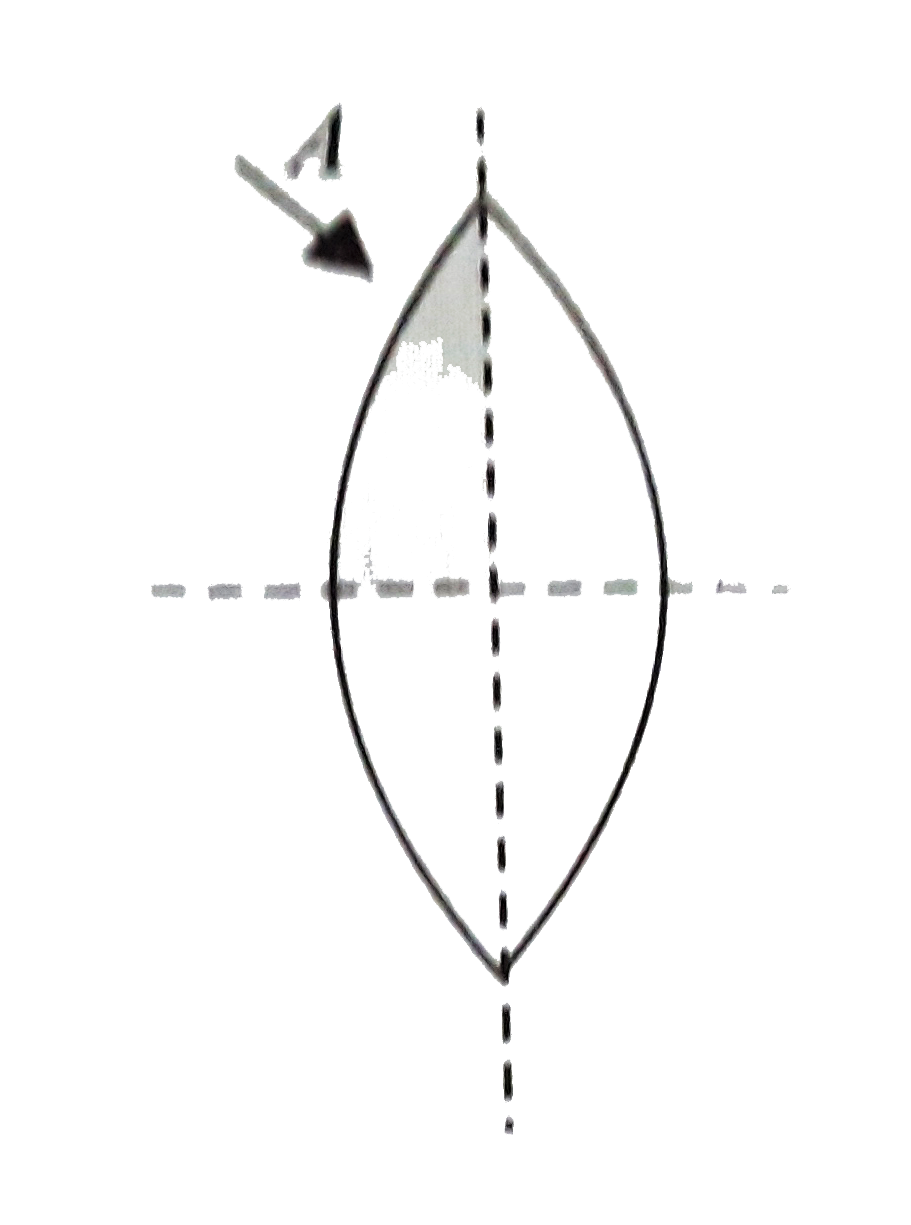
View SolutionAn object of length 2.0 cm is placed perpendicular to the principal axis of a convex lens of focal length 12 cm. Find the size of the image of the object is at a distance of 8.0 cm from the lens.
View SolutionAn equiconvex lens of focal length 15cm is cut into two equal halves in thickness. What is the focal length of each half ?
View SolutionOne half of a convex lens of focal length 10 cm is covered with a black paper. Can such
a lens produce an image of a complete object placed at a distance of 30 cm from the
lens? Draw a ray diagram to justify your answer. A 4 can tall object is placed
perpendicular to the perpendicular to the principal axis of a convex lens of focal length 20 cm. The distince
of the object from the less is 15 cm. Find nature, position and size of the image.
View SolutionA convex lens of focal length f1 is kept in contact with a concave lens of focal length f2. Find the focal length of the combination.
View Solution
