On the axis of a spherical mirror of focal length f a short linear object of length L lies on the axis at a distance μ from the mirror. Its image has an axial length L' equal to
L[fu−f]1/2
L[u+ff]1/2
L[u−ff]2
L[fu−f]2
The correct Answer is:D
To find the axial length L′ of the image formed by a spherical mirror when a short linear object of length L is placed at a distance μ from the mirror, we can follow these steps:
Step 1: Understand the setup
We have a spherical mirror with focal length f and a linear object of length L placed on the axis at a distance μ from the mirror. The object can be represented as having two ends, which we will denote as point 1 and point 2.
Step 2: Determine the object distances
For the two ends of the object:
- The distance of point 1 from the mirror is u1=μ−L2
- The distance of point 2 from the mirror is u2=μ+L2
Step 3: Use the mirror formula to find image distances
The mirror formula is given by:
1f=1v+1u
Where v is the image distance and u is the object distance.
For point 1:
1f=1v1+1u1
Rearranging gives:
v1=fu1u1−f
For point 2:
1f=1v2+1u2
Rearranging gives:
v2=fu2u2−f
Step 4: Calculate the image distances
Substituting u1 and u2:
1. For point 1:
v1=f(μ−L2)μ−L2−f
2. For point 2:
v2=f(μ+L2)μ+L2−f
Step 5: Find the axial length of the image
The axial length L′ of the image is given by the difference in the image distances:
L′=v2−v1
Step 6: Substitute the values of v1 and v2
Substituting the expressions for v1 and v2:
L′=f(μ+L2)μ+L2−f−f(μ−L2)μ−L2−f
Step 7: Simplify the expression
To simplify L′:
1. Find a common denominator.
2. Combine the fractions.
3. Factor out common terms.
After simplification, we find:
L′=f2L(μ−f)2
Final Result
The axial length of the image L′ is given by:
L′=Lf2(μ−f)2
We have a spherical mirror with focal length f and a linear object of length L placed on the axis at a distance μ from the mirror. The object can be represented as having two ends, which we will denote as point 1 and point 2.
For the two ends of the object:
- The distance of point 1 from the mirror is u1=μ−L2
- The distance of point 2 from the mirror is u2=μ+L2
The mirror formula is given by:
1f=1v+1u
Where v is the image distance and u is the object distance.
1f=1v1+1u1
Rearranging gives:
v1=fu1u1−f
1f=1v2+1u2
Rearranging gives:
v2=fu2u2−f
Substituting u1 and u2:
1. For point 1:
v1=f(μ−L2)μ−L2−f
v2=f(μ+L2)μ+L2−f
The axial length L′ of the image is given by the difference in the image distances:
L′=v2−v1
Substituting the expressions for v1 and v2:
L′=f(μ+L2)μ+L2−f−f(μ−L2)μ−L2−f
To simplify L′:
1. Find a common denominator.
2. Combine the fractions.
3. Factor out common terms.
L′=f2L(μ−f)2
The axial length of the image L′ is given by:
L′=Lf2(μ−f)2
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - medical entrance special format question
23 Videos
- NUCLEIBOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY
46 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
Similar Questions
An infinitely long rod lies along the axis of a concave mirror of focal length f. The near end of the rod is distance u>f from the mirror. Its image will have length
View SolutionA short linear object of length b lies along the axis of a concave mirror or focal length f at a distance u from the pole of the mirror. The size of the image is approximately equal to
View SolutionFor a concave mirror of focal length f, image is 2 times larger. Then the object distance from the mirror can be
View SolutionThe image formed by a convex mirror of focal length 30cm. is a quarter of the object. What is the distance of the object from the mirror ?
View SolutionA point object is moving on the principal axis of a concave mirror of focal length 24cm towards the mirror. When it is at a distance of 60cm from the mirror, its velocity is . 9sec/cm What is the velocity of the image at that instant
View SolutionA convex mirror offocal length fforms an image which is 1/n times the object. The distance of the object from the mirror is:
View SolutionA convex mirror of focal length f produced an image (1/n)th of the size of the object. The distance of the object from the mirror is
View SolutionA concave mirror of focal length f produces a real image n times the size of the object. What is the distance of the object from the mirror?
View SolutionThe radius of curvature of a spherical mirror is 20 cm. What is its focal length?
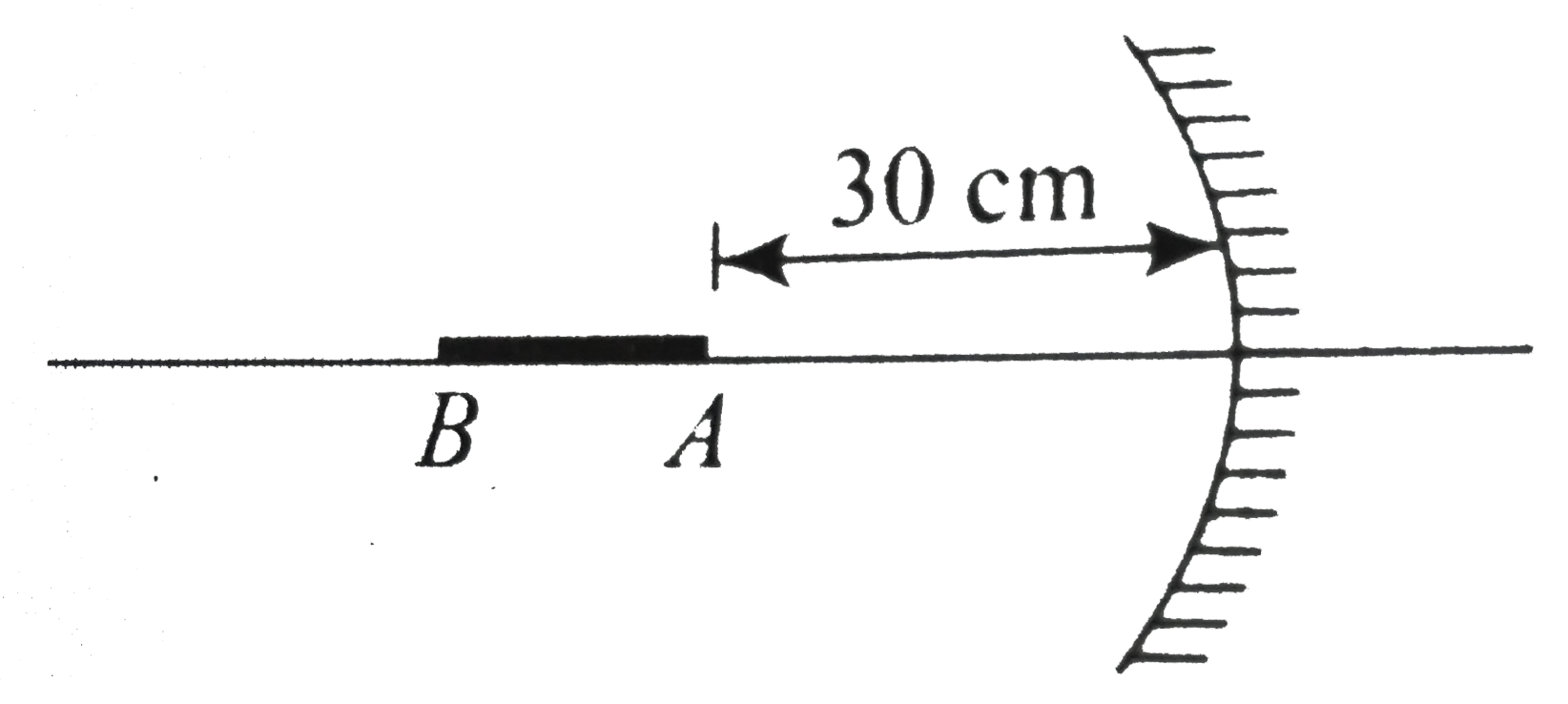
View SolutionObject AB is placed on the axis of a concave mirror of focal length 10cm. End A of the object is at 30cm from the mirror. Find the length of the image
a. if length of object is 5cm.
b. if length of object is 1 mm.
View Solution
