AU235 reactor generated power at a rate of P producting 2×1018 fussion per second. The energy released per fission is 185 MeV. The value of P is
59.2 MW
370×1018MW
0.59MW
370 MW
The correct Answer is:A
To solve the problem, we need to calculate the power P generated by a U-235 reactor based on the given data. Here’s a step-by-step solution:
Step 1: Identify the given values
- The number of fissions per second, n=2×1018 fissions/second.
- The energy released per fission, E=185 MeV.
Step 2: Convert energy from MeV to Joules
1. We know that 1 MeV = 1.6×10−13 Joules.
2. Therefore, to convert E from MeV to Joules:
E=185MeV×1.6×10−13J/MeV=185×1.6×10−13J
E=296×10−13J=2.96×10−11J
Step 3: Calculate the power P
1. The power generated by the reactor can be calculated using the formula:
P=n×E
where n is the number of fissions per second and E is the energy released per fission in Joules.
2. Substitute the values:
P=(2×1018fissions/second)×(2.96×10−11J)
P=5.92×107W
Step 4: Convert power from Watts to Megawatts
1. To convert Watts to Megawatts, we divide by 106:
P=5.92×107W106=59.2MW
Final Answer
The power P generated by the U-235 reactor is 59.2MW.
---
- The number of fissions per second, n=2×1018 fissions/second.
- The energy released per fission, E=185 MeV.
1. We know that 1 MeV = 1.6×10−13 Joules.
2. Therefore, to convert E from MeV to Joules:
E=185MeV×1.6×10−13J/MeV=185×1.6×10−13J
E=296×10−13J=2.96×10−11J
1. The power generated by the reactor can be calculated using the formula:
P=n×E
where n is the number of fissions per second and E is the energy released per fission in Joules.
2. Substitute the values:
P=(2×1018fissions/second)×(2.96×10−11J)
P=5.92×107W
1. To convert Watts to Megawatts, we divide by 106:
P=5.92×107W106=59.2MW
The power P generated by the U-235 reactor is 59.2MW.
Topper's Solved these Questions
Similar Questions
In a nuclear reactor, the number of U235 nuclei undergoing fissions per second is 4×1020. If the energy releases per fission is 250 MeV, then the total energy released in 10 h is (1eV=1.6×10−19J)
View SolutionA nuclear reactor has a power of 16 kW. If the energy released per fission is 200 MeV, the number of fissions per second is
View SolutionIn the given arrangement the block is released from the position where the spring is unstretched. The speed of the block when it has descended through 2cm is x10. Find the value of x.
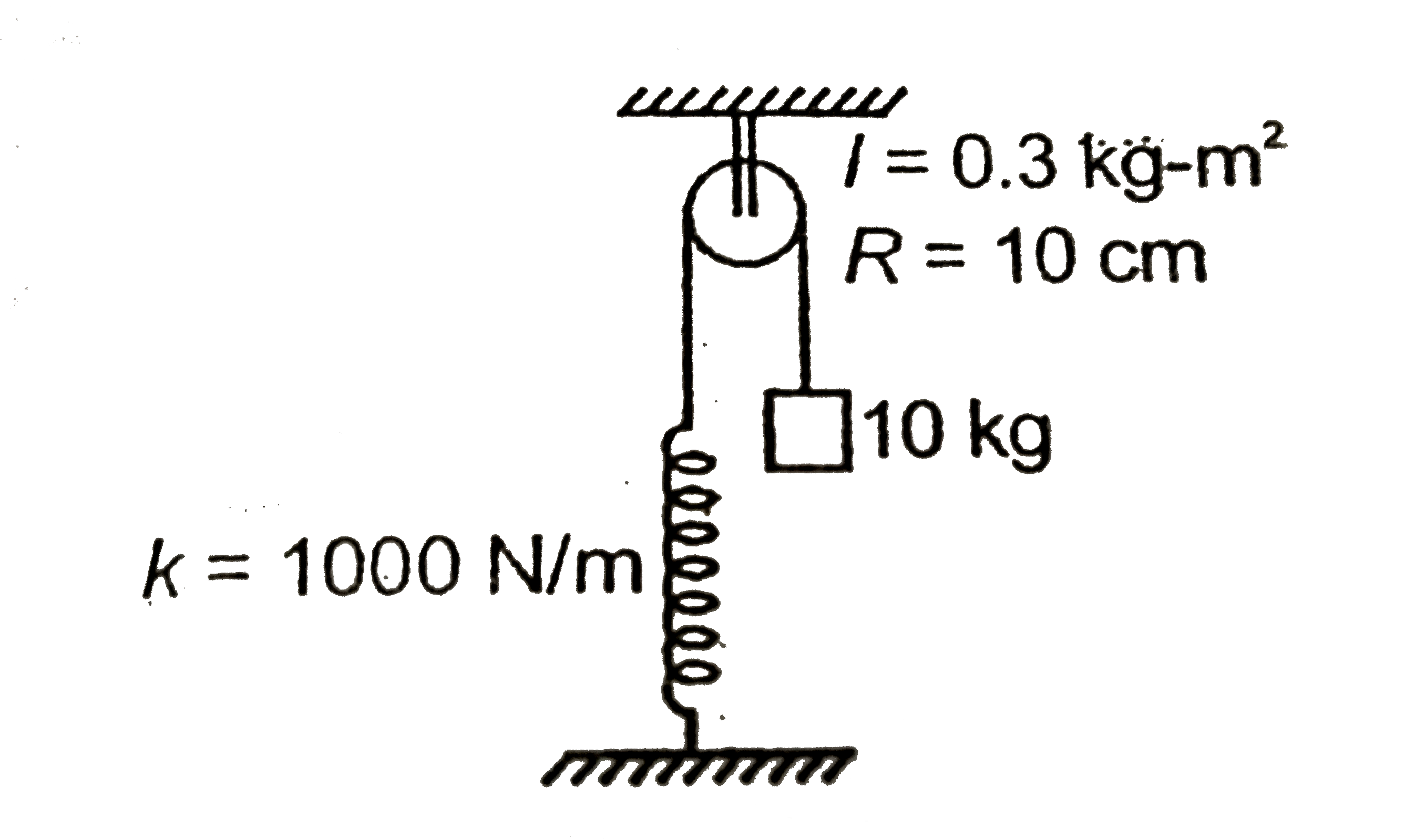
View SolutionTo generate a power of 3.2 mega watt, the number of fissions of U235 per minute is.
(Energy released per fission =200MeV,1eV=1.6×10−19J).
View SolutionCalculate the energy released by fission from 2 gm of .92U235 in KWH. Given that the energy released per fission is 200 Mev.
View SolutionCalculate the energy released by fission from 2 g of .23592U in k Wh. Given that the energy released per fission is 200 Mev.
View SolutionThe rate constant of a first order reaction is 3×10−6 per second. If the initial concentration is 0.10M, the initial rate of reaction is
View Solution1.00 kg of .235U undergoes fission process. If energy released per event is 200MeV, then the total energy released is
View SolutionA reactor is generating 1000 kW of power and 200 MeV of energy may be obtained per fission of U235 The rate of nuclear fission in the reactor is
View SolutionThe energy released during the fission of 1kg of U235 is a E1 and that product during the fusion of 1 kg of hydrogen is E2 . If energy released per fission of Uranium - 235 is 200 MeV and that per fusion of hydrogen is 24.7 MeV , then the ratio E2E1 is
View Solution
