The log - log graph between the energy E of an electron and its de - Broglie wavelength λ will be

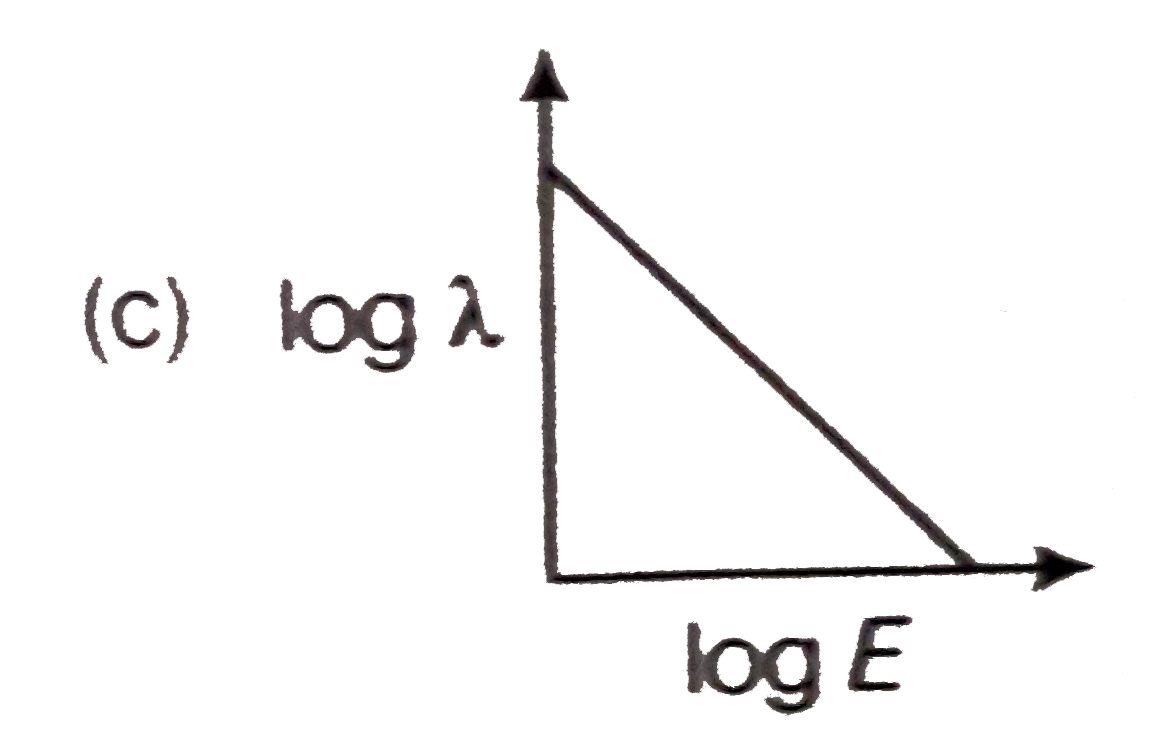
The correct Answer is:C
To solve the problem of determining the log-log graph between the energy E of an electron and its de Broglie wavelength λ, we can follow these steps:
1. Understand the de Broglie wavelength formula:
The de Broglie wavelength λ of a particle is given by the formula:
λ=h√2mE
where h is Planck's constant, m is the mass of the electron, and E is the energy of the electron.
2. Rearranging the formula:
We can rearrange the formula to express it in terms of E:
λ=h√2m⋅1√E
3. Taking logarithms:
To analyze the relationship in a log-log graph, we take the logarithm of both sides:
logλ=log(h√2m)−12logE
4. Breaking down the logarithm:
We can separate the logarithm on the right-hand side:
logλ=logh−12log(2m)−12logE
5. Identifying the linear relationship:
This equation can be rewritten as:
logλ=(−12)logE+(logh−12log(2m))
Here, we can identify:
- The slope of the graph is −12
- The y-intercept is logh−12log(2m)
6. Graph characteristics:
Since the slope is negative, the graph of logλ versus logE will be a straight line that slopes downwards.
Final Result:
The log-log graph between the energy E of an electron and its de Broglie wavelength λ will be a straight line with a negative slope of −12.
The de Broglie wavelength λ of a particle is given by the formula:
λ=h√2mE
where h is Planck's constant, m is the mass of the electron, and E is the energy of the electron.
We can rearrange the formula to express it in terms of E:
λ=h√2m⋅1√E
To analyze the relationship in a log-log graph, we take the logarithm of both sides:
logλ=log(h√2m)−12logE
We can separate the logarithm on the right-hand side:
logλ=logh−12log(2m)−12logE
This equation can be rewritten as:
logλ=(−12)logE+(logh−12log(2m))
Here, we can identify:
- The slope of the graph is −12
- The y-intercept is logh−12log(2m)
Since the slope is negative, the graph of logλ versus logE will be a straight line that slopes downwards.
The log-log graph between the energy E of an electron and its de Broglie wavelength λ will be a straight line with a negative slope of −12.
Topper's Solved these Questions
- SOLVED PAPERS 2018BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPERS 2018EXERCISE - Assertion and Reasons
10 Videos
- SOLVED PAPERS 2018BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER
22 Videos
- SOLVED PAPERS 2018BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER
22 Videos
- SOLVED PAPER 2017BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPER 2017EXERCISE - Solved papers 2017(JIPMER)
32 Videos
- WAVE OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced E. Integer Type Questions
13 Videos
Similar Questions
Knowledge Check
When the velocity of an electron increases, its de Broglie wavelength
AincreasesBdecreasesCremains sameDmay increase or decreaseIn an excited state of hydrogen like atom an electron has total energy of −3.4eV. If the kinetic energy of the electron is E and its de-Broglie wavelength is λ, then
AE=6.8eV,λ=6.6×10−10mBE=3.4eV,λ=6.6×10−10mCE=3.4eV,λ=6.6×10−11mDE=6.8eV,λ=6.6×10−11mIf a proton and electron have the same de Broglie wavelength, then
Akinetic energy of electron < kinetic energy of protonBkinetic energy of electron = kinetic energy of protonCmomentum of electron = momentum of protonDmomentum of electron < momentum of proton
If the kinetic energy of a free electron doubles , its de - Broglie wavelength changes by the factor
View SolutionIf the velocity of an electron is doubled, its de-Broglie frequency will be
View SolutionIf the kinetic energy of a moving particle is E , then the de-Broglie wavelength is
View SolutionThe kinetic energy (K.E.) of a beam of electrons, accelerated through a potential V, equalws the energy of a photon of wavelength of 5460 nm. Find the de-Broglie wavelength associated with this beam of electrons.
View SolutionThe energy that should be added to an electron to reduce its de - Broglie wavelength from one nm→0.5nm is
View Solution
