Let ABC be a triangle with integer sides a,b,c and ∠C=90∘
The number of triangles with r=2009 is
A
10
B
12
C
13
D
15
Text Solution
The correct Answer is:D
|
Topper's Solved these Questions
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - LECTURE SHEET EXERCISE - III (Straight Objective Type Question)
62 Videos
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - LECTURE SHEET EXERCISE - IV (Straight Objective Type Question)
38 Videos
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - LECTURE SHEET EXERCISE - II(Matrix Matching Type Questions)
5 Videos
- PLANESBOOK - AAKASH SERIESCHAPTER - PLANESEXERCISE - ADVANCED SUBJECTIVE TYPE QUESTIONS
30 Videos
- PROPERTIES OF VECTORSBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF VECTORSEXERCISE - PRACTICE EXERCISES
55 Videos
Similar Questions
Knowledge Check
If angle C of triangle ABC is 900, then prove that tanA+tanB=c2ab (where, a,b,c, are sides opposite to angles A,B,C, respectively).
Aa2bcBb2caCc2abDa+bIn ΔABC, if ∠A=60∘,∠B=50∘,and∠C=70∘, then find the longest side of the triangle ABC.
ABCBABCACDNone of thesePedal Triangle of a Triangle: Let ΔABC be any triangle and let D, E, F be the feet of perpendiculars from the vertices A, B, C on the opposite sides BC, CA, AB respectively, then the triangle DEF is known as Pedal triangle of ABC. H is the orthocentre of the ΔABC. We note that ∠HDC=∠HEC=90∘'
∴∠HDE=∠HCE=90∘−A.
Simillarly ∠HDF=∠HBF=90∘−A.
Hence, ∠FDE=180∘−2A.
Identically we can find ∠DEF=180∘−2B
and ∠EFD=180∘−2C.
Thus, the angles of Pedal triangle are 180∘−2A,180∘−2B,180∘−2C. Further in ΔBFD,∠FDB=90∘−(90∘−A)=A, thus FBsinB=FBsinA
∴FD=sinBsinA(BCcosB)=asinBcosBsinA=2RsinBcosB=bcosB.
Similarly, EF=acosAandDE=ccosC.
Thus, the sides of pedal triangle are acosA,bcosBandccosC(orRsin2A,Rsin2B,Rsin2C), where R is the circumradius of ΔABC.
In the above derivation, the triangle ABC is assumed an acute angled triangle. In case the triangle ABC be obtuse angled with A as obtuse angle, then, the angles of pedal triangle will be represented by 2A−180∘,2B,2Cand the sides will be represented by −acosA,bcosB,ccosC.
The inradius of the pedal triangle of a tringle ABC isAR/2BRsinA2sinB2sinC2C2RcosAcosBcosCD4RsinA2sinB2sinC2
Assertion : Δ ABC is an acute angled triangle, ∠A,∠Band∠C are less than 90∘
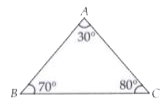
Reason : Triangles are classified according to the sides and the angles.
View SolutionIf in a ΔABC,b=√3,c=1andB−C=90∘, then ∠A is
View SolutionIf in a ΔABC,b=√3,c=1andB−C=90∘, then ∠A is
View SolutionIn a ΔABC∠C=60∘∠B=90∘ find the ratio of sides
View SolutionGive a right angle triangle ABC with right angled at C. ∠C=90∘ and a,b,c are lengths of corresponding sides. (b>a).
cos(A−B) will be equal to
View Solution