In triangle ABC, base BC and area of triangle D are fixed. Locus of the centroid of triangle ABC is a striaght line is
parallel to side BC
right bisector of side BC
right angle of BC
inclined at an angle sin−1(√ΔBC) to side BC
The correct Answer is:A
Topper's Solved these Questions
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - PRACTICE SHEET (EXERCISE-III) (LEVEL-II) (More than one correct answer Type Questions)
5 Videos
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - PRACTICE SHEET (EXERCISE-III) (LEVEL-II) (Linked Comprehension Type Questions)
9 Videos
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - PRACTICE SHEET (EXERCISE-III) (LEVEL-I) (Straight Objective Type Questions)
42 Videos
- PLANESBOOK - AAKASH SERIESCHAPTER - PLANESEXERCISE - ADVANCED SUBJECTIVE TYPE QUESTIONS
30 Videos
- PROPERTIES OF VECTORSBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF VECTORSEXERCISE - PRACTICE EXERCISES
55 Videos
Similar Questions
In triangle ABC , base BC and area of triangle are fixed. The locus of the centroid of triangle ABC is a straight line that is a) parallel to side BC (b)right bisector of side BC (c)perpendicular to BC (d)inclined at an angle sin−1(√BC) to side BC
View SolutionIn triangle ABC , base BC and area of triangle are fixed. The locus of the centroid of triangle ABC is a straight line that is a) parallel to side BC (b)right bisector of side BC (c)perpendicular to BC (d)inclined at an angle sin−1(√BC) to side BC
View Solution
Knowledge Check
Two of the vertices of a triangle ABC and A (1,1) B-1,3) and the area of ΔABC is 6 sq units. If P is the centroid of the ΔABC, then find the locus of P.
A2x−y+1=0B2x−y−3=0C2x+y+3=0DBoth (a) and (b)If D, E and F are the mid-points of sides BC, CA and AB respectively of △ABC, then the ratio of the areas of △DEF to the area of △ABC is :
A1:4B1:2C1:3D2:3The triangle is formed by joining the mid-points of the sides AB,BC and CA of △ABCand the area of △PQR is 6cm2, then the area of △ABC is :
A36cm2B12cm2C18cm2D24cm2
In Fig. 9.29 ratio of the area of triangle ABC to the area of triangle ACD is the same as the ratio of base BC of triangle ABC to the base CD of triangle ACD.
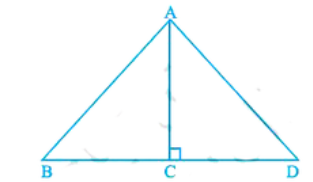
View SolutionThe centroid of a triangle ABC is G. The area of triangle ABC is 60 cm2. The area of triangle GBC is.
View SolutionIn the figure given below, ABC is a triangle with AB = BC and D is an interior point of the triangle ABC such that ∠DAC=∠DCA.
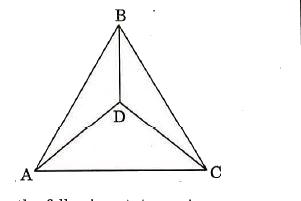
Consider the following statements :
1. Triangle ADC is an isosceles triangle.
2. Dis the centroid of the triangle ABC.
3. Triangle ABD is congruent to the triangle CBD.
Which of the above statements are correct ?
View SolutionG is the centroid of the triangle ABC. Prove that the area of the triangle ABG is one third of the area of the triangle ABC.
View Solution. If G is the centroid of ΔABC and AG−BC, then ∠BGC is :
View Solution
