Two wires of same radius and legth are subjected to the same load . One wire is of steel an the other is of copper . If the young modulus of steel is twice that of copper find the ratio of elastic energy stored per unit volume in steel to that of copper .Solution in Assamese
Topper's Solved these Questions
- BOOK - BINA LIBRARYCHAPTER - PROPERTIES OF SOLID EXERCISE - EXERCISE65videos
PROPERTIES OF SOLID
- BOOK - BINA LIBRARYCHAPTER - PROPERTIES OF FLUIDSEXERCISE - EXERCISE109videos
PROPERTIES OF FLUIDS
- BOOK - BINA LIBRARYCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EXERCISE61videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
Similar Questions
What is inter - atomic force ?
05:05View SolutionWhat is sarface tension? What is its unit?
04:32View SolutionDefine torque. State its dimensions.
05:45View SolutionTwo wires of equal cross section but one made of steel and the other of copper, are joined end to end. When the combination is kept under tension, the elongations in the two wires are found to be equal. Find the ratio of the lengths of the two wires. Young modulus of steel =2.0×1011Nm−2 and that of copper =1.1×101Nm−2
02:08View SolutionA homogeneous block with a mass m hangs on three vertical wires of equal length arranged symmetrically . Find the tensions of the wires if the middle wire is of steel and the other two are of copper . All the wires have the same cross - section . Consider the modulus of elasticity of steel to be double than that of copper .
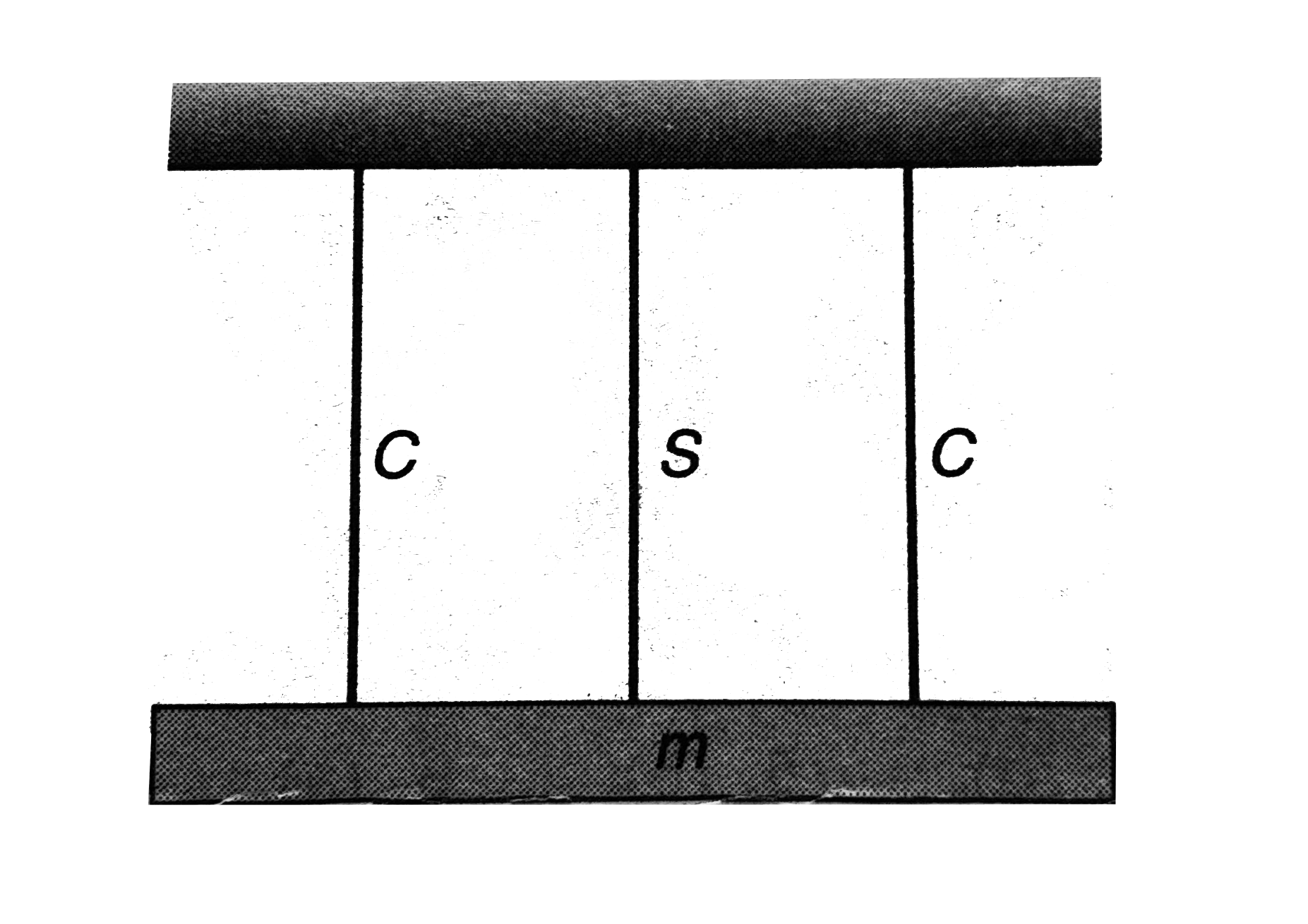 01:58View Solution
01:58View SolutionA piece of copper wire has twice the radius of a piece of steel wire. Young's modulus for steel is twice that of the copper. One end of the copper wire is joined to one end of the steel wire so that both can be subjected to the same longitudinal force. By what fraction of its length will the steel have stretched when the length of the copper has increased by 1%?
04:23View SolutionSteel and copper wires of same length are stretched by the same weight one after the other. Young's modulus of steel and copper are 2xx10^(11)(N)/(m^2) and 1.2xx10^(11)(N)/(m^2) . The ratio of increase in length is
07:09View SolutionTwo wire of same radius and lengh the are subjected to the same load. One wire is of steel and the other is of copper. If the Young's modulus of steel is twice that of copper, the ratio the energy stored per unit volume in steel to that of copper wire is
View SolutionTwo identical wires, one of copper and the other os steel are of the same length. They are equally stretched. If Ysteel>Ycopper then in stretching
05:20View SolutionTwo wires, out of copper and the other of steel, are of same length and cross section. They are welded together to from a long wire. On suspending a weight at its one end, increment in length is found to be 3 cms. If Young's modulus of steel is double that of copper the increment in steel wire will be
04:03View SolutionThe Young's Modulus of steel is twice that of brass. Two wires of same length and of same area of cross-section, one of steel and another of brass are suspended from the same roof. If then the weights added to the steel and brass wires must be in the ratio of
03:06View SolutionThe radius of a copper wire is twice that of a steel wire. One end of the copper wire is joined with one end of the steel wire and the same tensile force is applied on them . If the length of the copper wire increases by 1% , what will be the percentage increase in the length of the steel wire? Given Young's modulus of steel is twice that of copper.
04:55View SolutionTwo wires of equal cross section but one made of steel and the other of copper, are joined end to end. When the combination is kept under tension, the elongations in the two wires are found to be equal. Find the ratio of the lengths of the two wires. Young modulus of steel =2.0×1011Nm−2 and that of copper =1.1×1011Nm−2
04:24View SolutionTwo wires of same radius and length are subjected to the same load. One wire is of steel and the other is of copper. It the Young's modulus of steel is twice that of copper, the ratio of elastic energy stored per unit volume in steel to that of copper wire is
04:01View SolutionOne end of a steel wire of radius .r. is fixed to a ceiling and a load of 3 kg is attached to the free end of the wire. Another wire made of copper of radius .2r. is attached to the bottom of 3 kg load and a 2 kg load is attached to the free end of the copper wire. The ratio of longitudinal strains produced in copper and steel wires is
(Young modulus of steel =20×1010Nm−2)
(Young modulus of copper =12×1010Nm−2)05:25View Solution

