Several identical capacitors were connected in parallel and charged to a voltage φ0. Subsequently they were reconnected in series with the aid of a switch. What will be the voltage across the terminals? Will the energy of the system change?
The solution is obvious from Figs. 24.11a and 24.11b, which give the diagrams of the parallel and series connections of four identical capacitors. The energy in the case of parallel connection is
Wpar=CparΨ2par2=nCnφ202
and of series connections
Wser=Cserφ2ser2=(C0/n)n2φ202=Wpar
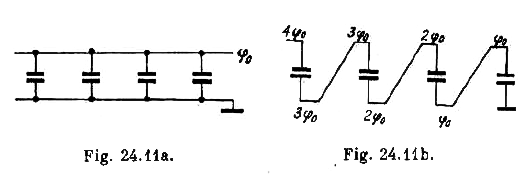
Similar Questions
A capacitor C is fully charged with voltage V0. After disconnecting the voltage source, it is connected in parallel with another uncharged capacitor of capacitance C2. The energy loss in the process after the charge is distributed between the two capacitors is :
View Solutionn identical capacitors are connected in parallel to a potential difference V. These capacitors are then reconnected in series, their charges being left undisturbed. The potential difference obtained is
View SolutionA 1μF capacitor and a 2µF capacitor are connected in series across a 1200V supply line.
a. Find the charge on each capacitor and the voltage across them.
b. The charged capacitors are disconnected from the line and from each other and reconnected with terminals of like sign together. Find the final charge on each and the voltage across them.
View SolutionThree capacitors having capacitances of 8.4 muF, 8.4 muF and 4.2 muF are connected in series across a 36 potential difference. (a) What is the charge on 4.2muF capacitor? (b) What is the total energy stored in all three capacitors? (c) The capacitors are disconnected from the potential difference without allowing them to discharge. They are then reconnected in parallel with each other, with the positively charged plates connected together. What is the voltage across each capacitor in the parallel combination? (d) What is the total energy now stored in the capacitors?
View SolutionA 2μF capacitor C1 is charge to a voltage 100 V and a 4μF capacitor C2 is charged to a voltage 50 V. The capacitors are then connected in parallel What is the loss of energy due to parallel connection?
View SolutionA capacitor (0.2F) is charged to 600V. After removing the charge battery it is connected across another capacitor (1.0 farad). The voltage across the capacitor changes from 600V
View SolutionTwo identical capacitors are charged to different potentials φ_(1) and φ_(2) relative to the negative earthed electrodes. The capacitors are then connected in parallel (Fig. 24.21). Find the potential of the battery after the connection was made and the change in the energy of the system.
View Solution
