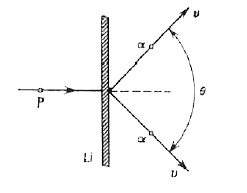
Protons accelerated by a potential difference of 6.8 MV bombard a stationary lithium target. The collision of a proton with a nucleus of Li7 isotope results in the birth of two alpha-particles which separate symmetrically with respect to the direction of the proton beam. Find the kinetic energy and the separation angle of the alpha-particles.
The correct Answer is:12.1MeV;158.4∘.
The nuclear reaction takes the form 1H1+3Li7=22He4. The kinetic energy of the alpha-particle is found from the law of conservation of energy which for this reaction is of the form
2Kα=(MH+MLi−2Mα)×931.5+KH=24.2MeV
SInce the kinetic energy of the alpha-particle turns out to be much lower than its rest energy, the alpha-particles produced in the reaction are nonrelativistic. The separation angle is found from the law of conservation of momentum
pH=2pαcosθ2
But for a nonrelativistic particle p=√2mK, so
cosθ2=12√mHKHmαKα
समान प्रश्न
A proton and an alpha - particle are accelerated through same potential difference. Then, the ratio of de-Broglie wavelength of proton and alpha-particle is
View SolutionA proton is bombarded on a stationary lithium nucleus. As a result of the collision, two α-particles are produced. If the direction of motion of the α-particles with the initial direction of motion makes an angles cos−1(1/4), find the kinetic energy of the striking proton. Given, binding energies per nucleon of Li7 and He4 are 5.60 and 7.06 MeV, respectively.
(Assume mass of proton ≈ mass of neutron).
View SolutionProtons accelerated by a potential difference of 6.8 MV bombard a stationary lithium target. The collision of a proton with a nucleus of Li7 isotope results in the birth of two alpha-particles which separate symmetrically with respect to the direction of the proton beam. Find the kinetic energy and the separation angle of the alpha-particles.
View SolutionIf an alpha particle and a proton are accelerated from rest by a potential difference of 1MeV, then the ratio of their kinetic energies will be
View SolutionA proton is bombarded on a stationary lithium nucleus. As a result of the collision two alpha -particles are produced. If the direction of motion of the alpha -particles with the initial direction of motion makes an angle cos ^(-1) ((1)/(4)), find the kinetic energy of the striking proton. Given binding energies per nucleon of Li^(7) and He^(4) are 5.60 and 7.06 MeV respectively. (Assume mass of proton = mass of neutron)
View SolutionA proton and an α- particle are accelerated through the same potential difference. The ratio of de-Broglie wavelength of the proton to that of the α particle will be
View SolutionA proton and an α- particle are accelerated through the same potential difference. Find the ratio of de-Broglie wavelength of the proton to the α-particel
View Solution
