Write the equation for the Verhulst-Pearl logistic growth of population.
Step-by-Step Solution:
1. Understanding the Logistic Growth Model:
- The logistic growth model describes how a population grows in an environment with limited resources. It results in an S-shaped curve, indicating that the population grows rapidly at first, then slows down as it approaches the carrying capacity of the environment.
2. Identifying the Key Variables:
- In the logistic growth equation, we have:
- dndt: the rate of change of the population over time.
- n: the current population density.
- R: the intrinsic growth rate of the population.
- K: the carrying capacity of the environment (the maximum population size that the environment can sustain).
3. Writing the Logistic Growth Equation:
- The equation for the logistic growth of a population can be expressed as:
dndt=Rn(K−nK)
- Here, K−nK represents the fraction of the carrying capacity that is still available for the population to grow.
4. Understanding the Components of the Equation:
- The term Rn indicates that the growth rate is proportional to the current population size.
- The term K−nK decreases as the population n approaches the carrying capacity K, thus reducing the growth rate as resources become limited.
5. Conclusion:
- The Verhulst-Pearl logistic growth equation effectively models how populations grow in a limited environment, taking into account both the growth potential and the constraints imposed by resource availability.
- The logistic growth model describes how a population grows in an environment with limited resources. It results in an S-shaped curve, indicating that the population grows rapidly at first, then slows down as it approaches the carrying capacity of the environment.
- In the logistic growth equation, we have:
- dndt: the rate of change of the population over time.
- n: the current population density.
- R: the intrinsic growth rate of the population.
- K: the carrying capacity of the environment (the maximum population size that the environment can sustain).
- The equation for the logistic growth of a population can be expressed as:
dndt=Rn(K−nK)
- Here, K−nK represents the fraction of the carrying capacity that is still available for the population to grow.
- The term Rn indicates that the growth rate is proportional to the current population size.
- The term K−nK decreases as the population n approaches the carrying capacity K, thus reducing the growth rate as resources become limited.
- The Verhulst-Pearl logistic growth equation effectively models how populations grow in a limited environment, taking into account both the growth potential and the constraints imposed by resource availability.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - ORGANISMS AND POPULATIONSEXERCISE - PRACTICE QUESTIONS ( SHORT ANSWER TYPE -I QUESTIONS)16videos
ORGANISMS AND POPULATIONS
- BOOK - ICSECHAPTER - ORGANISMS AND POPULATIONSEXERCISE - PRACTICE QUESTIONS ( SHORT ANSWER TYPE -II QUESTIONS)18videos
ORGANISMS AND POPULATIONS
- BOOK - ICSECHAPTER - ORGANISMS AND POPULATIONSEXERCISE - COMPETITION CORNER (ASSERTION AND REASON TYPE QUESTIONS)22videos
ORGANISMS AND POPULATIONS
- BOOK - ICSECHAPTER - MOLECULAR BASIS OF INHERITANCEEXERCISE - MCQ (Source Based Questions)23videos
MOLECULAR BASIS OF INHERITANCE
- BOOK - ICSECHAPTER - PRINCIPLES OF INHERITANCE AND VARIATIONEXERCISE - MULTIPLE CHOICE QUESTION98videos
PRINCIPLES OF INHERITANCE AND VARIATION
Similar Questions
Assertion : A population growing in a habitat with limited resources shows initially a lag phase, followed by phase of accelerated and deceleration and finally as asymptote, when the population density reaches the carrying capacity.
Reason : In Verhulst-Pearl Logistic growth, plot of N (population density) at time (t) results in a sigmoid curve.View Solutionwhich of the following option is true for verhulst Pearl logistic growth?
(A)population growth is decreased by the equation dNdt=rN[KK−N].
(B) r' represents biotic potential.
(C) applicable to a population growing in habitat with limited resources.
(D)K represents carrying capacityView Solution
Knowledge Check
- Question 1 - Select One
Which of the following equations correctly represents Verhulst-Pearl logistic growth ?
AdN/dt=rN(K−NK)BdN/dt=rNKCdN/dt=N(K−N)KDdN/dt=r(K−N)K
What is a logistic growth ?
View SolutionWhich of the following curve represents Verhulst Pearl Logistic growth in a populations ?
View SolutionThe growth of human population shows
View SolutionIn the equation for S-shaped population growth, represents
View SolutionStudy the population growth curves in the graph given below and answer the questions which follow :
(i) Identify the growth curves ‘a’ and ‘b’.
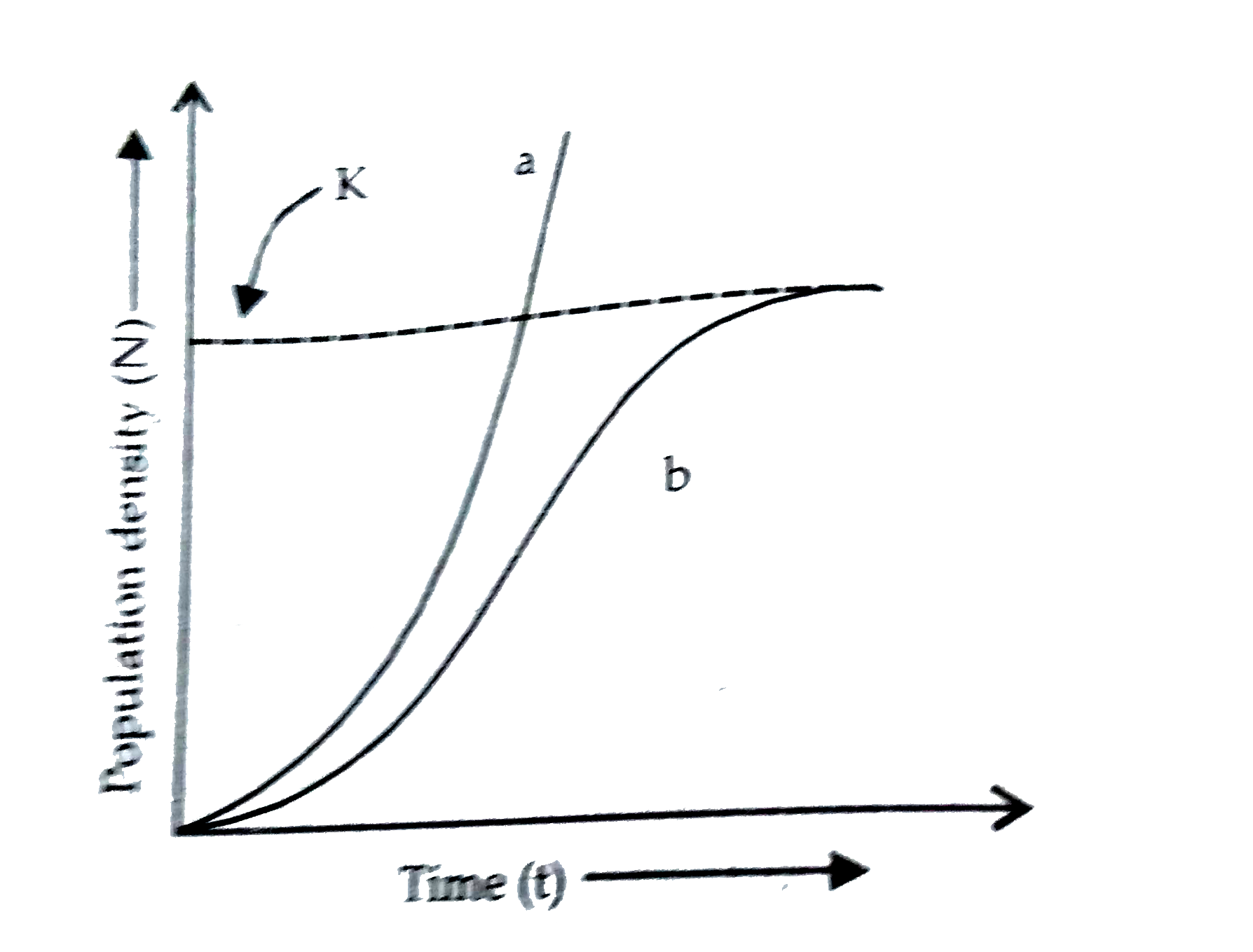
(ii) Which one. of them is considered a more realistic one and why ?
If dN9dt)=rN(K−NK) is the equation of the logistic growth curve, what does K stand for ?
(iv) What is symbolised by N ?View SolutionWhich has an explosive impact on the growth of population ?
View SolutionWhich of the following factors would not limit the growth of a population?
View Solution

