If W1andW2 are the weights of two reactants in any reaction, having their equivalent weights E1andE2 respectively, which of the following equations represents the law of equivalence correctly?
(a) W1E1=W2E2 (b) W1E2=W2E1 ( c ) W1W2=E1E2
Text Solution
The answer is (b), because number of equivalents
=W1/E1=W2/E2.
|
Topper's Solved these Questions
- CHEMICAL EQUIVALENCEBOOK - RC MUKHERJEECHAPTER - CHEMICAL EQUIVALENCEEXERCISE - PROBLEMS
24 Videos
- CHEMICAL EQUILIBRIUMBOOK - RC MUKHERJEECHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Objective Problems
57 Videos
- CHEMICAL KINETICSBOOK - RC MUKHERJEECHAPTER - CHEMICAL KINETICSEXERCISE - OBJECTIVE PROBLEMS
51 Videos
Similar Questions
Knowledge Check
If m1 and m2 are masses of two reactants in any reaction, having their gram equivalent masses E1 and E2 respectively, which of the following equatios represents the law of equivence correctly?
AmE_=m2E2Bm1m1=E1ECm1E2=m2E1D√m1/E1=√m2/E2If W1andW2 are the equivalent weight of two reactants in any reation, having their equivalent weights E1andE2 respectively. Which of the following option is correct?
AW1E1=W2E2BW1E2=W2E1CW1W2=E1E2DAllIf the weight of a body in vacuum is w and w1 and w2 are weights when it is immersed in a liquid of specific gravity ρ1 and ρ2 respectively, then find the relation among w,w1andw2
Aw=w1ρ2+w2ρ1w1+w2Bw=w1ρ2−w2ρ1ρ2−ρ1Cw=w1ρ2+w2ρ1ρ1+ρ2Dω22gr2
In the circuit, the cells E1andE2 have emfs of 4V and 8V and internal resistance 0.5Ωand1.0Ω, respectively. Calculate the current through 6Ω resistance.
View SolutionIn the ciruit the cells E1andE2 have emfs of 4V and 8V and internal resistance 0.5Ωand1.0Ω, respectively. Calculate the current through 6Ω resistance.

View SolutionIn the circuit shown in figure find:
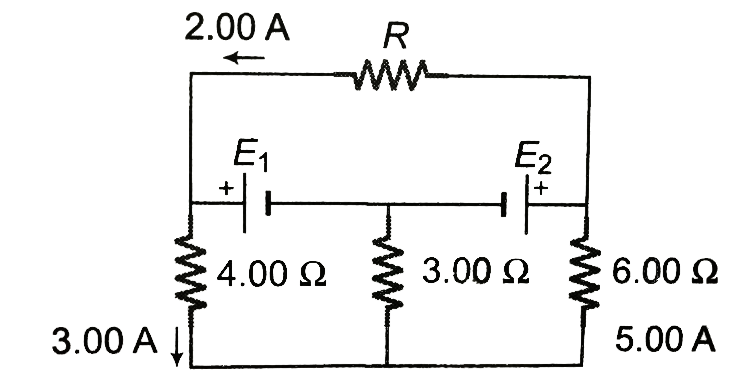
a. the current in the 3.00Ω resistor,b. the unknown emfsE1 and E2 and c the resistance R.
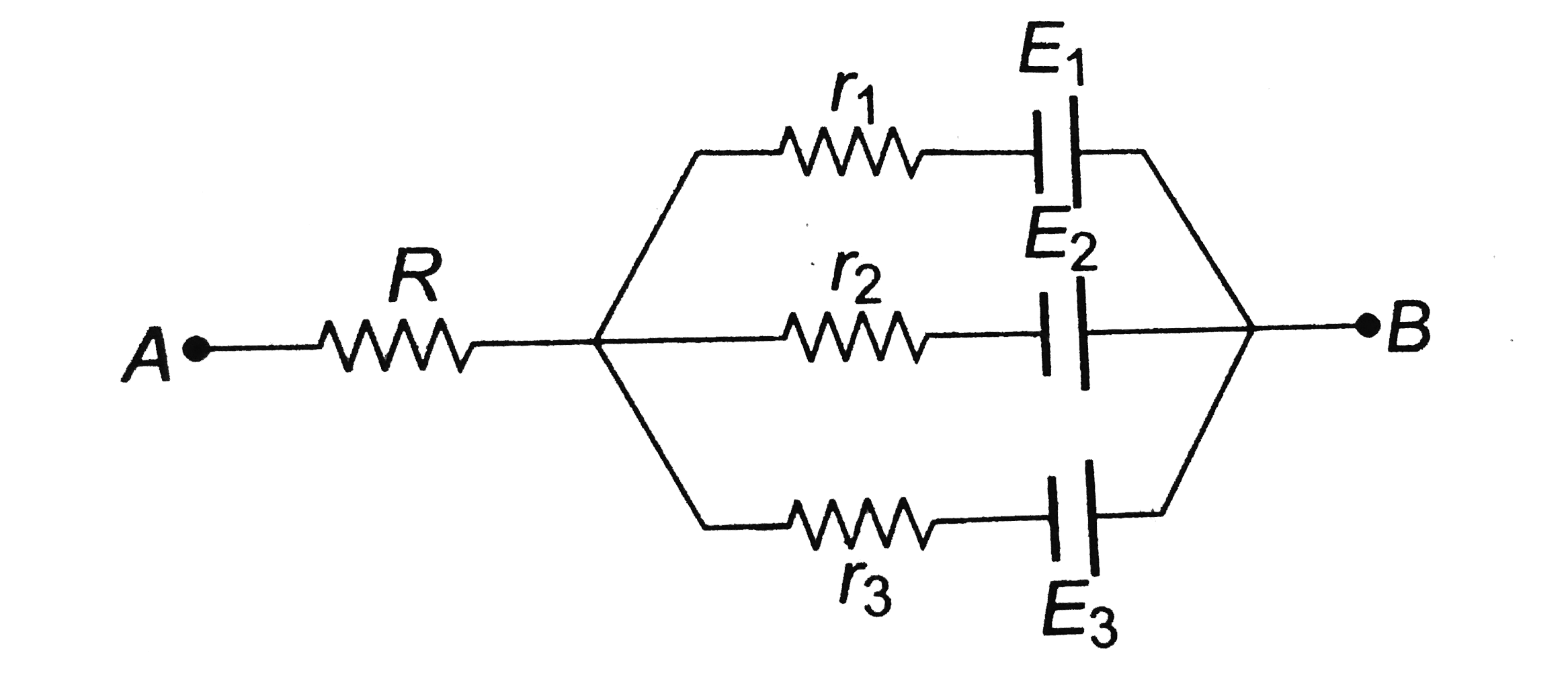
View SolutionIn the network shown the potential difference between A and B is (R=r1=r2=r3=1Ω,E1=3V,E2=2V,E3=1V)
View SolutionIf W1:W2=2:3 and W1:W3=1:2 then W2:W3 is
View Solution
