The number of points required to name a line is
1
2
3
4
The correct Answer is:B
To determine the number of points required to name a line, we can follow these steps:
1. Understanding a Line: A line is a straight path that extends infinitely in both directions. It does not have endpoints.
2. Identifying Points: To name a line, we need to identify at least two distinct points on that line.
3. Naming the Points: Let's denote these points as Point A and Point B.
4. Conclusion: Since a line is defined by extending infinitely in both directions, we need a minimum of two points to uniquely identify and name that line.
Thus, the answer is that 2 points are required to name a line.
Similar Questions
The number 0 (zero) is required for
View SolutionFrom an algebraic expression to give the number of line segments required to make the following pattern and hence find the number of line segments required for 10 houses.

View SolutionFind a rule that gives the number of line segments required to make the following pattern.

View SolutionFrom an algebraic expression to give the number of line segments required to make the following pattern and hence find the number of line segments required to make the 10th pattern.
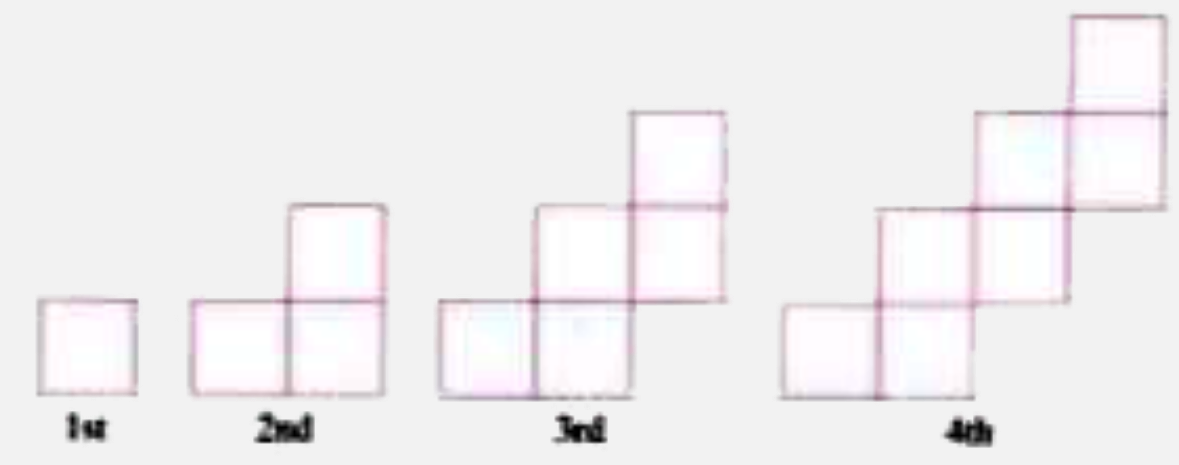
View SolutionForm the algebraic expression to give the number of line segments required to make the given pattern. Also, find the number of line segments required to make 14th pattern of the series.

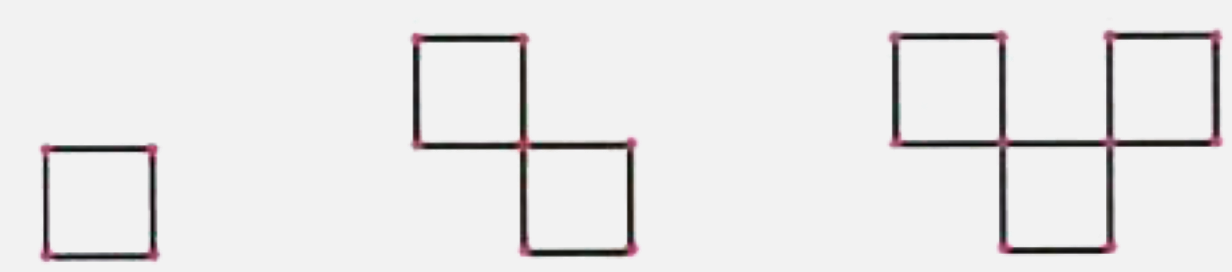
View SolutionFind a rule that gives the number of line segments required to make the following pattern of squares.
View SolutionA line passes through A(1,1)andB(100,1000). The number of points with integral co-ordinates on the line strictly between A and B is
View SolutionThere are n-points (n>2) in each to two parallel lines. Every point on one line is joined to every point on the other line by a line segment drawn within the lines. The number of point (between the lines) in which these segments intersect, is
View SolutionIf there are six straight lines in a plane, no two of which are parallel and no three of which pass through the same point, then find the number of points in which these lines intersect.
View Solution25 lines are drawn in a plane. Such that no two of them are parallel and no three of them are concurrent. The number of points in which these lines intersect, is:
View Solution
