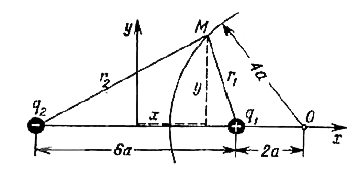
Two electric charges $q_{1} = q and q_{2} = - 2 q$ are placed at a distance l = 6a apart. Find the locus of points in the plane of the charges where the field potential is zero

Text Solution

Verified by Experts

Direct the coordinate axes as shown in Fig. 24.2. Let M be one of the points where the potential is zero:
$φ = φ_{1} = \frac{q}{4 π e p o s i_{0} r_{1}} - \frac{2 q}{4 π ε_{0} r_{2}} = 0$
Hence $r_{2} = 2 r_{1}$
Substituting $r_{1} = \sqrt{{(3 a - x)}^{2} + y^{2}} and r_{2} = \sqrt{{(3 a + x)}^{2} + y^{2}}$ , we obtain after some manipulations
${(x - 5 a)}^{2} + y^{2} = 16 a^{2}$
This is the equation of a circle of radius 4a, with centre at (5a, 0)

Updated on:21/07/2023

Two electric $+ q and + 4 q$ are placed at a distance 6a apart on a horizontal plane. Find the position of the point on the line joining the two charges where the electric field is zero.
A2a from +q
B3a from +q
C2a from +4q
Da from +q
Two positive point charges $q_{1} = 16 μ C$ and $q_{2} = 4 μ C$ are separated in vacuum by a distance of $3.0 m$ . Find the point on the line between the charges where the net electric field is zero.-
A $2 m$ from $q_{1}$
B $1 m$ from $q_{1}$
C $2 m$ from $q_{2}$
D $0.5 m$ from $q_{1}$