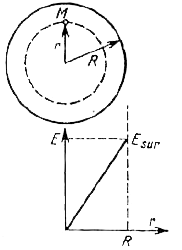
Find the electric field at an arbitrary point of a sphere carrying a charge uniformly distributed over its volume.
Let the volume density of the charge ρ=QV=3Q4πR3, where R is the radius of the sphere. Choose a point M Inside the spliere al a distance r<R from its centre and consider a concentric sphere through it. From the results obtained in Problem 24.13 it is evident that the spherical layer lying outside point M does not create a field at this point. The field is created solely by the chargeq=43πr3ρ contained inside the smaller sphere. By the result of the previous problem, the field at the surlace of this sphere, 1.e. at point M, will be
E=q4πε0r2=4πr3ρ3×4πε0r2=ρr3ε0
We see that the field inside the sphere increases in proportion to the radius, at the centre its i tensity is zero, and on the surface it is
Esur=ρR3ε0=Q4πε0R2
This dependence is plotted in Fig. 24.15. 24.16. The feld potentials on the surfaces of both spheres are φ=q/(4πε0R),φ0=q/(4πε0,R1) respectively. The potential difference is
φ−φ1=q4πε0(1R−1R1)=qR(_(1)−R)4πε0RR1
The Capacitance is C=qφ−φ1=4πε0RR1R1−R2
If d=R1−Rlr<R, we obtaine the approximation C=4πε0R2d=ε0Sd
which is the expression for the capacitance of a plane capacitor. The error is
δ=Csp−CplCap=R1−RR=dR
Similar Questions
There is one charged tiny spherical oil drop and the magnitude of electric field intensity at a point on its surface is E. If n such drops are combined to make a single drop then what will be the net electric field intensity at a point on the surface of the bigger spherical drop. Assume that charge is uniformly distributed over the volume of oil drop.
View SolutionCalculate electric field due to a charge uniformly distributed in a spherical volume
View Solution
Knowledge Check
Assertion: Electric potential on the surface of a charged sphere of radius R is V. Then electric field at distance r=R2 from centre is V2R. Charge is distributed uniformly over the volume.
Reason: From centre to surface, electric field varies linearly with r. Here r is distance from centre.AIf both Assertion and Reason are true but Reason is the correct explanation of Assertion.BIf both Assertion and Reason are true but Reason is not the correct explanation of Assertion.CIf Assertion is true, but the Reason is false.DIf Assertion is false but the Reason is true.
Calculate electric field due to a charge uniformly distributed in a spherical volume
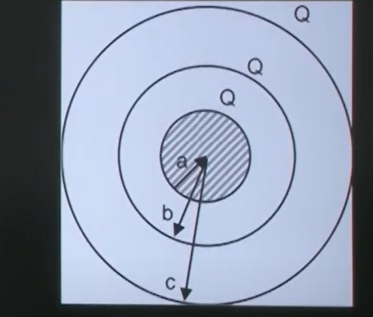
View SolutionFind the graph between electric field intensity and distance 'r' from the center. For the given arrangement of concentric spheres. Charge in inner solid sphere is uniformly distributed in volume.
View SolutionProve that the field on the surface of a sphere carrying a uniformly distributed electric charge is equal to that which would have been established, if the entire charge were concentrated in the centre of the sphere.
View SolutionProve that the field on the surface of a sphere carrying a uniformly distributed electric charge is equal to that which would have been established, if the entire charge were concentrated in the centre of the sphere.
View SolutionAssertion: Electric potential on the surface of a charged sphere of radius R is V. Then electric field at distance r=R2 from centre is V2R. Charge is distributed uniformly over the volume.
Reason: From centre to surface, electric field varies linearly with r. Here r is distance from centre.
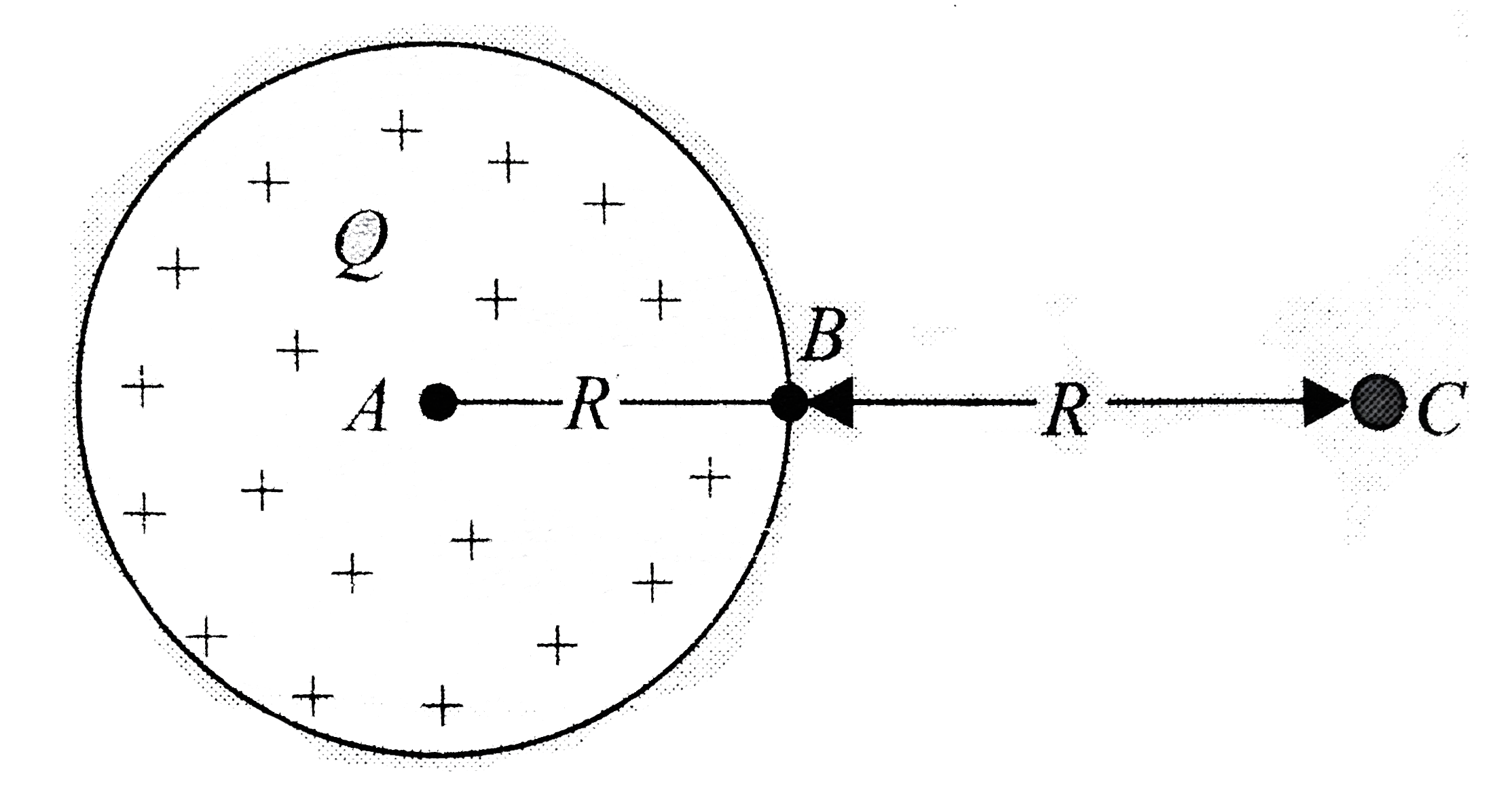
View SolutionFind the electric work done in bringing a charge q from A to B in a sphere of charge Q distributed uniformly throughout its volume.
 .
.
View SolutionFind the electric work done in bringing a charge q from A to B in a sphere of charge Q distributed uniformly throughout its volume.
 .
.
View Solution
