A spherical shell of radius R carries a uniformly distributed charge q. The electrical forces arising cause the ex-pansion of the shell. Find the mechanical stress in the shell.
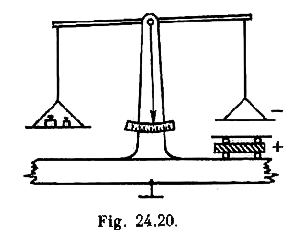
The mechanical stress is equal to the energy density of the electric field (see 37.8). We have p=w0=ε0E2/2. But we have already found the field on the surface of a sphere (see Problem 24.14): E=q/(4πε0R2). Hence the result sought.
Similar Questions
A spherical shell of radius R has a uniformly distributed charge ,then electric field varies as
View SolutionA thin spherical shell radius of R has a charge Q uniformly distributed on it. At the centre of the shell, a negative point charge -q is placed. If the shell is cut into two identical hemispheres, still equilibrium is maintained. Then find the relation between Q and q?

View Solution
Knowledge Check
A spherical shell of radius R has a uniformly distributed charge ,then electric field varies as
Ainside is zero and varies as x−1 outside itBinside is non zero constant and varies as x2 outside itCinside is zero and varies as x−2 outside itDinside is constant and varies as x−1 outside itA spherical shell of radius R has a charge +q units. The electric field due to the shell at a point
Ainside is zero and varies as r−1 outside itBinside is constant and varies as r−2Cinside is zero and varies as r−2 outside itDinside is constant and varies as r−1 outside itA spherical conducting shell of radius r0 carry a charge q0. Then value of electric field inside it is :-
Akq0/r2Bkq0/r3CzeroDuniform but non zero
A spherical shell having charge +Q (uniformly distributed) and a point charge +q0 are placed as shown. Find the force between shell and the point charge (r>>R).
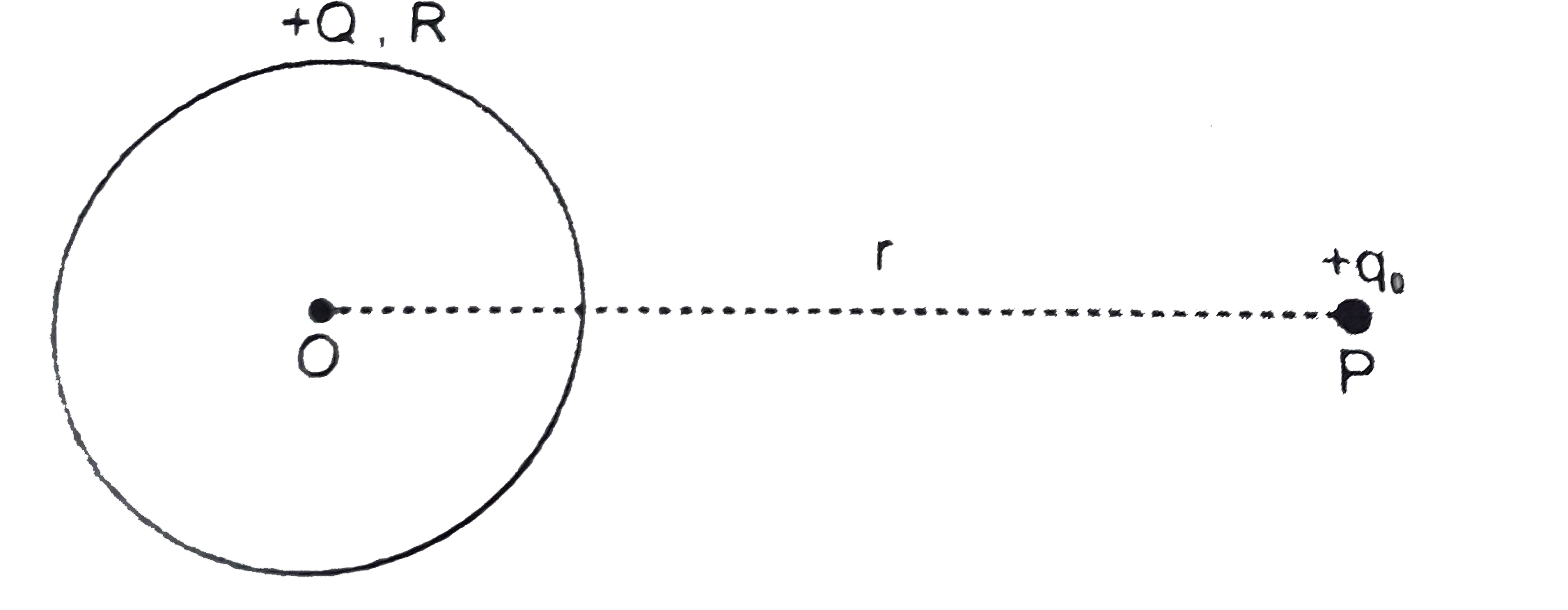
View SolutionA spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q.
A charge q is placed at the centre of the shell. Find out the surface charge density on the inner and outer surface of the shell.
Is the electric field inside a cavity (with no charge ) zero. Independent of the fact wheather the shell is spherical or not ? Explain.
View SolutionA non-conducting spherical shell of radius R surrounds a point charge q (q at center). The electric flux through a cap of the shell of half angle θ is:
View SolutionA non-conducting spherical shell of radius R surrounds a point charge q (q at center). The electric flux through a cap of the shell of half angle θ is:
View SolutionA non-conducting spherical shell of radius R surrounds a point charge q (q at center). The electric flux through a cap of the shell of half angle θ is:
View Solution
