Find the force of interaction between two current carrying coils, the distance between the centres of which is much greater than their linear dimensions (Fig. 28.16).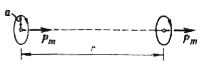

Text Solution

Verified by Experts

The correct Answer is: $F = - \frac{6 μ_{0} p_{m}^{2}}{4 π r^{4}}$

It is easily seen that the moving coils hanging freely will arrange themselves so that their planes will be perpendicular to their common axis. The force of interaction is $F = p_{m} \frac{d B}{d x}$ Noting that
$\frac{d B}{d x} = \frac{6 μ_{0} p_{m} x}{4 π {(a^{2} + x^{2})}^{5 / 2}}$
(see Problem 27.11) and that according to the condition of the problem $x \approx r \geq \geq a,$ we have $\frac{d B}{d x} = \frac{6 μ_{0} p_{m}}{4 π r^{4}}$
The force of interaction is $F = p_{m} \frac{d B}{d x} = - \frac{6 μ_{0} p_{m}^{2}}{4 π r^{4}}$
It is quite similar to the force of interaction between electric dipoles.

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Similar Questions

Knowledge Check

Question 1 - Select One
There will be no force between two current carrying wires if currents are
Aparallel to each other
Bantiparallel to each other
Cperpendicular to each other
Dnothing can be said
Question 2 - Select One
The force between two parallel current carrying wires is independent of
Atheir distance of separation
Bthe length of the wires
Cthe radii of the wires
Dthe medium in which they are placed
Question 3 - Select One
The force between two parallel current carrying wires is independent of
Atheir distance of separation
Bthe length of the wires
Cthe magnitude of currents
Dthe radii of the wires

Recommended Questions

Home
Ask Doubt
Profile