A core with the shape and dimensions in millimeters shown in Fig. 30.14 has been manufactured of a ferromagnetic material whose magnetization curve is shown in Fig. 29.9a (p. 76). One layer of wire of 0.6 mm diameter (including insulation) was closely wound on the core. Find the inductance of the coil for a current of 200 mA flowing in it. Find the energy of the magnetic field and the energy density. 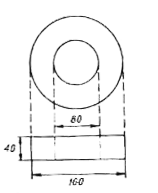
The correct Answer is:41H; 0.82J ; 1.36×102J/m3
To find the number of turns divide the length of the torus internal circuiference by the wire diameter. We obtain.
wπD/d=80π/0.6=420
The magnetic field strength is H=iwl−iwπDm=IDdDm
where Dm=120nm is twice the distance from cho axis of revolution of the torus to the centro of tbo gonorating circle.
Knowing the magnetic field strength and making use of the graph of Fig. 20.9a, we find the induction of the field. Applying the formula wm=BH/2 we find the energy density, and multiplying this by the volume of the core we find the total onergy of the magnetic field. The inductance is found using the formula Wm−Li2/2
Similar Questions
Knowledge Check
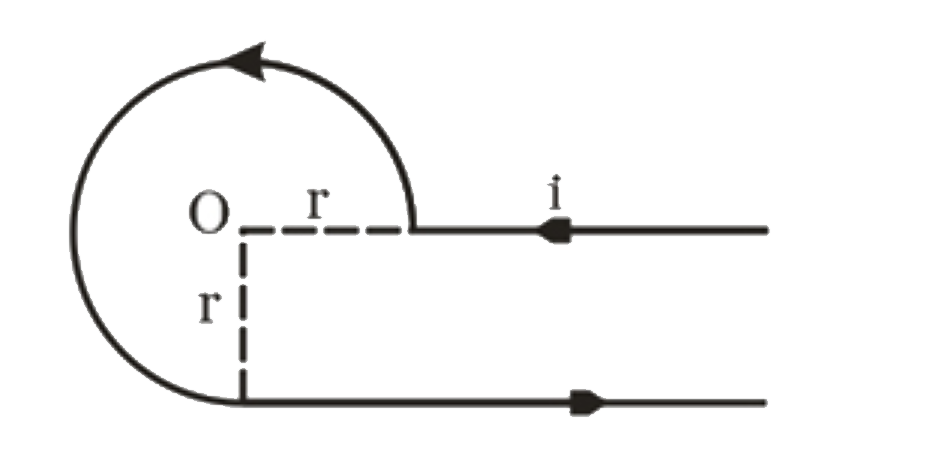
Find the magnetic field at the origin in the fig. Shown in fig.
 Aμ0I4R(34ˆk+1πˆj)Bμ0I4R(32ˆk+1πˆj)Cμ0I4R(34ˆk+12πˆj)DNone of these
Aμ0I4R(34ˆk+1πˆj)Bμ0I4R(32ˆk+1πˆj)Cμ0I4R(34ˆk+12πˆj)DNone of theseFind the magnetic induction at point O, if the current carrying wire is in the shape shown in the figure.
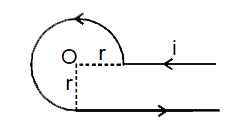
 Aμ0l5πr[3π2+1]⊙Bμ0l3πr[3π4+1]⊙Cμ0l2πr[3π4+1]⊙Dμ0l4πr[3π2+1]⊙
Aμ0l5πr[3π2+1]⊙Bμ0l3πr[3π4+1]⊙Cμ0l2πr[3π4+1]⊙Dμ0l4πr[3π2+1]⊙
A long wire having a semi-circular loop of radius r carries a current I, as shown in Fig. Find the magnetic field due to entire wire.

View SolutionFind the magnetic induction at point O, if the current carrying wire is in the shape shown in the figure
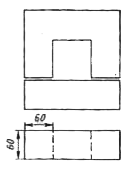
View SolutionThe core and the armature of an electromagnet with dimensions in millimeters as shown in Fig. 30.16 have been manufactured from a ferromagnetic material whose properties have been described in Problem 29.10. What is the force with which the core attracts the armature, if the material has been magnetized to saturation? What force will remain active after the current is switched off?
View SolutionFind the magnetic induction at point O, if the current carrying wire is in the shape shown in the figure.

View SolutionFind the magnetic induction at point O if the current carrying wire is in the shape shown in the figure.
 .
.
View SolutionA circular coil of 200 mm diameter is made of 100 turns of thin wire and carries a current of 50 mA. Find the magnetic field induction at the centre of the coil.
View Solution
