A spherical copper weight of 3.0 cm radius submerged in olive oil hangs from a spring whose elasticity coefficient (force constant) is 1.0×102N/m Fig. Find the natural frequency of the oscillatory system, its Q-factor and the time the oscillations will take to practically damp out.

The correct Answer is:1.6Hz;2.1xx10^(2);21s
Qk/hω0, where h=Ffr/v=6πrη
Similar Questions
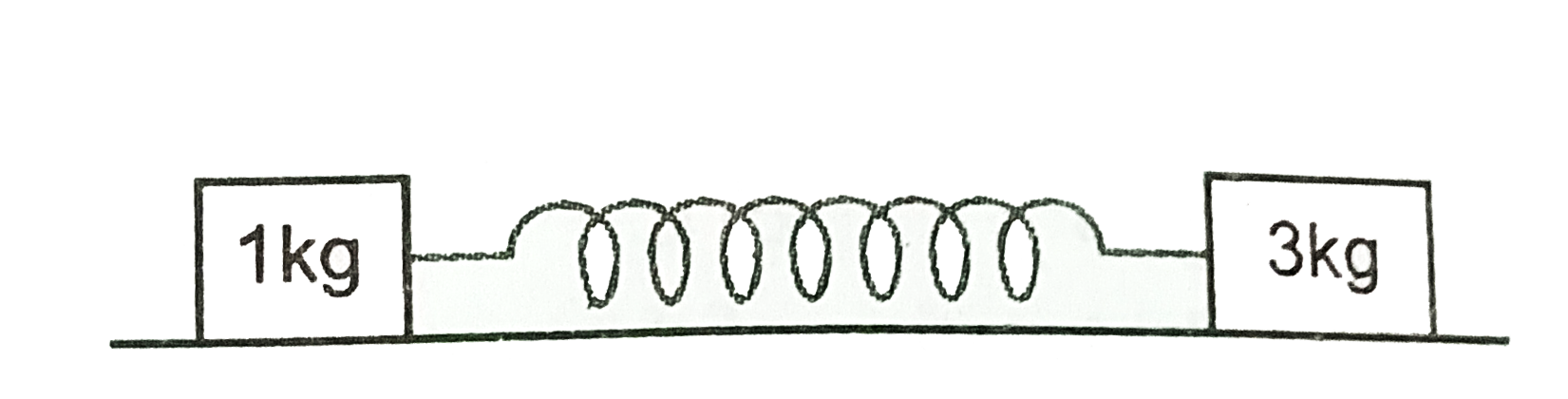
Two point masses of 3.0 kg and 1.0 kg are attached to opposite ends of a horizontal spring whose spring constant is 300Nm−1 as shown in the figure. The natural vibration frequency of the system is of the order of :
 View Solution
View SolutionTwo point masses of 3.0 kg and 1.0 kg are attached to opposite ends of a horizontal spring whose spring constant is 300Nm−1 as shown in the figure. The natural vibration frequency of the system is of the order of :
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One or More
A linear harmonic oscilator of force constant 2×106N/m times and amplitude 0.01 m has a total mechanical energy of 160 Joules. Its
AMaximum P.E is 100 J.BMaximum K.E is 100JCMaximum P.E is 160 JDMinimum P.E is zero - Question 2 - Select One
A particle of mass 2g is initially displaced through 2cm and then released. The frictional force constant due to air on it is 12×10−3N/m. The restoring force constant is 50×10−3N/m. If it is in oscillatory motion, its time period is
AπsecBπ/2secC2πsecD4πsec - Question 3 - Select One or More
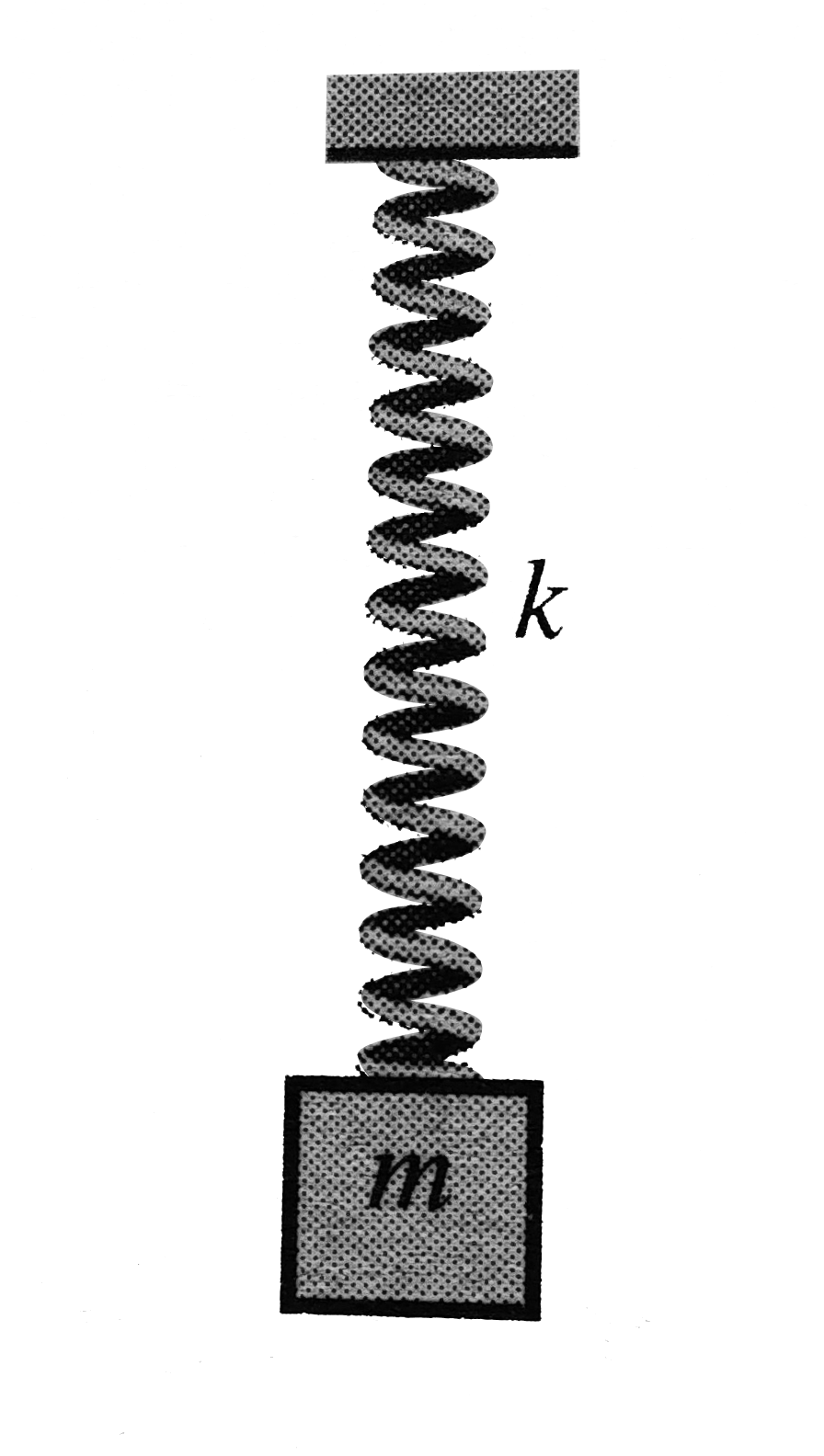
For the spring pendulum shown in fig. the value of spring constant is 3×104Nm and amplitude of oscillation is 0.1m. The total mechanical energy of oscillating system is 200 J. Mark out the correct option (s).AMinimum PE of the oscillating system is 50 JBMaximum PE of the oscillating system is 200JCmaximum KE of the oscillating system is 200 JDminimum KE of the oscillating system is 150 J.
Two point masses of 3.0kg and 1.0kg are attached to opposite ends of a horizontal spring whose spring constant is 3Nm−1 as shown in figure . The natural frequency of vibration so this system is n/πHz. Find the integral value of n.
 View Solution
View SolutionA linear harmonic oscillator of force constant 2×106N/m and amplitude 0.01m has a total mechanical energy of 160J. Its
View SolutionA linear harmonic oscillator of force constant 2×106N/m and amplitude 0.01m has a total mechanical energy of 160J. Its
View Solution
For the spring pendulum shown in fig. the value of spring constant is 3×104Nm and amplitude of oscillation is 0.1m. The total mechanical energy of oscillating system is 200 J. Mark out the correct option (s).View SolutionA linear harmonic oscillator of force constant 2×106N/m and amplitude (0.01 m) has a total mechanical energy of (160 J). Its.
View Solution

