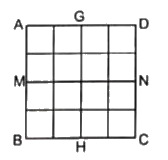
Is it possible to write the numbers 17, 18, 19,…32 in a 4×f grid of unit squares, with one number in each square, such that the product of the numbers in each 2×2 sub-grids AMRG, GRND, MBHR and RHCN is divisible by 16?
Text Solution
The correct Answer is:Not possible
|
Topper's Solved these Questions
- COMBINATORICSBOOK - RESONANCECHAPTER - COMBINATORICSEXERCISE - Exercise-2 (Part-I: Previous Asked Question for Pre RMO)
23 Videos
- APPLICATION OF DERIVATIVESBOOK - RESONANCECHAPTER - APPLICATION OF DERIVATIVESEXERCISE - High Level Problems (HLP)
33 Videos
- DEFINITE INTEGRATION & ITS APPLICATION BOOK - RESONANCECHAPTER - DEFINITE INTEGRATION & ITS APPLICATION EXERCISE - High Level Problem
37 Videos
Similar Questions
Knowledge Check
If the square of the sum of two numbers is equal to 4 times of their product, then the ratio of these numbers is:
A2:1B1:3C1:1D1:2The least number , which is a perfect square and is divisible by each of the numbers 16, 20 and 24 is
A1600B3600C6400D14400
Find the smallest square number that is divisible by each of the numbers 4, 9 and 10.
View SolutionFind the smallest square number that is divisible by each of the numbers 4, 9 and 10.
View SolutionFind the smallest square number divisible by each one of the numbers 8, 9 and 10.
View SolutionThe least number which is a perfect square and is divisible by each of 16, 20 and 24 is
View SolutionWrite down the square of each of the following numbers in the form of the number multiplied by itself.
-2.7
View SolutionWrite following numbers using the square notation.
(−25)×(−25)
View Solution
