A particle is thrown with a velocity u at angle θ from the horizontal. Another particle is thrown with the same velocity at an angle a from the vertical. The ratio of times of flight of the two particles will be
Tan2θ:1
Cot2θ:1
Tanθ:1
Cotθ:1
The correct Answer is:C
Similar Questions
A particle is thrown with velocity u at an angle ∝ from the horizontal. Another particle is thrown with the same velocity at an angle ∝ from the verticle. What will be the ratio of times of flight of two particles ?
View SolutionA particle is thrown with velocity u at an angle ∝ from the horizontal. Another particle is thrown with the same velocity at an angle ∝ from the verticle. What will be the ratio of times of flight of two particles ?
View Solution
Knowledge Check
A particle is thrown with a speed u at an angle θ with the horizontal. When the particle makes an angle ϕ with the horizontal. Its speed changes to v :
Av=ucosθBv=ucosθ.cosϕCv=ucosθ.secϕDv=usecθ.cosϕA particle is thrown with a speed is at an angle θ with the horizontal. When the particle makes an angle ϕ with the horizontal, its speed changes to v, then
Av=v0cosθBv=v0cosθ⋅cosϕCv=v0cosθ⋅secϕDv=v0secθ⋅cosϕA ball is thrown up with a certain velocity at angle θ to the horizontal. The kinetic energy varies with height h of the particle as:
A B
B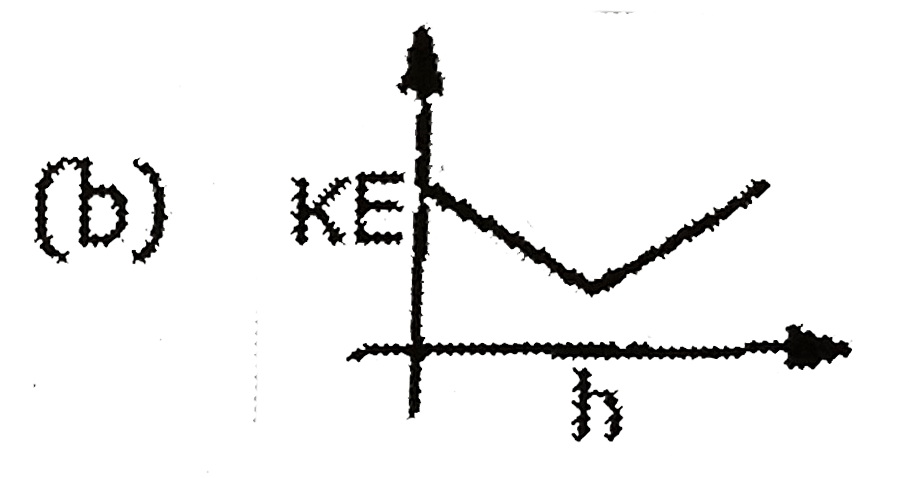 C
C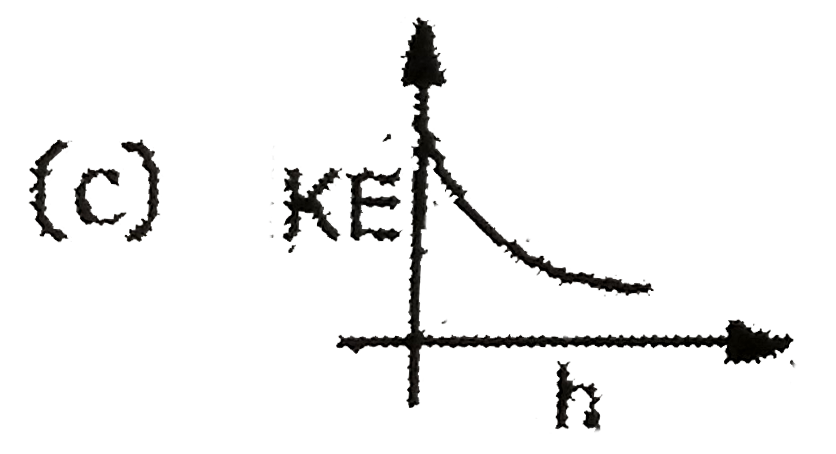 D
D
A particle is thrown with a speed u at an angle θ with the horizontal. When the particle makes an angle ϕ with the horizontal. Its speed changes to v :
View SolutionA particle is projected form ground with velocity u ar angle θ from horizontal. Match the following two columns.

View SolutionA particle is projected form ground with velocity u ar angle θ from horizontal. Match the following two columns.

View SolutionA particle is thrown with speed u of an angle theta with the horizontal when the particle makes an angle phi with the horizontal its speed changes to v
View SolutionA ball is thrown up with a certain velocity at angle θ to the horizontal. The kinetic energy varies with height h of the particle as:
View Solution
