Explain the working of a venturimeter, Obtain an expression for the volume of liquid flowing per second through pipe.
Step-by-Step Solution
Step 1: Understanding the Venturimeter
A Venturimeter is a device used to measure the flow velocity of liquids in a pipe. It operates based on the principle of Bernoulli's theorem, which relates the pressure, velocity, and height of a fluid in motion.
Step 2: Structure of the Venturimeter
The Venturimeter consists of a pipe with a gradually narrowing section (the throat). The wider section has an area A1 and the narrower section has an area A2. As the liquid flows through the Venturimeter, its velocity changes due to the change in cross-sectional area.
Step 3: Applying the Equation of Continuity
According to the equation of continuity, the product of the cross-sectional area and the velocity at any two points in a flow must be constant. Therefore, we can write:
A1V1=A2V2
where V1 is the velocity at the wider section and V2 is the velocity at the narrower section.
Step 4: Applying Bernoulli's Theorem
Bernoulli's theorem states that for an incompressible, non-viscous fluid, the sum of pressure energy, kinetic energy, and potential energy per unit volume remains constant. We can express this as:
PA+12ρV21+ρgh=PB+12ρV22+ρgh
Since both points A and B are at the same height, the potential energy terms cancel out:
PA+12ρV21=PB+12ρV22
Step 5: Rearranging the Bernoulli's Equation
Rearranging the above equation gives:
PA−PB=12ρV22−12ρV21
This can be simplified to:
PA−PB=ρ2(V22−V21)
Step 6: Relating Pressure Difference to Height Difference
The pressure difference PA−PB can also be expressed in terms of the height difference h of the liquid column in the manometer connected to the Venturimeter:
PA−PB=ρgh
Equating the two expressions gives:
ρgh=ρ2(V22−V21)
Step 7: Simplifying the Equation
Cancelling ρ from both sides leads to:
2gh=V22−V21
Rearranging gives:
V22=2gh+V21
Step 8: Substituting for V2
Using the equation of continuity V2=A1A2V1 in the above equation:
(A1A2V1)2=2gh+V21
This leads to:
A21A22V21=2gh+V21
Step 9: Solving for V1
Rearranging gives:
(A21A22−1)V21=2gh
Thus,
V21=2ghA21A22−1
Taking the square root yields:
V1=
⎷2ghA21A22−1
Step 10: Finding Volume Flow Rate
The volume flow rate Q (volume of liquid flowing per second) can be calculated as:
Q=A1V1
Substituting for V1:
Q=A1
⎷2ghA21A22−1
A Venturimeter is a device used to measure the flow velocity of liquids in a pipe. It operates based on the principle of Bernoulli's theorem, which relates the pressure, velocity, and height of a fluid in motion.
The Venturimeter consists of a pipe with a gradually narrowing section (the throat). The wider section has an area A1 and the narrower section has an area A2. As the liquid flows through the Venturimeter, its velocity changes due to the change in cross-sectional area.
According to the equation of continuity, the product of the cross-sectional area and the velocity at any two points in a flow must be constant. Therefore, we can write:
A1V1=A2V2
where V1 is the velocity at the wider section and V2 is the velocity at the narrower section.
Bernoulli's theorem states that for an incompressible, non-viscous fluid, the sum of pressure energy, kinetic energy, and potential energy per unit volume remains constant. We can express this as:
PA+12ρV21+ρgh=PB+12ρV22+ρgh
Since both points A and B are at the same height, the potential energy terms cancel out:
PA+12ρV21=PB+12ρV22
Rearranging the above equation gives:
PA−PB=12ρV22−12ρV21
This can be simplified to:
PA−PB=ρ2(V22−V21)
The pressure difference PA−PB can also be expressed in terms of the height difference h of the liquid column in the manometer connected to the Venturimeter:
PA−PB=ρgh
Equating the two expressions gives:
ρgh=ρ2(V22−V21)
Cancelling ρ from both sides leads to:
2gh=V22−V21
Rearranging gives:
V22=2gh+V21
Using the equation of continuity V2=A1A2V1 in the above equation:
(A1A2V1)2=2gh+V21
This leads to:
A21A22V21=2gh+V21
Rearranging gives:
(A21A22−1)V21=2gh
Thus,
V21=2ghA21A22−1
Taking the square root yields:
V1=
⎷2ghA21A22−1
The volume flow rate Q (volume of liquid flowing per second) can be calculated as:
Q=A1V1
Substituting for V1:
Q=A1
⎷2ghA21A22−1
Topper's Solved these Questions
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SHORT ANSWER QUESTIONS
36 Videos
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - VERY SHORT ANSWER QUESTIONS
8 Videos
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - CONCEPTUAL SHORT ANSWER QUESTIONS WITH ANSWERS
16 Videos
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - SELECTED PROBLEMS (FROM HEAT ENGINES)
21 Videos
- OSCILLATIONS BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SELECTED PROBLEMS (OSCILLATION IN A TUNNEL BORED THROUGH THE EARTH)
2 Videos
Similar Questions
Statement-1 : When an orifice is made in the middle of the wall of a vessel, the range of the liquid coming out of the orifice is equal to the height of the liquid.
Statement-2 : Liquid is flowing through two identical pipes A and B. Volume of liquid flowing per second through A and B are v0and2v0 respectively. Flow in A is turbulent and steady in B.
Statement-3 : Rate of flow of a viscous liquid through a pipe is directly proportional to he fourth power of the radius of pipe.
View SolutionThe rate of a flow V a of liquid through a capillary under a constant pressure depends upon (i) the pressure gradient (P/l) (ii) coefficient of viscosity of the liquid η (iii) the radius of the capillary tube r. Show dimesionally that the rate of volume of liquid flowing per sec V∝ Pr^4 /ηl
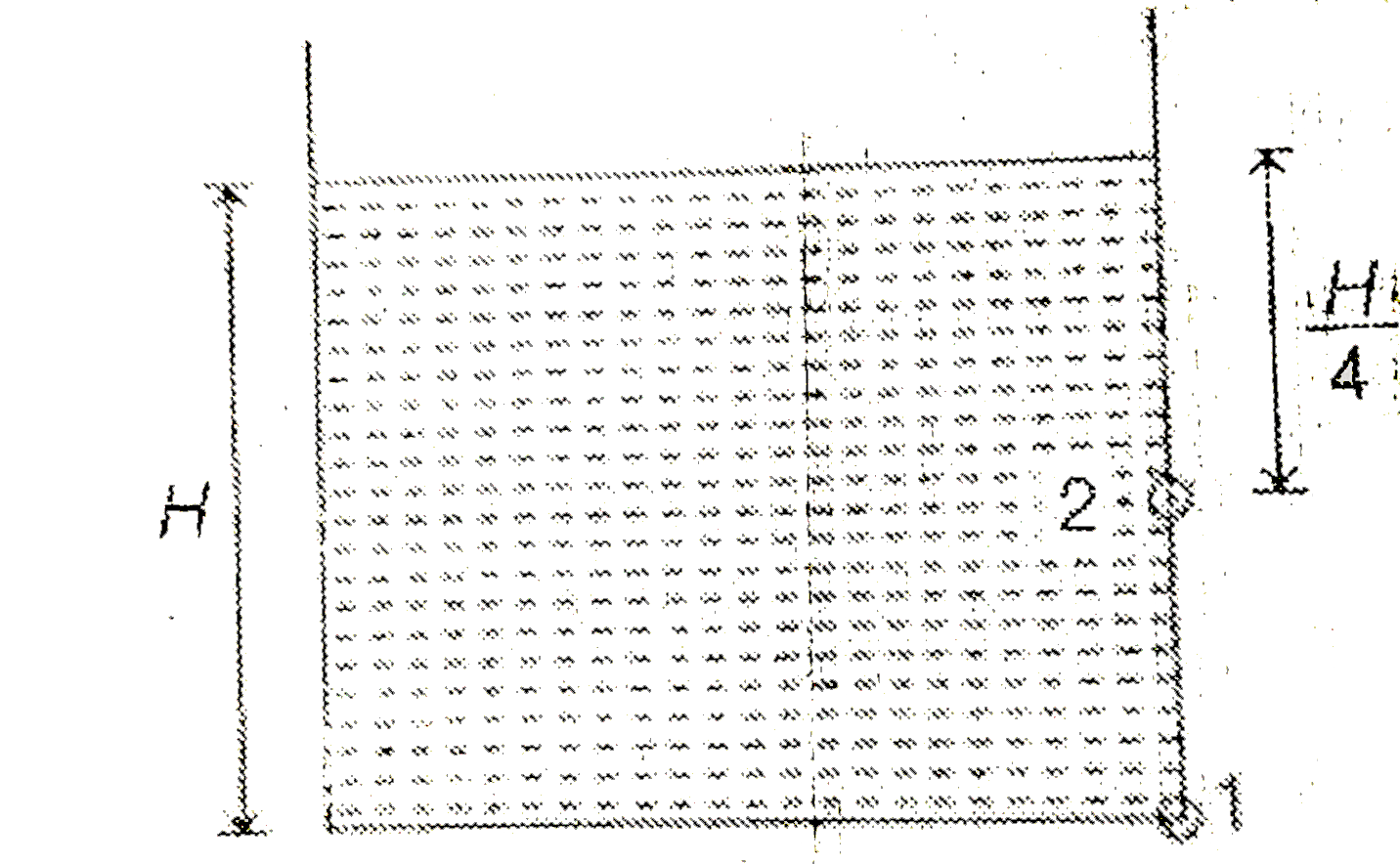
View SolutionThere are two holes on a water tank as shown in figure. Area of hole 2 is two times that of area of hole 1. Suppose v is the speed of liquid coming out and Q the volume of liquid flowing per second. Then
View SolutionExplain the term 'drift velocity' of electrons in a conductor. Hence obtain the expression for the current through a conductor in terms of 'drift velocity'.
View SolutionShow that the pressure energy per unit volume of a liquid is P.
View SolutionThe ratio of the radius of the tube of a venturimeter is η(η>1) . The ratio of the densities of the liquid in the manometer and the moving fluid is η1 . If the difference in heights of the liquid column in the manometer is h , find the minimum speed of flow of the fluid .
View SolutionObtain an expression for the volume strain in a medium when a longitudinal wave propagates through the medium.
View Solution(a) What is a heat engine ?
Name the important part of a heat engine.
(b) Explain the working of a Carnot engine. Obtain an expression for its efficiency.
View SolutionExplain Poiseuille's formula for the volume of liquid flowing through a capillary tube - Describe an experiment to determine the coefficient of viscosity of a liquid by Poiseuiles formula.
View SolutionA dimensionally consistent relation for the volume V of a liquid of coefficiet of viscosity η flowing per second through a tube of radius r and length l and having a pressure difference p across its end, is
View Solution
