Define terminal velocity: A steel ball is dropped in a viscous oil. Draw a simple labelled diagram to show the forces acting on the ball as it falls through the oil.
Step-by-Step Text Solution
Step 1: Define Terminal Velocity
Terminal velocity is defined as the constant speed that a freely falling object eventually reaches when the resistance of the medium through which it is falling prevents further acceleration. In simpler terms, it is the speed at which the force of gravity pulling the object downwards is balanced by the resistance forces acting upwards, resulting in no net force and thus no further acceleration.
Step 2: Identify the Forces Acting on the Steel Ball
When a steel ball is dropped into a viscous oil, three main forces act on it:
1. Weight (W): This is the force due to gravity acting downwards on the ball.
2. Buoyant Force (B): This is the upward force exerted by the fluid (oil) on the ball, which opposes the weight.
3. Viscous Force (F_v): This is the resistance force due to the viscosity of the oil, also acting upwards against the motion of the ball.
Step 3: Draw the Diagram
To illustrate the forces acting on the steel ball as it falls through the viscous oil, we can draw a simple labeled diagram:
↑
| (B) Buoyant Force
|
| (F_v) Viscous Force
|
|
● (Steel Ball)
|
|
↓
| (W) Weight
Step 4: Explain the Forces in the Diagram
- The weight (W) of the ball acts downwards due to gravity.
- The buoyant force (B) acts upwards, opposing the weight of the ball.
- The viscous force (F_v) also acts upwards, opposing the motion of the ball through the viscous oil.
When the steel ball reaches terminal velocity, the sum of the upward forces (buoyant force and viscous force) equals the downward force (weight), resulting in no net force acting on the ball.
Terminal velocity is defined as the constant speed that a freely falling object eventually reaches when the resistance of the medium through which it is falling prevents further acceleration. In simpler terms, it is the speed at which the force of gravity pulling the object downwards is balanced by the resistance forces acting upwards, resulting in no net force and thus no further acceleration.
When a steel ball is dropped into a viscous oil, three main forces act on it:
1. Weight (W): This is the force due to gravity acting downwards on the ball.
2. Buoyant Force (B): This is the upward force exerted by the fluid (oil) on the ball, which opposes the weight.
3. Viscous Force (F_v): This is the resistance force due to the viscosity of the oil, also acting upwards against the motion of the ball.
To illustrate the forces acting on the steel ball as it falls through the viscous oil, we can draw a simple labeled diagram:
↑
| (B) Buoyant Force
|
| (F_v) Viscous Force
|
|
● (Steel Ball)
|
|
↓
| (W) Weight
- The weight (W) of the ball acts downwards due to gravity.
- The buoyant force (B) acts upwards, opposing the weight of the ball.
- The viscous force (F_v) also acts upwards, opposing the motion of the ball through the viscous oil.
Topper's Solved these Questions
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - VERY SHORT ANSWER QUESTIONS
8 Videos
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SELECTED PROBLEMS ( FROM PRESSURE, ARCHIMEDES. PRINCIPLE)
7 Videos
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - LONG ANSWER QUESTIONS
5 Videos
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - SELECTED PROBLEMS (FROM HEAT ENGINES)
21 Videos
- OSCILLATIONS BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SELECTED PROBLEMS (OSCILLATION IN A TUNNEL BORED THROUGH THE EARTH)
2 Videos
Similar Questions
Knowledge Check
Spherical balls of radius R are falling in a viscous fluid of velocity v .The retarding viscous force acting on the spherical ball is
Adirectly proportional to R but inversely proportional v.Bdirectly proportional to both radius R and velocity v.Cinversely proportional to both radius R and velocity v.Dinversely proportional to R but directly proportional to velocity v.After terminal velocity is reached the acceleration of a body falling through a viscous fluid is:
AzeroBequal to gCless than gDmore than gAfter terminal velocity is reached the acceleration of a body falling through a viscous fluid is:
AzeroBequal to gCless than gDmore than g
Define geotropism. Draw a labelled diagram of a plant showing geotropic movements of its parts.
View SolutionThe terminal velocity v of a spherical ball of lead of radius R falling through a viscous liquid varies with R such that
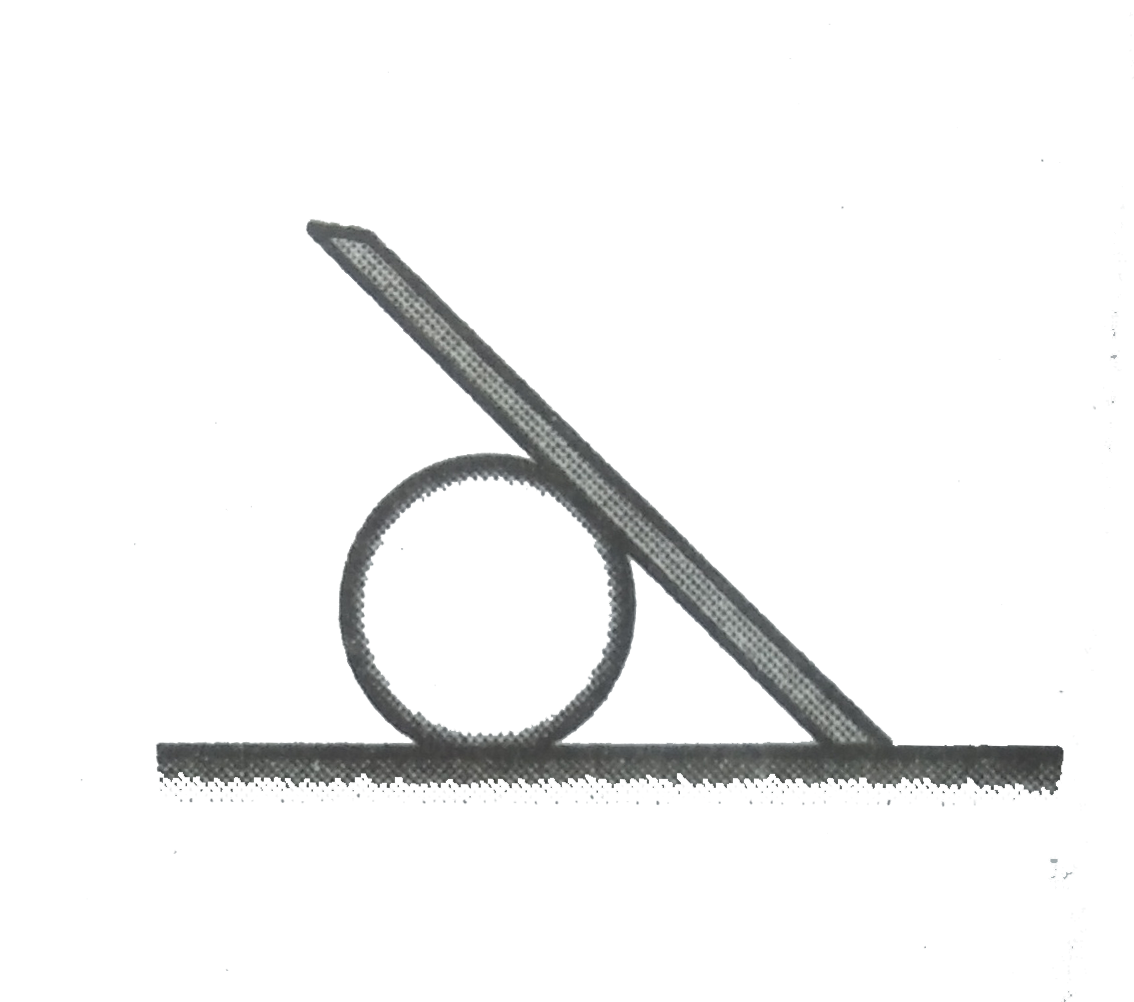
View SolutionThe diagram shows a rough plank resting on a cylinder with one and of the plank on rough ground. Negtect friction between plank and cylinder. Draw digrams to show
(a) the force acting on the plank,
(b)the force acting on the cylinder.
View SolutionA small spherical ball falling through a viscous medium of negligible density has terminal velocity v. Another ball of the same mass but of radius twice that of the earlier falling through the same viscous medium will have terminal velocity
View SolutionA spherical ball falls through viscous medium with terminal velocity v. If this ball is replaced by another ball of the same mass but half the radius, then the terminal velocity will be (neglect the effect of buoyancy.)
View Solution
