A cylindrical metallic rod 0.5 m long conduct heat at the rate of 50Js−1 when its ends are kept a 400∘Cand0∘C respectively. Coefficient of thermal conductivity of metal is 72Wm−1K−1. What is the diameter of rod?
The correct Answer is:0.033 m
To find the diameter of the cylindrical metallic rod, we can use the formula for the rate of heat conduction:
dQdt=K⋅A⋅ΔTL
Where:
- dQdt = rate of heat transfer (in watts or joules per second)
- K = coefficient of thermal conductivity (in W/m·K)
- A = cross-sectional area of the rod (in m²)
- ΔT = temperature difference (in K or °C)
- L = length of the rod (in m)
Step 1: Identify the given values
- Length of the rod, L=0.5m
- Rate of heat transfer, dQdt=50J/s
- Coefficient of thermal conductivity, K=72W/m⋅K
- Temperature difference, ΔT=400−0=400K
Step 2: Calculate the cross-sectional area A
The area A of a cylindrical rod can be expressed in terms of its diameter d:
A=πd24
Step 3: Substitute the known values into the heat conduction formula
Substituting the values into the heat conduction formula:
50=72⋅πd24⋅4000.5
Step 4: Simplify the equation
First, simplify the equation:
50=72⋅πd24⋅4000.5
50=72⋅πd2⋅4002
50=36⋅πd2⋅400
50=14400⋅πd2
Step 5: Solve for d2
Rearranging gives:
d2=5014400⋅π
Step 6: Calculate d2
Using π≈3.14:
d2=5014400⋅3.14≈5045216≈0.001107
Step 7: Calculate d
Taking the square root to find d:
d=√0.001107≈0.0332m
Step 8: Convert to centimeters
To convert meters to centimeters:
d≈0.0332m×100≈3.32cm
Final Answer
The diameter of the rod is approximately 3.32cm.
---
- dQdt = rate of heat transfer (in watts or joules per second)
- K = coefficient of thermal conductivity (in W/m·K)
- A = cross-sectional area of the rod (in m²)
- ΔT = temperature difference (in K or °C)
- L = length of the rod (in m)
- Length of the rod, L=0.5m
- Rate of heat transfer, dQdt=50J/s
- Coefficient of thermal conductivity, K=72W/m⋅K
- Temperature difference, ΔT=400−0=400K
The area A of a cylindrical rod can be expressed in terms of its diameter d:
A=πd24
Substituting the values into the heat conduction formula:
50=72⋅πd24⋅4000.5
First, simplify the equation:
50=72⋅πd24⋅4000.5
50=72⋅πd2⋅4002
50=36⋅πd2⋅400
50=14400⋅πd2
Rearranging gives:
d2=5014400⋅π
Using π≈3.14:
d2=5014400⋅3.14≈5045216≈0.001107
Taking the square root to find d:
d=√0.001107≈0.0332m
To convert meters to centimeters:
d≈0.0332m×100≈3.32cm
The diameter of the rod is approximately 3.32cm.
Topper's Solved these Questions
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - SELECTED PROBLEMS (Taken from the Previous Years ISC, AISSCE, HSSCE various States. Boards Roorke Qns & NCERT text) FROM MASS OF ICE MELTED
17 Videos
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - SELECTED PROBLEMS (Taken from the Previous Years ISC, AISSCE, HSSCE various States. Boards Roorke Qns & NCERT text) FROM THE RATE OF INCREASE OF THICKNESS OF ICE ON A LAKE
3 Videos
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - VERY SHORT ANSWER QUESTIONS
5 Videos
- SELF ASSESSMENT PAPER 5BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 5EXERCISE - Section-D
6 Videos
- THERMAL RADIATION BOOK - ICSECHAPTER - THERMAL RADIATION EXERCISE - SELECTED PROBLEMS (from WIEN.S DISPLACEMENT LAW)
14 Videos
Similar Questions
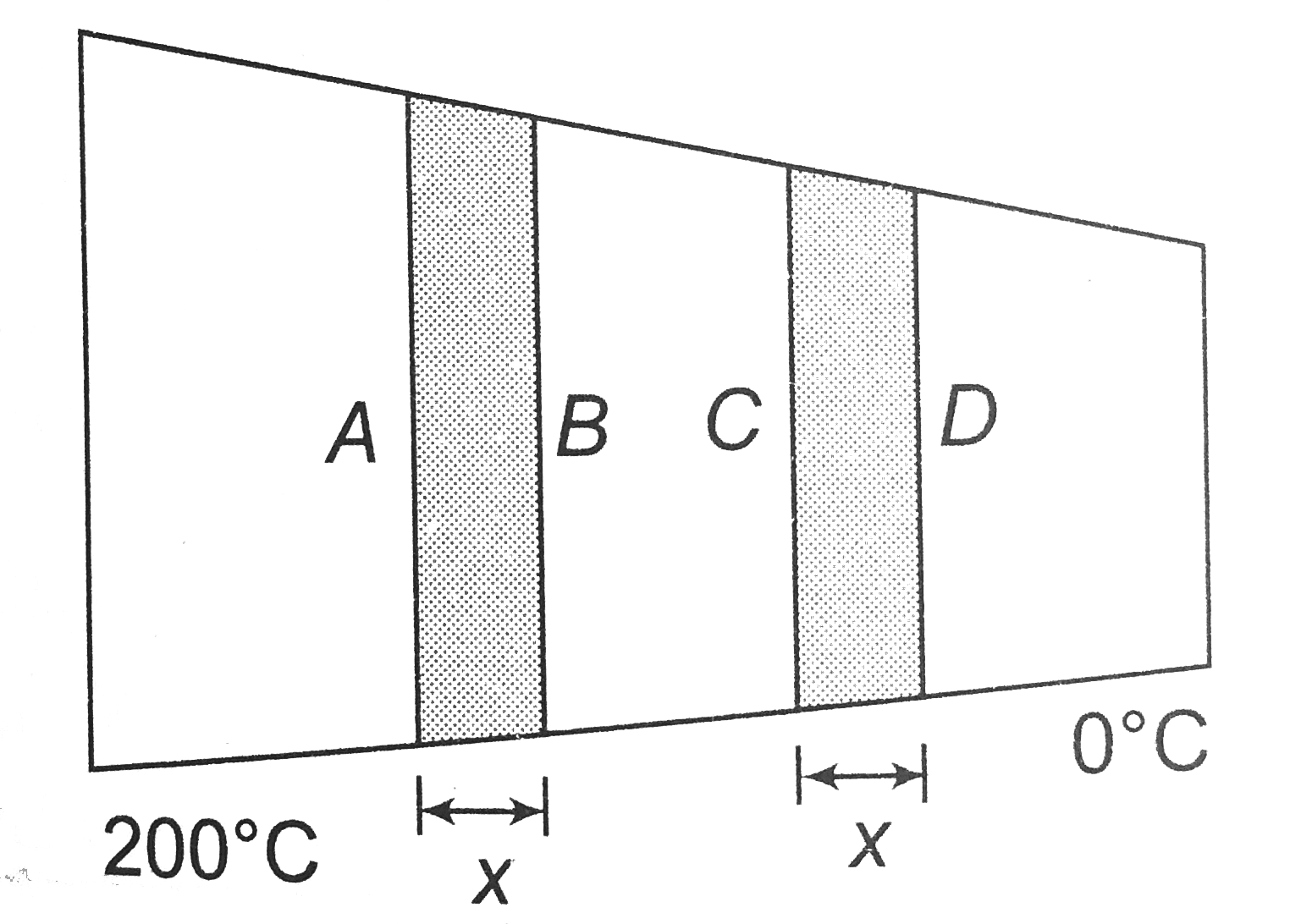
Two ends of a conducting rod of varying cross-section are maintained at 200∘C and 0∘C respectively. In steady state:
View SolutionA metallic bar is heated from 0∘C to 100∘C. The coefficient of linear expansion is 10−5K−1. What will be the percentage increase in length
View SolutionTwo identical metal rods A and B are joined end to end. The free end of A is kept at 27°C and the free end of B at 37°C. Calculate the temperature of the interface. Thermal conductivity of A=385Wm−1K−1, that of B 110Wm−1K−1.
View SolutionA conducting cylindrical rod of uniform cross-sectional area is kept between two large chambers which are at temperatures 100∘C and 0∘C, respectively. The cconductivety of the rod increases with x, where x is distance from 100∘C end. The temperature profile of the rod in steady -state will be as

View SolutionIn a steady state of thermal conduction, temperature of the ends A and B of a 20 cm long rod are 100∘C and 0∘C respectively. What will be the temperature of the rod at a point at a distance of 9 cm from the end A of the rod
View SolutionA metallic rod of cross-sectional area 20 cm2, with the lateral surface insulated to prevent heat loss, has one end immersed in boiling water and the other in ice water mixture. The heat conducted through the rod melts the ice at the rate of 1 gm for every 84 sec. The thermal conductivity of the rod is 160Wm−1K−1. Latent heat of ice=80 cal/gm, 1 ca=4.2 joule. What is the length (in m) of the rod?
View SolutionFigure shows a copper rod joined to a steel rod. The rods have equal length and and the equal cross sectional area. The free end of the copper rod is kept at 0∘C and that of the steel rod is kept at 100∘C . Find the temperature at the junction of the rods. conductivity of copper =390Wm−1C−1 and that of steel =46Wm−1C−1 .

View SolutionThe ends of copper rod of length 1m and area of cross section 1cm2 are maintained at 0∘C and 100∘C. At the centre of rod, there is a source of heat of 32W. The thermal conductivity of copper is 400W/mK

View SolutionA steel bar 10.0 cm long is welded end to end to a copper bar 20.0 cm long. Both bars are insulated perfectly on their sides . Each bar has a separate cross-section, 2.00 cm on a side . The free end of the steel bar is maintained at 100∘C by placing it in contact with steam and free end of the copper bar is maintained at 0∘C by placing it in contact with ice . find the temperature at the junction of the two bars and the total rate of heat flow . thermal conductivity of steel =50.2Wm−1K−1.thermal conductivity of copper =385Wm−1K−1.
View SolutionTemperature of hot end and cold end of a rod, which is in steady state are 100∘C and 40∘C respectively. The area of cross section of rod and its thermal conductivity are uniform. The temperature of rod at its mid point is (Assume no heat loss thorugh lateral surface)
View Solution
