If D is such a point on the side BC of △ABC that AB/AC=BD/CD, then AD must be a/an :
altitude of △ABC
median of △ABC
angle bisector of △ABC
perpendicular bisector of △ABC
The correct Answer is:C
To solve the problem, we need to determine what type of line segment AD is in triangle ABC, given the condition that ABAC=BDCD.
1. Understanding the Given Condition:
We are given that ABAC=BDCD. This means that the ratio of the lengths of sides AB and AC is equal to the ratio of the lengths of segments BD and CD.
2. Applying the Angle Bisector Theorem:
The Angle Bisector Theorem states that if a point D lies on the side BC of triangle ABC such that ABAC=BDCD, then AD is the angle bisector of angle A. This theorem indicates that the point D divides the opposite side (BC) in the same ratio as the other two sides (AB and AC).
3. Conclusion:
Since the condition ABAC=BDCD holds true, we can conclude that AD must be the angle bisector of angle A in triangle ABC.
Final Answer:
AD must be an angle bisector of triangle ABC.
---
We are given that ABAC=BDCD. This means that the ratio of the lengths of sides AB and AC is equal to the ratio of the lengths of segments BD and CD.
The Angle Bisector Theorem states that if a point D lies on the side BC of triangle ABC such that ABAC=BDCD, then AD is the angle bisector of angle A. This theorem indicates that the point D divides the opposite side (BC) in the same ratio as the other two sides (AB and AC).
Since the condition ABAC=BDCD holds true, we can conclude that AD must be the angle bisector of angle A in triangle ABC.
AD must be an angle bisector of triangle ABC.
Topper's Solved these Questions
Similar Questions
Knowledge Check
If D is such a point on the side, BC of ΔABC that ABAC=BDCD, then AD must be a/an:
Aaltitude of ΔABCBmedian of ΔABCCangle bisector of ΔABCDperpendicular bisector of ΔABCD is any point on side AC of a ΔABC with AB=AC then
ACD<BDBCD=BDCCD>BDDNONEIf D id the mid-point of the side BC of a triangle ABC and AD is perpendicular to AC , then
Aa2+b2+c2=4b2Ba2+b2−c2=4b2Ca2−b2+c2=4b2Da2−b2−c2=4b2
If D is the mid point of side BC of a triangle ABC such that →AB+→AC=λ →AD, write the value of λ.
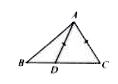
View SolutionD is a point on side BC of triangle ABC such that AD = AC (see figure). Show that AB>AD.
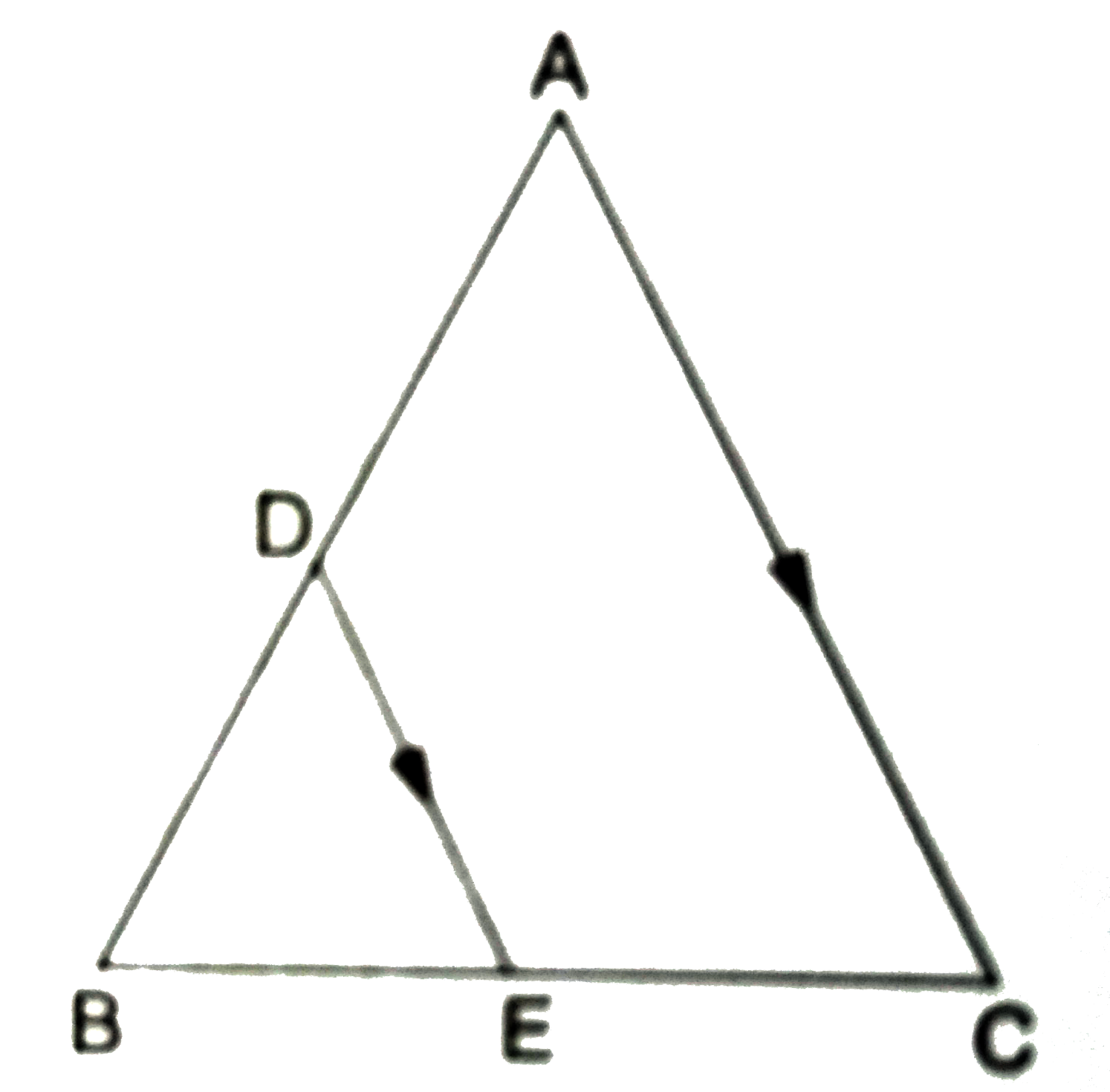
View SolutionIf D is a point on the side AB of ΔABC such that AD:BD=3:2andE is a point on BC such that DE∣∣AC, find the ratio of the areas of ΔABCandΔDBE
View SolutionIf D is a point of the base BC of an isosceles triangle ABC such that AD is perpendicular to AC, then a=
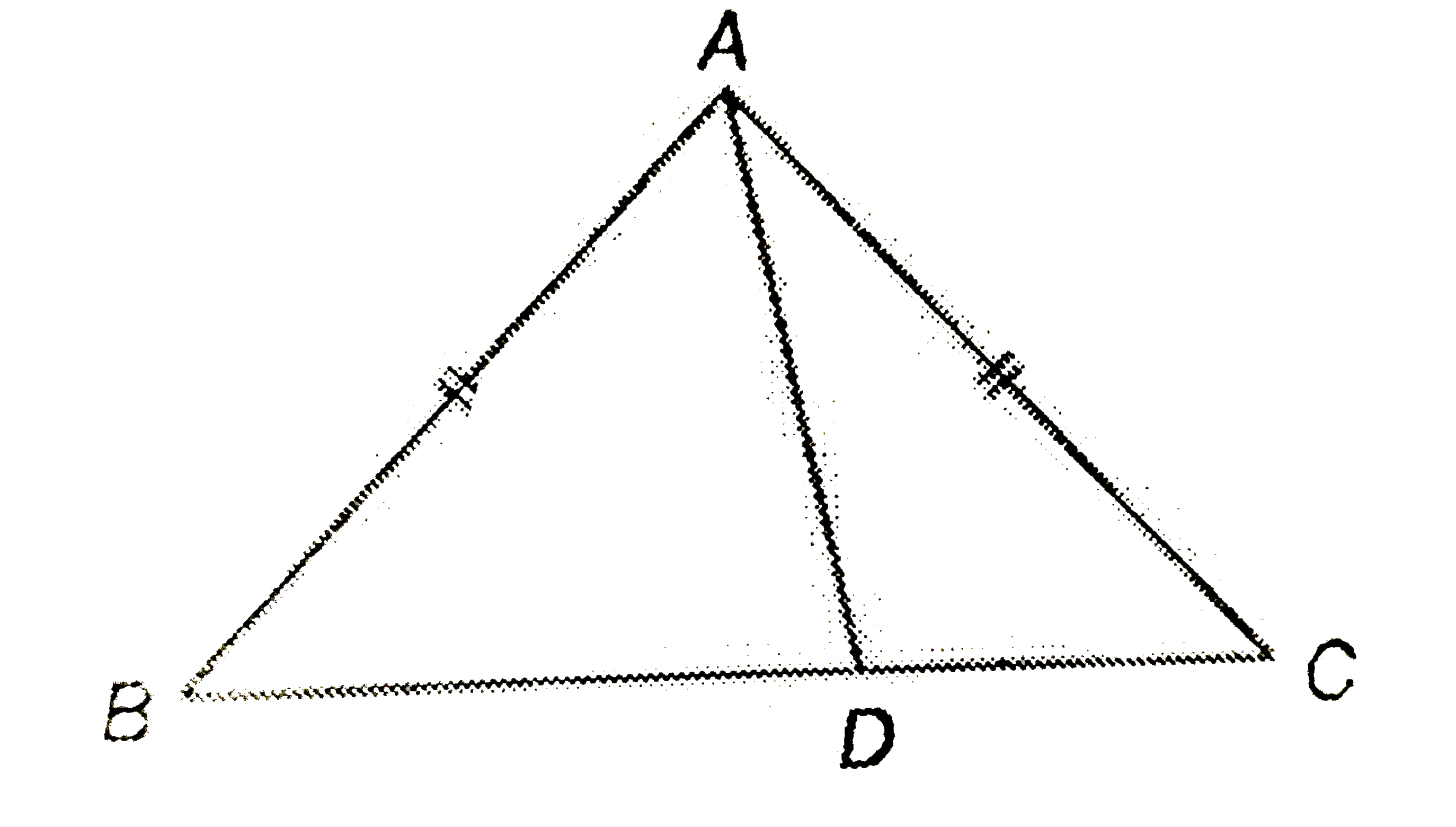
View SolutionIf D any point on the BC of an isosceles triangle ABC then prove that AB>AD and AC>AD.
View Solution
