In a hostel there are.250 students. 120 watch news channels, 80 watch sports channels and 90 watch music channels. Each channel telecasts the content as per their nature. 50 students watch both news and sports channel 60 students watch both sports and music channel and 65 students watch both news and music channels. What is the maximum number of students who watch all the three types of channels?
To solve the problem, we will use the principle of inclusion-exclusion for three sets. Let's denote:
- A: the set of students who watch news channels.
- B: the set of students who watch sports channels.
- C: the set of students who watch music channels.
Given data:
- Total students, n(U)=250
- Students who watch news channels, n(A)=120
- Students who watch sports channels, n(B)=80
- Students who watch music channels, n(C)=90
- Students who watch both news and sports, n(A∩B)=50
- Students who watch both sports and music, n(B∩C)=60
- Students who watch both news and music, n(A∩C)=65
Let x be the number of students who watch all three types of channels, i.e., n(A∩B∩C)=x.
Using the principle of inclusion-exclusion, we can express the total number of students who watch at least one type of channel as follows:
n(A∪B∪C)=n(A)+n(B)+n(C)−n(A∩B)−n(B∩C)−n(A∩C)+n(A∩B∩C)
Substituting the known values into the equation:
250=120+80+90−50−60−65+x
Now, let's simplify the equation step by step:
1. Calculate the sum of the number of students watching each type of channel:
120+80+90=290
2. Calculate the sum of the students watching two types of channels:
50+60+65=175
3. Substitute these values back into the equation:
250=290−175+x
4. Simplify the right side:
250=115+x
5. Solve for x:
x=250−115
x=135
Thus, the maximum number of students who watch all three types of channels is 135.
- B: the set of students who watch sports channels.
- C: the set of students who watch music channels.
- Students who watch news channels, n(A)=120
- Students who watch sports channels, n(B)=80
- Students who watch music channels, n(C)=90
- Students who watch both news and sports, n(A∩B)=50
- Students who watch both sports and music, n(B∩C)=60
- Students who watch both news and music, n(A∩C)=65
120+80+90=290
50+60+65=175
250=290−175+x
250=115+x
x=250−115
x=135
Topper's Solved these Questions
Similar Questions
Find the 11th term of -2,3,8,13………
View SolutionWhich of the following is/are the solution(s) of x2−7×+12=0
View SolutionA survey shows that 63% of the people watch a news channel whereas 76% watch another channel. If x% of the people watch both channel then,
View SolutionA survey shows that 63 % of the pepole watch a news channel whereas , 76 % watch an entertiament channel at a particular time If X% of the pepole watch both types of channels , then
View SolutionA survey shows that 60% of the people watch a News achannel, whereas 76% watch another channel. If x% of the people watch both are channels, then
View SolutionThere are 100 children in a colony. Of them, 70 watch Disney channel, 50 watch both Cartoon network and Disney channels, and 20 watch none of these. Find the number of children who watch only cartoon network.
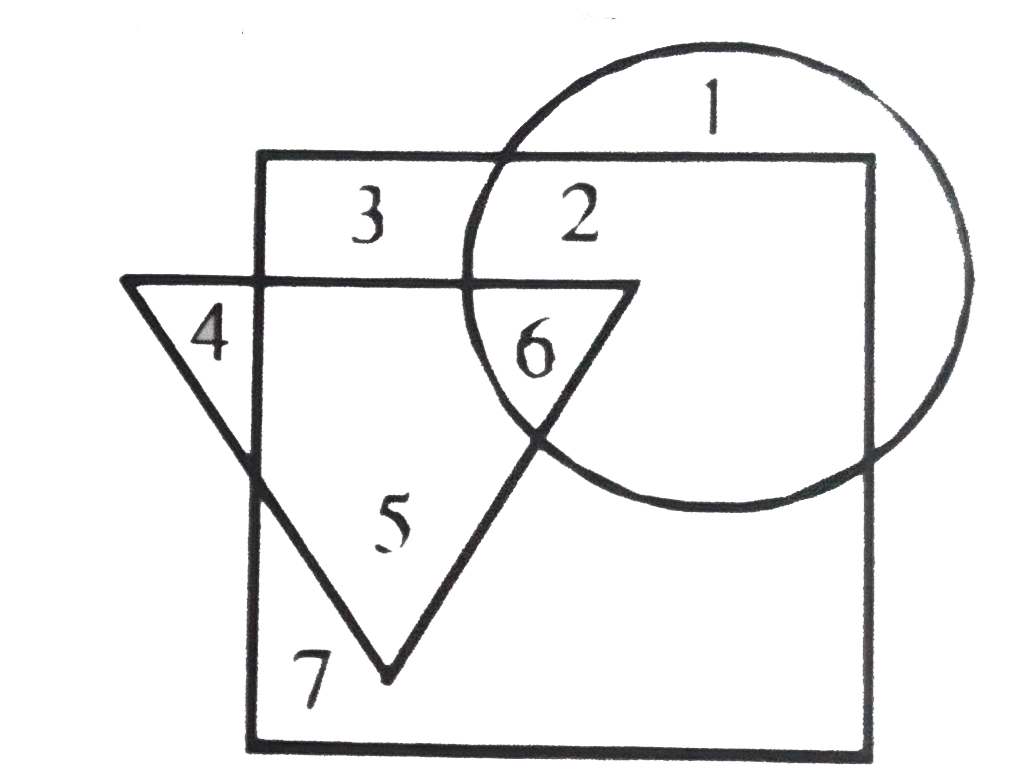
View Solutionटीवी दर्शकों की पसंद के बारे में सर्वक्षण किया गया जिनमें कुछ लोगों ने कहा कि Z केवल टी वी चैनल देखते हैं दूसरे लोगों को केवल SUM टीवी चैनल ही पसंद है जबकि कुछ अन्य दर्शकों एशियनेट (Asianet) टी वी देखना पसंद करते थे ऐसे लोगों की प्रतिशतता बहुत कम थी जिन्होंने यह कहा कि वे तीनों चैनलों को देखते हैं दी गई आकृति में वत एशियानेट (Asianet) टी वी चैनल को वर्ग Z टी. वी. को तथा त्रिभुज SUM टी वी चैनल को दर्शाते हैं |
आकृति में दी गई किस संख्या से तीनों चैनलों को देखने वाले व्यक्तियों का पता चलता है |
View SolutionA survey shows that 63% of the people watch at news channel whereas 76% watch another channel. If x% of the people watch both channels , then :
View SolutionA survey shows that 63% of the people watch a news channel whereas 76% watch another channel. If x% of the people watch both channel then(a) x = 35(b)x=63(c)39≤x≤63(d)x = 39
View SolutionA survey shows that 63 % of the pepole watch a news channel whereas , 76 % watch an entertiament channel at a particular time If X% of the pepole watch both types of channels , then
View SolutionA survey shows that 63% of people watch a news channel while 76% of people watch another channel. If:% of people watch both channels, then-
View SolutionA survey shows that 63% of the people watch a news channel where as 76% watch another channel. If x% of the people watch both channel then,
View Solutionएक सर्वेक्षण से यह पाया कि 60% व्यक्ति एक न्यूज चैनल देखते है, जबकि 76% व्यक्ति दूसरा चैनल देखते है। यदि x % व्यक्ति दोनों चैनल देखते है तो
View SolutionIn a hostel there are.250 students. 120 watch news channels, 80 watch sports channels and 90 watch music channels. Each channel telecasts the content as per their nature. 50 students watch both news and sports channel 60 students watch both sports and music channel and 65 students watch both news and music channels. What is the minimum number of students who watch atleast one of the given channel?
View SolutionIn a hostel there are.250 students. 120 watch news channels, 80 watch sports channels and 90 watch music channels. Each channel telecasts the content as per their nature. 50 students watch both news and sports channel 60 students watch both sports and music channel and 65 students watch both news and music channels. What is the maximum number of students who watch atleast one of the given channels?
View Solution
