Axis of symmetry is obtained by x=−b/2a It implies that how far the axis of symmetry is from the U-axis.
Step by step video & image solution for Axis of symmetry is obtained by x= -b//2a It implies that how far the axis of symmetry is from the U-axis. by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Topper's Solved these Questions
Similar Questions
Write the axis of symmetry of the parabola y2=x.
View SolutionAlternate Axis OF symmetry
View Solution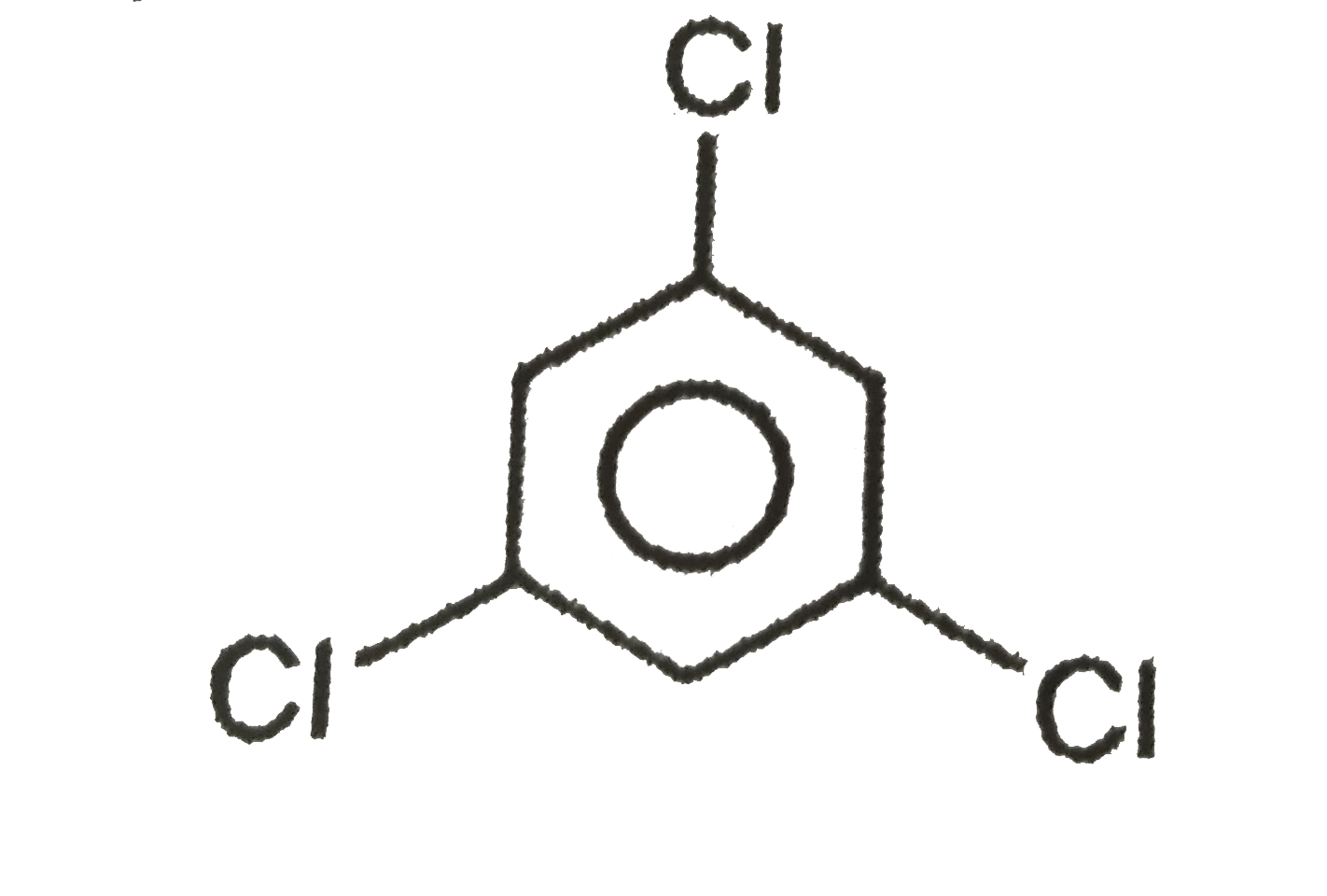
Sum of C_(2) & C_(3) axis of symmetry is View SolutionIdentify axis of symmetry in the given compound.
View SolutionForm the differential equation of the family of parabolas with focus at the origin and the axis of symmetry along the axis.
View SolutionCase Study Based- 3
Applications of Parabolas-Highway Overpasses/Underpasses A highway underpass is parabolic in shape.
Parabola
A parabola is the graph that results from p(x)=ax2+bx+c
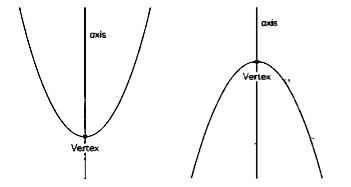
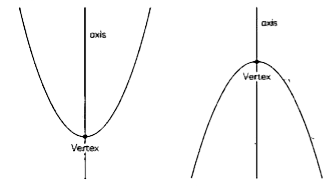
Parabolas are symmetric about a vertical line known as the Axis of Symmetry. The Axis of Symmetry runs through the maximum or minimum point of the parabola which is called the Vertex.
The number of zeroes that polynomial f(x)=(x−2)2+4 can have is:View SolutionCase Study Based- 3
Applications of Parabolas-Highway Overpasses/Underpasses A highway underpass is parabolic in shape.
Parabola
A parabola is the graph that results from p(x)=ax2+bx+c
Parabolas are symmetric about a vertical line known as the Axis of Symmetry. The Axis of Symmetry runs through the maximum or minimum point of the parabola which is called the Vertex.
If the highway overpass is represented by x2−2x−8. Then its zeroes areView SolutionFind the differential equation that represents the family of all parabolas having their axis of symmetry with the x-axis.
View SolutionLine of symmetry - if a line divides a given figure into two identical halves then we say that the given figure is symmetrical about that line and the line is called the axis of symmetry or line of symmetry.
View SolutionDefine axis of symmetry with the help of suitable figure.
View Solution