Calculate the standard deviation from the following following set of observations:
8, 9, 15, 23, 5, 11, 19, 8, 10, 12
The correct Answer is:5.23
To calculate the standard deviation from the given set of observations (8, 9, 15, 23, 5, 11, 19, 8, 10, 12), we will follow these steps:
Step 1: Calculate the Mean (x̄)
The mean (x̄) is calculated using the formula:
¯x=∑xn
where ∑x is the sum of all observations and n is the number of observations.
Calculating the sum:
∑x=8+9+15+23+5+11+19+8+10+12=120
Calculating the number of observations:
n=10
Calculating the mean:
¯x=12010=12
Step 2: Calculate the Deviations from the Mean
Next, we calculate the deviations of each observation from the mean and then square these deviations.
8−12=−4⇒(−4)2=169−12=−3⇒(−3)2=915−12=3⇒(3)2=923−12=11⇒(11)2=1215−12=−7⇒(−7)2=4911−12=−1⇒(−1)2=119−12=7⇒(7)2=498−12=−4⇒(−4)2=1610−12=−2⇒(−2)2=412−12=0⇒(0)2=0
Step 3: Sum of Squared Deviations
Now, we sum all the squared deviations:
∑(xi−¯x)2=16+9+9+121+49+1+49+16+4+0=274
Step 4: Calculate the Variance (σ²)
The variance is calculated using the formula:
σ2=∑(xi−¯x)2n
Substituting the values we have:
σ2=27410=27.4
Step 5: Calculate the Standard Deviation (σ)
Finally, the standard deviation is the square root of the variance:
σ=√27.4≈5.23
Final Answer:
The standard deviation of the given set of observations is approximately 5.23.
---
The mean (x̄) is calculated using the formula:
¯x=∑xn
where ∑x is the sum of all observations and n is the number of observations.
∑x=8+9+15+23+5+11+19+8+10+12=120
n=10
¯x=12010=12
Next, we calculate the deviations of each observation from the mean and then square these deviations.
Now, we sum all the squared deviations:
∑(xi−¯x)2=16+9+9+121+49+1+49+16+4+0=274
The variance is calculated using the formula:
σ2=∑(xi−¯x)2n
Substituting the values we have:
σ2=27410=27.4
Finally, the standard deviation is the square root of the variance:
σ=√27.4≈5.23
The standard deviation of the given set of observations is approximately 5.23.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - MEASURES OF DISPERSIONEXERCISE - EXERCISE 21 (a)12videos
MEASURES OF DISPERSION
- BOOK - ICSECHAPTER - MEASURES OF DISPERSIONEXERCISE - EXERCISE 21 (b)19videos
MEASURES OF DISPERSION
- BOOK - ICSECHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - EXERCISE14videos
MEASURES OF CENTRAL TENDENCY
- BOOK - ICSECHAPTER - MOCK TEST PAPER-2021EXERCISE - SECTION - C10videos
MOCK TEST PAPER-2021
Similar Questions
Calculate the standard deviation of the following data:
 View Solution
View SolutionCalculate the standard deviation of the following data:
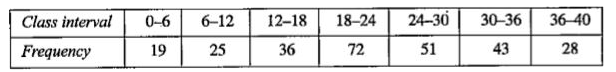 View Solution
View SolutionCalculate the standard deviation for the following distribution:
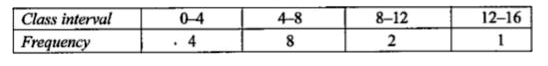 View Solution
View SolutionCalculate the standard deviation for the following distribution.
 View Solution
View SolutionCalculate the standard deviation of the following distribution:
 View Solution
View SolutionCalculate the standard deviation of the following distribuition :
 View Solution
View SolutionCalculate the standard deviation of the following given in the following table.
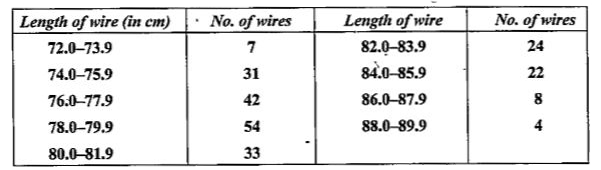 View Solution
View SolutionFind the standard deviation from the following observations:
15,18,13,20,17,10,16,19,22,20.View SolutionThe range of the following set of observations 2,3,5,9,8,7,6,5,7,4,3 is
View SolutionFind the standard deviation for the following data: (i) x: , 3, 8, 13, 18, 23 f: , 7, 10, 15, 10, 6
View Solution

