Draw a right angled isosceles triangle whose hypotenuse is 5.2 cm long.
To construct a right-angled isosceles triangle with a hypotenuse of 5.2 cm, follow these steps:
1. Draw the Hypotenuse:
- Start by drawing a straight line segment that is 5.2 cm long. Label the endpoints of this line segment as P and R. This line segment PR will be the hypotenuse of the triangle.
Hint: Use a ruler to measure and draw the line accurately.
2. Construct a Right Angle:
- At point P, you need to construct a right angle. To do this, use a compass to draw an arc from point P that intersects the line segment PR. Label the intersection point as O.
Hint: Ensure your compass is set to a reasonable width to create a clear arc.
3. Draw the Perpendicular Line:
- With the compass still at the same width, place the compass point on point O and draw an arc above and below the line segment PR. Label the intersection points of these arcs with a vertical line as L and T.
Hint: Make sure the arcs are of equal radius for accuracy.
4. Draw the 45-degree Angles:
- Now, you need to bisect the right angle at point P. To do this, place the compass on point L and draw an arc that intersects the line drawn from point P to T. Label the intersection point as Q.
Hint: The intersection point Q should lie on the line you drew from P through T.
5. Complete the Triangle:
- Connect points Q and R with a straight line. This line segment QR will form the second leg of the triangle. Now connect points Q and P to complete the triangle PQR.
Hint: Use a ruler to ensure that the lines are straight.
6. Verify the Angles:
- Since triangle PQR is isosceles and right-angled, angles P and R should both measure 45 degrees, and angle Q should measure 90 degrees.
Hint: You can use a protractor to check the angles if needed.
Final Notes:
- You should now have a right-angled isosceles triangle PQR with PR as the hypotenuse measuring 5.2 cm, and both legs PQ and QR should be equal in length.
- Start by drawing a straight line segment that is 5.2 cm long. Label the endpoints of this line segment as P and R. This line segment PR will be the hypotenuse of the triangle.
- At point P, you need to construct a right angle. To do this, use a compass to draw an arc from point P that intersects the line segment PR. Label the intersection point as O.
- With the compass still at the same width, place the compass point on point O and draw an arc above and below the line segment PR. Label the intersection points of these arcs with a vertical line as L and T.
- Now, you need to bisect the right angle at point P. To do this, place the compass on point L and draw an arc that intersects the line drawn from point P to T. Label the intersection point as Q.
- Connect points Q and R with a straight line. This line segment QR will form the second leg of the triangle. Now connect points Q and P to complete the triangle PQR.
- Since triangle PQR is isosceles and right-angled, angles P and R should both measure 45 degrees, and angle Q should measure 90 degrees.
- You should now have a right-angled isosceles triangle PQR with PR as the hypotenuse measuring 5.2 cm, and both legs PQ and QR should be equal in length.
Topper's Solved these Questions
Similar Questions
Knowledge Check

The figure above shows a right triangle whose hypotenuse is 4 feet long. How many feet long is the shorter leg of this triangle ?A2B8C2√3D2√33
In the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔABC is right angled isosceles triangle whose hypotenuse AC=4√2 units.
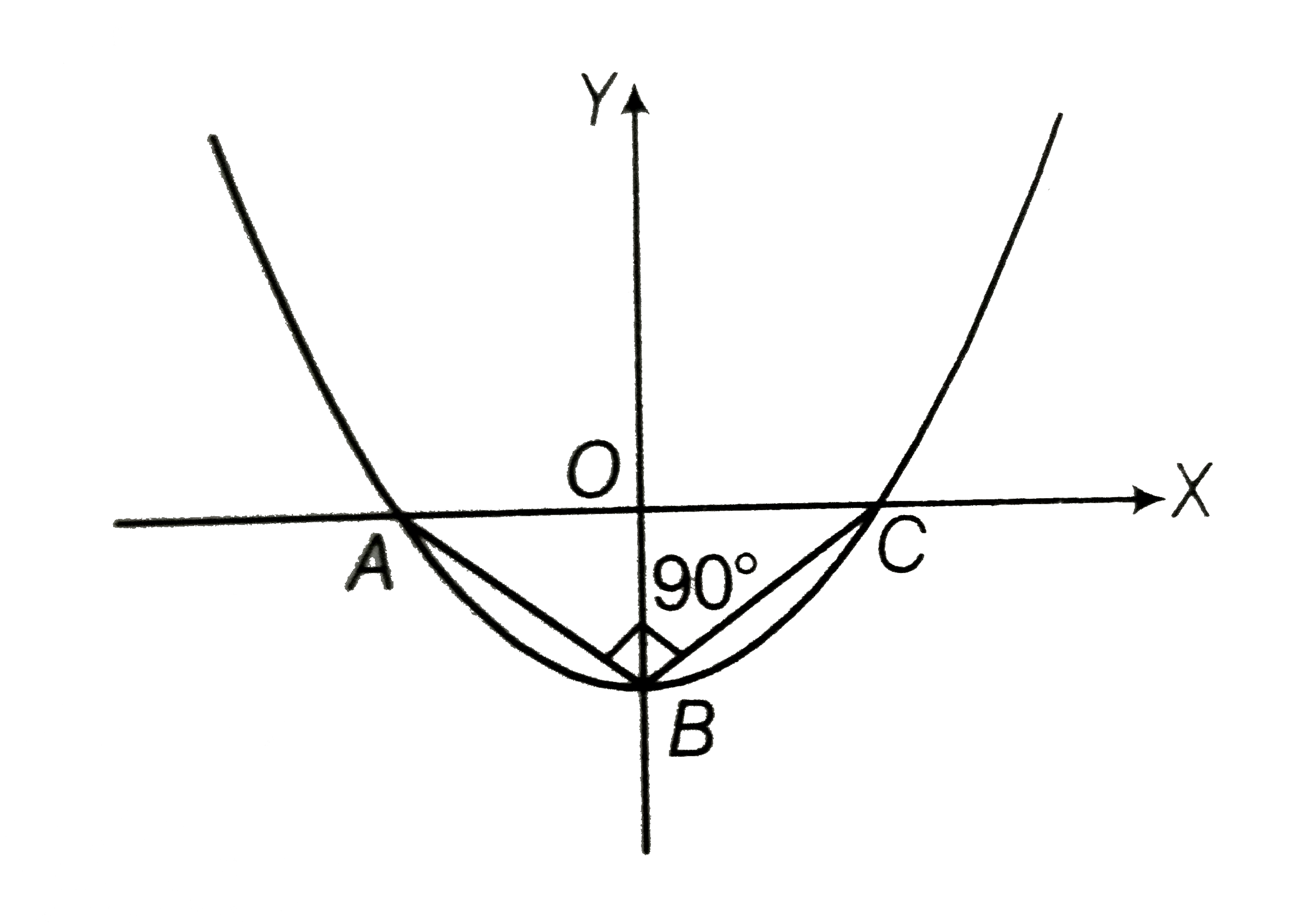
y=f(x) is given by
View SolutionShow that in a right angled triangle, the hypotenuse is the longest side.
View SolutionFind the area of a right triangle whose hypotenuse is 26 cm long and one of the sides containing the right angle measures 10 cm .
View SolutionConstruct a right-angled triangle with hypotenuse 8.2 cm long and one of the perpendicular sides of length 6 cm.
View SolutionDraw a right triangle whose hypotenuse is of length 4 cm and one side is of length 2.5 cm.
View SolutionIn a right angled triangle, the hypotenuse is four times as long as the perpendicular drawn to it from the opposite vertex. One of the acute angle is
View SolutionThe perimeter of a right angled triangle is 60 cm. Its hypotenuse is 25 cm. Find the area of the triangle.
View Solution
